Another way of thinking about the question is, "how close do you need to be for a dim star (our Sun) to outshine a brighter one?"
Consider our Sun, and another star a distance $d$ away that is $m$ times brighter than the Sun (in terms of luminosity or absolute magnitude). Let's look a location in space that is a distance $x$ from the Sun in the direction of the second star, and a distance $y$ in the perpendicular direction.
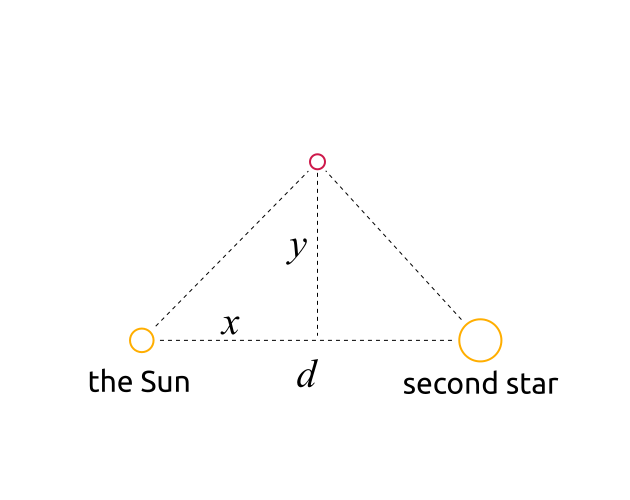
The amount of light seen at the location is proportional to the inverse square of the distance. The relative amount of light from each star is therefore just:
$$ \frac{1}{x^2+y^2} \qquad\qquad \frac{m}{(d-x)^2+y^2}. $$
We want to know when the first is greater than the second:
$$ \frac{1}{x^2+y^2} > \frac{m}{(d-x)^2+y^2} \\ (d-x)^2+y^2 > m x^2 + m y^2 \\ d^2 - 2dx > (m-1)x^2 + (m-1)y^2 \\ \left(1+\frac{1}{m-1}\right)d^2 > \left(\frac{1}{m-1}\right)d^2 + 2dx + (m-1)\left(x^2 + y^2\right) \\ \left(\frac{m-1+1}{m-1}\right)d^2 > (m-1)\left(\frac{d^2}{(m-1)^2} + 2x\frac{d}{m-1} + x^2 + y^2\right) \\ m\left(\frac{d}{m-1}\right)^2 > \left(\frac{d}{m-1} + x\right)^2 + y^2 \\ $$
This inequality describes a circlesphere of radius $r=d \sqrt{m}/(m-1)$, centered around the point $x=-d/(m-1),\ y=0$.
Let's take the specific case of Sirius, for which $m=25.4$ and $d=8.60~\text{ly}$.
Applying the above equations, we get $r=1.77~\text{ly}$ and $x=-0.35~\text{ly}$. Therefore, even at half the distance of the next-closest star Sirius is brighter than the Sun. This means that the Sun is the brightest star in the sky for exactly eight planets.