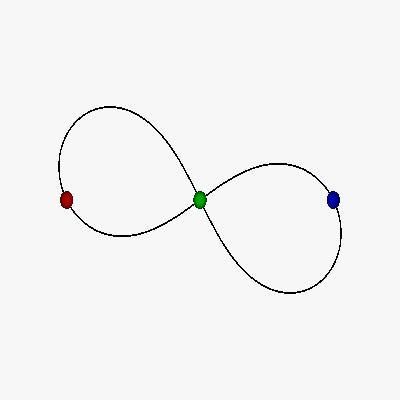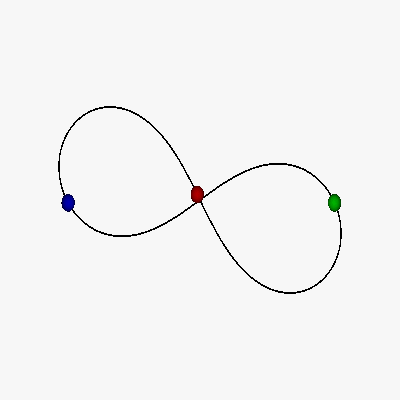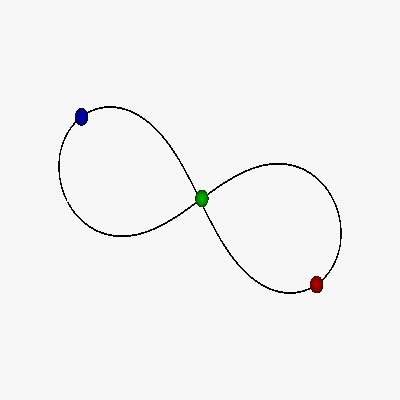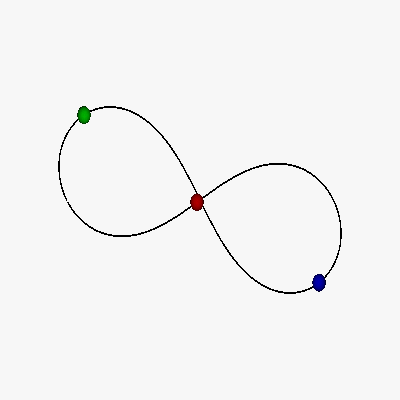
There may be some theethree-body periodic solutions that orbit around a common point, but in general they get a little crazy-looking and may not always contain an immediately obvious center of mass from casual observation. But of course it will always exist.
If you watch closely, you'll see that whenever one body reaches the intersection point, all three are on a straight line with the other two equidistant (symmetric). If you look where this orbit was originally described mathematically, they probably defined the initial conditions with the green one in the middle - so that the y coordinates of all there were zero and the line at that point is horizontal.
At those moments (all six permutations are present) the center of mass is at the intersection point since the masses are equal.
Since there are no external forces, the center of mass doesn't change in between those moments, so the center of mass is always at the intersection point.