This is a supplementary answer to an interesting question.
It seems to me that that the allowed margin of error for parabolic mirrors drops with an increasing focal length. It's already like 25 nm for advanced Earth-based telescopes - which is just 50-250 atoms.
I don't think that mirror figure error drops with focal length. Primarily it's the ratio of figure error to wavelength. Hubble's shortest wavelength is about 110 nm, well into the UV. 25 nm / 110 nm is... wait for it... $\approx \lambda/4$, the canonical figure error we often look for in telescope mirrors.
So a better question will be
Does the size of the atom limit the short wavelength limit of telescopes?
And the answer to that is probably not, and the reason is interesting! Using
$$E = \frac{hc}{\lambda}$$
and $hc = 1.24$ eV microns, we see that if an atom is 2.5 Angstroms and that's a quarter wavelength, the wavelength is 10 Angstrom or 0.001 micron. We're now talking about a photon energy of 1240 eV or 1.24 keV.
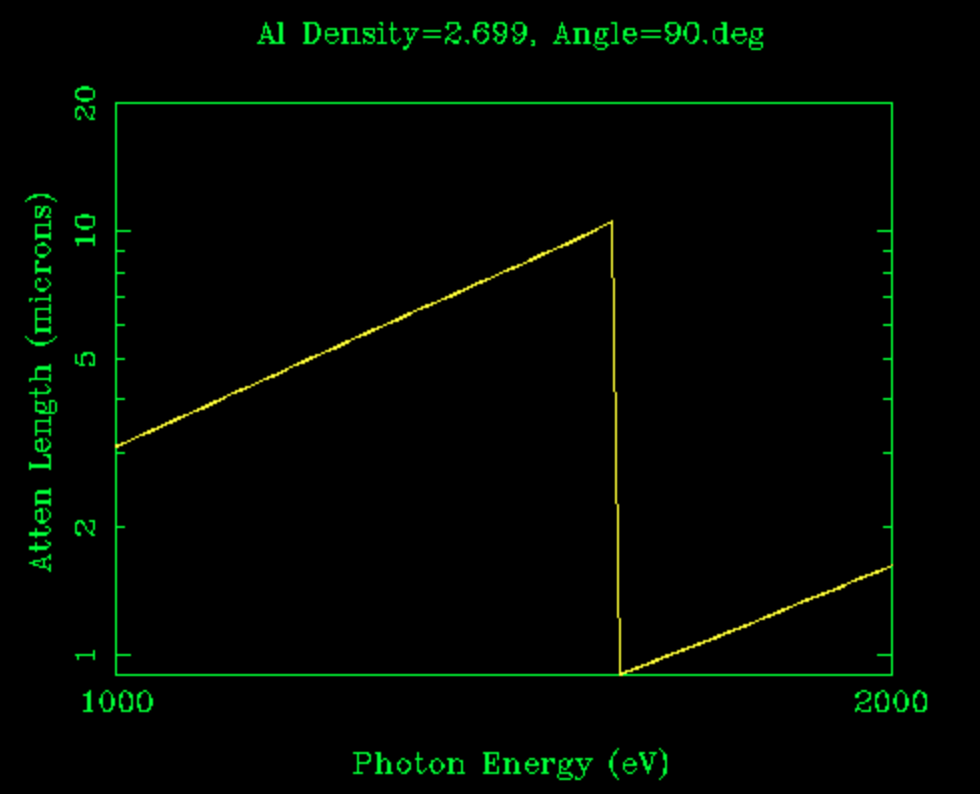
At that energy single atoms are mostly transparent. Here's a plot from https://henke.lbl.gov/optical_constants/atten2.html for the X-ray attenuation length in aluminum. It's of order of microns!
In other words, by the time that atomsindividual atoms become comparable to wavelength, they also become mostly transparent and it's really the bulk properties like crystal diffraction that become importantand/or tiny variations in index of refraction.
So atom size will never really matterinstead what's used for imaging X-rays in space telescopes (X-rays don't make it to the ground) is grazing incidence off of very specially manufactured surfaces. They are alternating layers of two materials with different index of refraction for X-rays. The difference is tiny and so the number of layers is large. The x-rays pass through many layers and sample the periodicity and depth of these material property variations. Single atoms out of place or atomic roughness of the layers might have a weak, second order effect, producing a bit of haze around very bright point sources, but they won't affect wavefront error.