Skip to main content
Astronomy

Return to Answer

added 294 characters in body
Source Link
HDE 226868 ♦
HDE 226868 ♦
  • 37.4k
  • 3
  • 130
  • 205

CouldIf you remove all milliparsec-separationwere able to construct a population of binaries, change parts at separations on the order of a parsec and crank up the GWB amplitude by manipulating your SMBHB population modelsparameters just right, and reproduce the spectrum? you'd might end up with a GWB with an amplitude at a few nanohertz comparable with what NANOGrav found. Loss-cone scattering, however, would mean you'd still be stuck with a power spectral density scaling as $\Phi(f)\propto h_c(f)^2/f^3\propto f^2/f^3=f^{-1}$. A glance at the posterior for $\gamma$ indicates we can firmly rule that out. Maybe, at

The bottom line: Even with the lowest frequency binsslight mis-match between $\gamma=13/3$ and what the NANOGrav results show, if you assumed models of disk interactions that allow you to getcan still reproduce the right scaling? But that would likely require you to get to weird parts of parameter spacedata reasonably well with adequate population models, and it's way, way more likely that weyou can't do it with just need to tweak the parameters of a populationbunch of milliparsecbinaries at parsec-separation SMBHBs and consider some simpler environmental effectsscale separations. Even "new physics" models (or invoke some slightly more exotic sources -- see Afzal et al. 2023 for "new physics" explanations of the results), which actually reproduce the spectrum quite well, are way more compelling.

Could you remove all milliparsec-separation binaries, change parts of your SMBHB population models, and reproduce the spectrum? . . . Maybe, at the lowest frequency bins, if you assumed models of disk interactions that allow you to get the right scaling? But that would likely require you to get to weird parts of parameter space, and it's way, way more likely that we just need to tweak the parameters of a population of milliparsec-separation SMBHBs and consider some simpler environmental effects (or invoke some slightly more exotic sources -- see Afzal et al. 2023 for "new physics" explanations of the results).

If you were able to construct a population of binaries at separations on the order of a parsec and crank up the GWB amplitude by manipulating your population parameters just right, you'd might end up with a GWB with an amplitude at a few nanohertz comparable with what NANOGrav found. Loss-cone scattering, however, would mean you'd still be stuck with a power spectral density scaling as $\Phi(f)\propto h_c(f)^2/f^3\propto f^2/f^3=f^{-1}$. A glance at the posterior for $\gamma$ indicates we can firmly rule that out.

The bottom line: Even with the slight mis-match between $\gamma=13/3$ and what the NANOGrav results show, you can still reproduce the data reasonably well with adequate population models, and you can't do it with just a bunch of binaries at parsec-scale separations. Even "new physics" models (Afzal et al. 2023), which actually reproduce the spectrum quite well, are way more compelling.

added 25 characters in body
Source Link
HDE 226868 ♦
HDE 226868 ♦
  • 37.4k
  • 3
  • 130
  • 205

The question, then, is how the power spectral density actually scales in the NANOGrav data. The answer: not quite as $\Phi(f)\propto f^{-13/3}$, but it's not obscenely far away. Here are the two big plots showing the results of a power law analysis (Fig. 1a and 1b, Agazie et al. 2023b). Note that these aremuch of the plot is at frequencies of tens ofabove a few nanohertz, and therefore where GW emission should drive the inspiral:

The question, then, is how the power spectral density actually scales in the NANOGrav data. The answer: not quite as $\Phi(f)\propto f^{-13/3}$, but it's not obscenely far away. Here are the two big plots showing the results of a power law analysis (Fig. 1a and 1b, Agazie et al. 2023b). Note that these are at frequencies of tens of nanohertz, where GW emission should drive the inspiral:

The question, then, is how the power spectral density actually scales in the NANOGrav data. The answer: not quite as $\Phi(f)\propto f^{-13/3}$, but it's not obscenely far away. Here are the two big plots showing the results of a power law analysis (Fig. 1a and 1b, Agazie et al. 2023b). Note that much of the plot is at frequencies above a few nanohertz, and therefore where GW emission should drive the inspiral:

Source Link
HDE 226868 ♦
HDE 226868 ♦
  • 37.4k
  • 3
  • 130
  • 205

TL;DR: We do have gravitational wave emission from supermassive black hole binaries (SMBHBs) at larger separations, but a background created from solely those binaries won't have the characteristic shape or cover the frequency ranges we both expect and observe it to have.

As a preface, there are numerous theoretical solutions to the final parsec problem, as mentioned by Agazie et al. 2023a, the astrophysical interpretations paper (see this nice answer on Physics Stack Exchange for a quick overview of the state of the problem). We don't know what the right explanation is, but both existing observational evidence and more sophisticated theoretical modeling show that, well, nature has found a way to solve it, and the problem might not be a problem at all. The new pulsar timing array results support that point even more.

First off, there are several different separation regimes, where different environmental processes dominate the evolution of the binary through hardening, decreasing its semimajor access (Taylor 2021). The different separations also mean we expect them to be important at different frequency ranges.

  1. Kiloparsecs to parsecs: Dynamical friction, the "drag" acting on a binary from an ensemble of stellar scattering events in the aftermath of a galaxy merger. This contributes no significant shape to the gravitational wave spectrum.
  2. Parsecs: Stellar loss-cone scattering of individual stars near the core. This contributes a strain scaling with frequency as $h_c(f)\propto f$.
  3. Centiparsecs to milliparsecs: Viscous interaction with a circumbinary disk. This can produce a variety of strain contributions from $h_c(f)\propto f^{-1/6}$ to $h_c(f)\propto f^{1/2}$.
  4. Milliparsecs and below: Gravitational wave emission. At this point, the inspiral is driven by nanohertz gravitational waves, producing a strain scaling as $h_c(f)\propto f^{-2/3}$. This is expected to be the (effectively) sole contribution to the gravitational wave background at the frequencies pulsar timing arrays are sensitive to. The power spectral density (PSD) then goes like $\Phi(f)\propto h_c(f)^2/f^3\propto f^{-13/3}$. You might have heard "13/3" mentioned as a key characteristic of the (idealized!) GWB; this is where that scaling comes from.

Here's a nice plot showing some of these contributions, Fig. 4 of Burke-Spolaor et al. 2019:

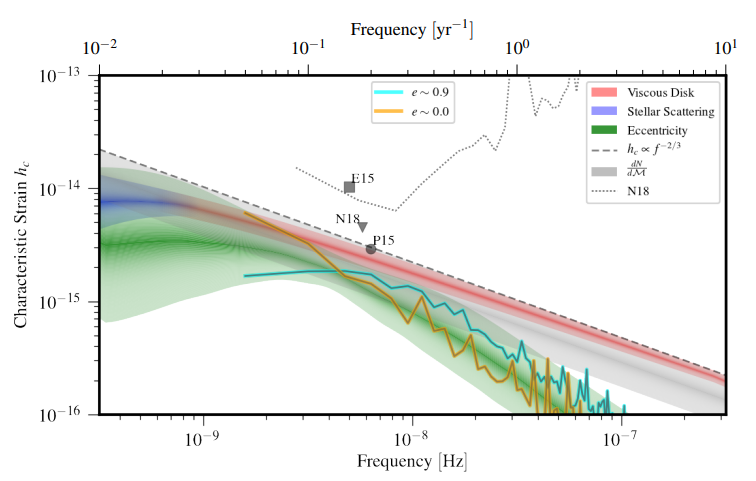
Plot showing the shape of the GW spectrum from the separations dominated by the different environmental processes across the nanohertz regime of the gravitational wave spectrum.

In short, we expect to see some contributions at lower frequencies from loss-cone scattering and disk interaction before gravitational wave emission takes over completely at frequencies around a few nanohertz. The different environmental mechanisms act at different frequency ranges and provide different shapes.

The question, then, is how the power spectral density actually scales in the NANOGrav data. The answer: not quite as $\Phi(f)\propto f^{-13/3}$, but it's not obscenely far away. Here are the two big plots showing the results of a power law analysis (Fig. 1a and 1b, Agazie et al. 2023b). Note that these are at frequencies of tens of nanohertz, where GW emission should drive the inspiral:

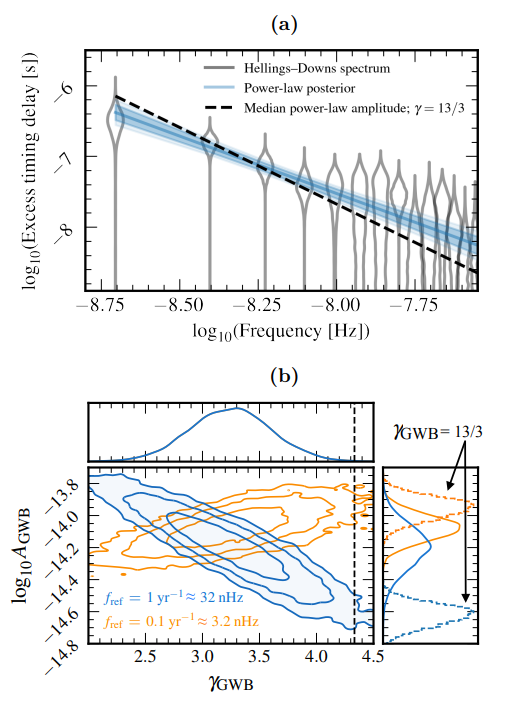
Plots of both a power-law fit to the data and posteriors for the amplitude and power-law index of the GWB.

The top plot shows both posteriors for the free spectrum fit -- i.e. allow $\Phi(f)$ to scale as $f^{-\gamma}$ for any $\gamma$ -- in blue, and a fit to a power law forcing $\gamma=13/3$, in black. The bottom plot shows joint and individual posteriors for the spectral index and the GWB amplitude. You can see that a shallower power law is favored over our idealized GWB. $\gamma=13/3$ is within the $3\sigma$ confidence region, but there's still a noticeable difference. Still, the posterior is definitely not consistent with the mechanisms driving evolution on the scale of parsecs.

So, yes, the strain doesn't scale the way we'd expect for our GWB where gravitational wave emission is the sole driver of the inspiral. However, $\gamma=13/3$ just represents the idealized model of gravitational wave emission at those milliparsec scales, and it's entirely possible -- likely, really -- that there are other environmental factors contributing to the inspiral and yielding a shallower power law. You can also tweak the population parameters and get something consistent with the spectral index and amplitude we see (and Agazie et al. 2023a talks about these a lot).

Could you remove all milliparsec-separation binaries, change parts of your SMBHB population models, and reproduce the spectrum? . . . Maybe, at the lowest frequency bins, if you assumed models of disk interactions that allow you to get the right scaling? But that would likely require you to get to weird parts of parameter space, and it's way, way more likely that we just need to tweak the parameters of a population of milliparsec-separation SMBHBs and consider some simpler environmental effects (or invoke some slightly more exotic sources -- see Afzal et al. 2023 for "new physics" explanations of the results).