How long until the Earth and Moon become a binary planet?

Question

With the rate at which the moon is further receding into a higher orbit, how long until the barycentre between us and the moon leaves the earth, and going by the IAUs 2006 definition update, we become a double planet?

Answer 1

I'm not sure I agree with the double planet POV, but the calculation is pretty simple. The earth weighs 81 moons, so for the Barycenter to be outside the earth, the distance (center of Moon to surface of earth), = 81 earth radii.

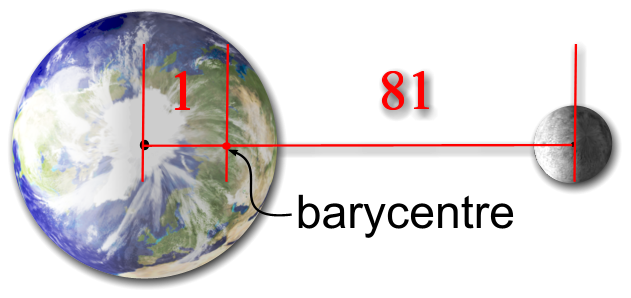
or about 515,000 KM. It's current farthest distance is 405,000 KM, average distance 384,000 KM and closest 363,000 KM Source, so, it depends on whether you mean, temporarily outside, outside more than 50% of the time or always outside - each would provide different answers.

But moving away 4 CM per year (same source) or 1 KM every 25,000 years, it would need 2.75 billion years to move away the necessary 110,000 KM necessary to have the barycenter move outside of earth at the moon's furthest point. (less if the orbit gains eccentricity which is possible). But probably more than that, cause as the moon moves away from the earth, the tidal forces that continue to push it away grow weaker and the earth slows down a little. If the earth ever gets tidally locked with the moon, the reverse will happen and the sun's tidal forces will draw the Earth and Moon slowly towards each other, so a precise answer is too mathematically difficult for me, but longer than 2.75 billion years seems a pretty good guestimate.