Why is the metallicity of elliptical galaxies low?

Question

As far as I understand, metallicities of old stars are usually low and those of new stars are usually high.

If elliptical galaxies form after merger of galaxies, then surely that means the elliptical galaxy passed through a stage where it was an irregular galaxy. Now, irregular galaxies are said to be sites of rapid, new star formation.

Why then do we say that elliptical galaxies have low metallicity, if the star formation went on for a long time there?

[New to astronomy, so a detailed explanation would help]

Answer 1

It isn't true that elliptical (or early-type) galaxies are metal-poor. This sounds like a pop-sci myth being propagated. The first line of the abstract of Pipino & Matteucci (2006) states that "Elliptical galaxies probably host the most metal rich stellar populations in the Universe". Thus your suspicion of the idea that "ellipticals are metal-poor" is well-founded.

Measurements of galaxy metallicities and metallicity gradients have been around for decades and show that ellipticals obey fairly simple scaling relationships between mass, luminosity, velocity dispersion and metallicity.

In terms of metallicity, massive ellipticals with bigger velocity dispersions are a bit more metal-rich than the Sun, whilst smaller galaxies may get down half the solar metallicity. The range is much larger for spiral galaxies. In addition, there is a tendency for ellipticals that are older to have higher metallicities. Examples of these trends can be seen in Graves & Faber (2010) and Li et al. (2018).

The central plot here (taken from Graves & Faber 2010) shows how the metallicity of galaxies varies with their stellar velocity dispersion (an indicator of their mass). [Z/H] is a logarithmic base 10 scale where 0.0 means the metallicity of the Sun and -1 would a tenth the metallicity of the Sun. You can see that the elliptical galaxies (red) have a relatively narrow range of metallicities, that most are as metal-rich as the Sun, that less massive ellipticals have lower metallicity and that spirals (blue) have a much bigger range of metallicity.

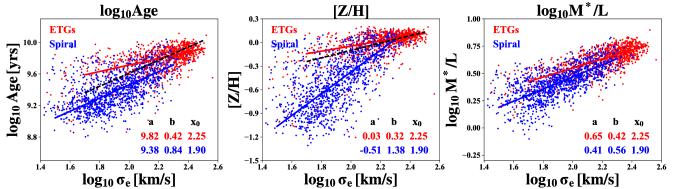
The reasons behind these trends are still debated. But as you rightly point out, ellipticals likely form from mergers of objects that have already been actively forming stars. A low metallicity is therefore not necessarily expected. Much depends on how much enriched gas from the ejecta of previous (higher mass) stellar populations is retained within the galaxy. Higher mass galaxies with deeper gravitational potentials are able to retain more of that enriched gas.

Answer 2

Elliptical galaxies have no regions of high density, so they are not forming stars any more. So all the stars in an elliptical galaxy are old stars, and as you say, tend to have low metallicity (actually, as pointed out, that doesn't seem to be true-- they have higher metallicity, presumably from old star formation no longer occurring). You are right that they might have had active star formation in the distant past, but it ended long ago. Spiral galaxies like the Milky Way have been forming new stars for their entire history, so have more opportunity for current metallicity increase, but seem to be starting out behind, as pointed out above.