Why doesn't the equipartition theorem disallow spinning dust?

Question

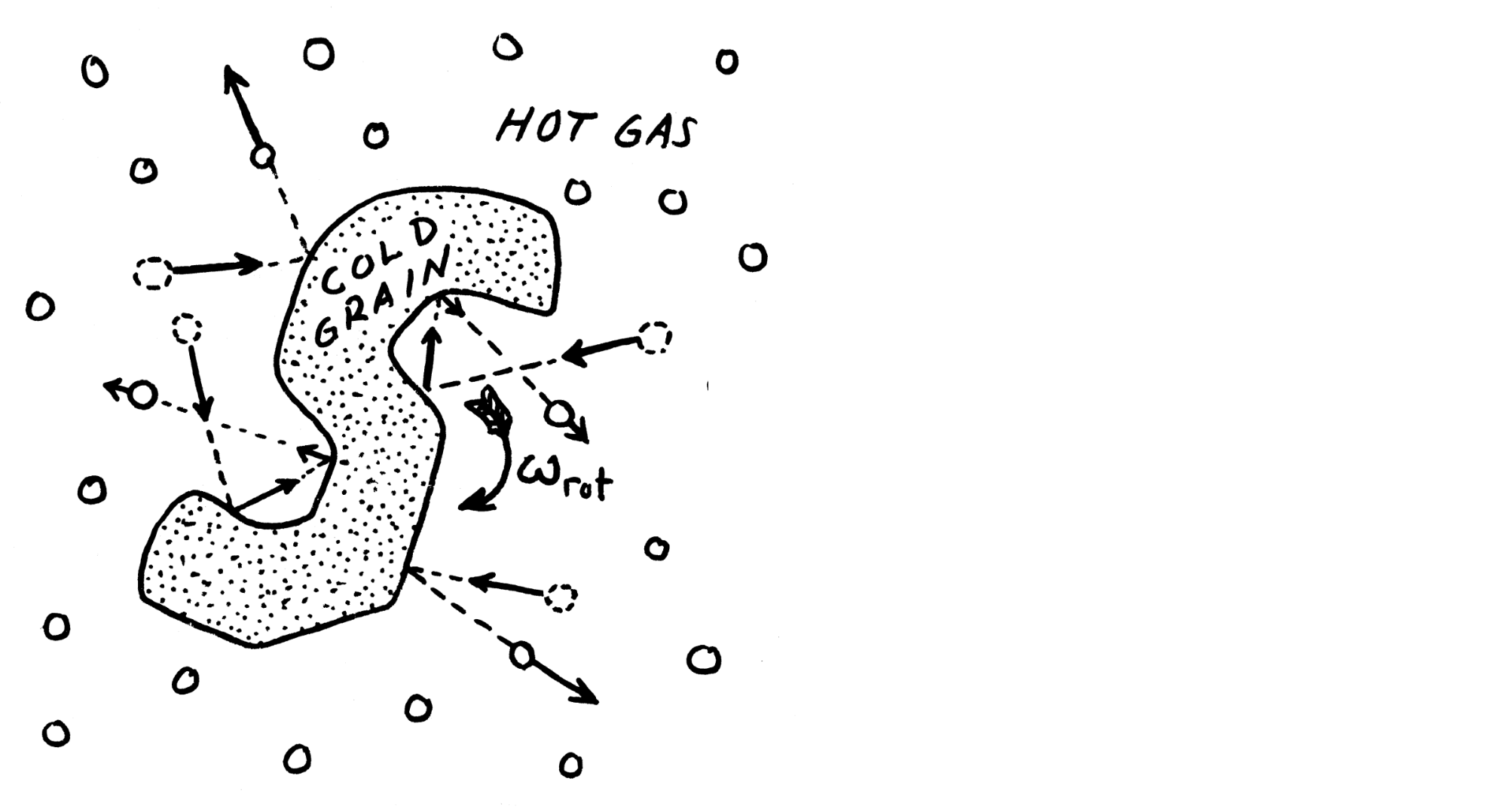
This excellent answer to the question What is the physics of the “spinning dust” contribution to Cosmic Microwave Background measurements? Is well sourced. The abstract of one of the papers there, Purcell 1975 Interstellar grains as pinwheels begins with:

Contrary to the assumption usually made in theories of grain alignment, the rotational energy of an interstellar grain is likely to be very much greater than 3/s kT, where T is the gas temperature, or the grain temperature, or any other temperature in the system. Any more or less permanent irregularity of the grain’s surface with respect to accommodation coefficient, distribution of H-H recombination sites, or photoelectric emissivity will result in an unbalanced torque capable of spinning the grain up to high angular velocity. Such a grain is, in effect, the rotor of a heat engine.

In the body of the paper, the first example given is of a sort-of gedankenexperiment involving a hypothetical Crookes radiometer with the gas removed. The author explains that it would spin up to high energy (without saying how):

[...] The kinetic energy of the rotating paddle wheel is now enormously greater than it would be if it were immersed in, and en equilibrium with, a 6000 K radiation field, for then it would exhibit only a residual “Brownian rotation” appropriate to that temperature. So the wheel is really a heat engine, depending on the difference between the temperature of the vanes and that of the radiation field to which they are exposed.

Without the gas to cool them, I suppose that after the whole thing reaches equilibrium temperature the black sides radiate more strongly than the white sides and so there should always be a torque, and the final speed and kinetic energy would be limited only by friction and other practical losses.

This excellent answer to the question What is the difference between gas and dust in astronomy? points out that there is no real distinction between the two, but that they are limits in size, gas particles being small and dust particles being large(r).

So if dust can become pinwheels, then I have a hunch that very very large molecules can do this too, and if so, then so can smaller molecules. All you need is some mechanism for torque. Molecules can certainly have a non-uniform distribution of H-H recombination probability just as dust can, and they can have some regions that are concave and some that aren't.

This makes me wonder if these gas molecules no longer obey the Equipartition theorem of thermodynamics. The spinning dust certainly doesn't.

Question: How can spinning dust and by induction, my hypothetical spinning molecules avoid conforming to the equipartition theorem? Why doesn't the equipartition theorem disallow spinning dust?

Source borrowed from here

Bonus points: Is that little arrow ($\omega_{rot}$) pointing the wrong way?

Answer 1

The first relevant point is that the equipartition theorem only formally applies in thermodynamic equilibrium, which requires that all temperatures be the same. Since the spinning dust only happens when there are temperature differences, holding to the analogy with a heat engine mentioned in the source, we should not expect that theorem to be the whole story. Granted, we often apply that theorem even when there are temperature differences, so the issue boils down to, when is the behavior more or less the same when all temperatures are the same, and when is the behavior fundamentally a heat engine? I will assume the source is correct that this is heat engine-like behavior for spinning grains.

Once you have a heat engine in operation, the sky is the limit on how much energy you can put in any given mode (as long as you can maintain the temperature difference), because a heat engine does work, and work energy never needs to be equipartitioned, you can partition it any way you like by setting up an appropriate apparatus. The dust particle is therefore a kind of apparatus for doing work, in the presence of temperature differences, and channeling the work energy into rotation.

How and why that heat engine operates and picks rotation to channel the work is a much more subtle issue. For example, do we need temperature differences across the dust grain itself, or just between the grain and the surroundings? If the "hypothetical" Crooke's radiometer (meaning, one that works quite differently from actual Crooke's radiometers and actually spins the opposite direction) is a good analogy, then the spin is from temperature differences across the dust grain itself, which warm the gas in the surroundings of the dust grain, producing gas flows that maintain constant pressure. Those flows require the gas to receive torques, and conservation of angular momentum requires an opposite torque on the dust grain.

To get torque like that, it seems that you not only need a temperature difference across the dust grain, but it also has to have a kind of "quadrupole" character-- hot-cold on one side of the grain, cold-hot on the other, producing a "handedness" to the temperature structure in the grain. So we immediately see that one cannot get spin until one has a large enough system to be able to support the concept of "temperature difference" across the object, and molecules are generally not thought of that way-- though maybe in the case of very large molecules, they could be. So the question then comes down to, how large does a molecule need to get before it can support a concept of spatially nonuniform temperature? I don't know how big that needs to be, but my guess is, very big indeed-- there are certainly polymers that can get very large, but the molecules talked about in astronomy are generally not polymers.

As for the sign of the rotation, that can be very difficult to figure out! It seems to depend on how the temperature differences get created in response to absorption of a radiation field (or other interactions with the environment, perhaps thermal contact with warmer gas). Generally speaking, concave sides warm up more quickly than convex, so cause the gas in the concave part to be warmer than in the convex, so a flow sets up from concave to convex. That acts like a jet engine that pushes the concave side forward. That this is subtle can be seen from the fact that the higher pressure gas would seem to push the other way on the dust, but it's like the way a sail on a sailboat works-- follow the deflection of the air and conclude that the boat deflects the opposite way.

If this is all correct (see the Wiki on Crooke's radiometers for background information), then I would say the shape of the dust grain you drew produces spin in the direction that you indicated, but that no astrophysical molecules are large enough to produce the same effect.