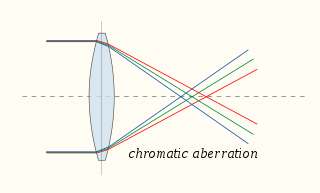
The actual math is a bit complicated, but there's a simple intuitive explanation.
Longitudinal chromatic aberration happens because, when you cut a convergent lens in two, and you look at the cross-section, the edge of the lens looks a bit like a prism, doesn't it? (look at the diagram that you've posted above, the top of the lens) And it does exactly what a prism does - it makes a "pretty" little rainbow. Not so pretty when you're trying to do astronomy, however.
Short, fat, triangular prisms make widespread rainbows. Thin, elongated, thin-wedge shaped prisms make tight, narrow rainbows. The spread of the colors increases as the two sides of the prism are separated by a wider angle. It decreases as the two sides get closer together at a narrow angle. At the extreme, if the two sides of a "prism" were parallel, there would be no rainbow - but then it's not a prism anymore, it's just a glass plate, completely flat.
All else being equal, a single-lens-objective refractor has a "fatter" lens when focal length gets shorter (the two sides of the lens are more strongly curved, and/or less parallel); it has a "thinner" lens when the focal length gets bigger (the two sides of the lens are less strongly curved, and/or closer to parallel). A "fat" lens at the edge is like a fat, blunt prism. A "thin" lens at the edge is like a sharp, slender prism. The former makes a big spread of color. The latter makes a much more tight rainbow.
So that's what the old telescope makers did, back in the day (Hevelius, late 1600s). They just pumped up the focal ratio, made these super-long refractors with weakly curved lenses to combat the rainbow edge images which are so annoying and ultimately can falsify your observations.
Now, if you study the properties of lenses, you notice that divergent lenses (the kind that make the image smaller) have a chromatic aberration opposite to convergent lenses (lenses that magnify, and that were used as primary, or objective lenses in telescopes back then). It could be argued that by combining a convergent and a divergent lens, chromatic aberration might be greatly reduced; as long as the convergent lens is stronger than the divergent companion, the combination should remain convergent.
Also, different glasses need to be used, so that the convergent lens is strongly convergent, but weakly dispersing, while the divergent lens is weakly divergent, but strongly dispersing. That's how you get near zero dispersion, but still pretty decent convergence.
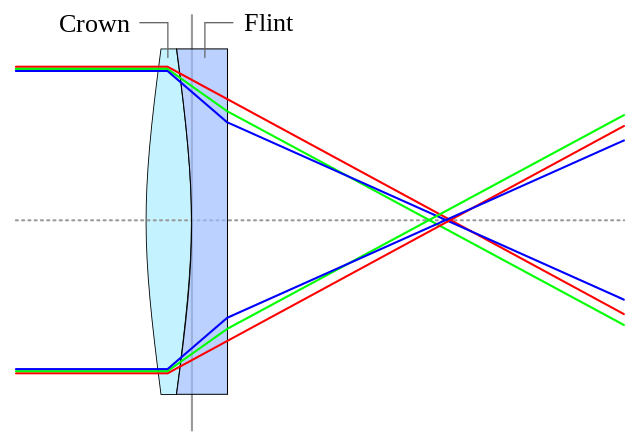
In the early 1700s this was accomplished by British opticians. Chester Moore Hall is credited with the making of the first achromatic objective lens using the scheme shown above. But the actual work of grinding/polishing the glass was contracted to George Bass, who noticed that the two lenses he was making, when put together, had near-zero chroma. Hall failed to recognize the practical applications of the achromats, neglected his own invention, and years later Bass mentioned the achromat scheme to John Dollond, who understood its value and started to popularize the new design.
https://en.wikipedia.org/wiki/History_of_the_telescope#Achromatic_refracting_telescopes
https://en.wikipedia.org/wiki/Achromatic_lens
The achromatic combo guarantees zero chromatic aberration at two wavelengths. So you basically correct the aberration in the red and blue, or something like that, and the rest is "good enough". Things can be improved further by adding another lens - the apochromatic objective, or the "apo", or the "triplet". This was invented by Peter Dollond, John's son, in the mid-1700s.
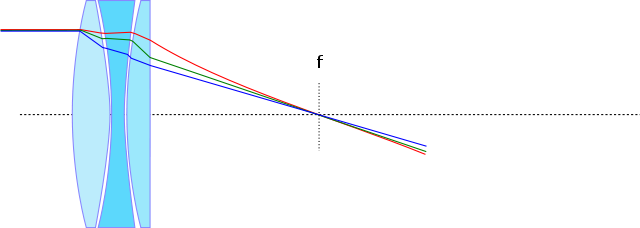
The apochromat guarantees zero chromatic aberration at three wavelengths - usually in the middle and then closer to the two extremes of the visual spectrum. So that's overall better than the previous scheme.
https://en.wikipedia.org/wiki/Apochromat
The best apo refractors have extremely low chromatic aberration, basically invisible to the eye in most situations. But they are expensive.
Further improvements can be made. The most expensive amateur refractors are "quad" schemes, whereby using 4 lenses zeroes out chroma at 4 wavelengths. Takahashi is a brand nowadays known for excellent quality quad refractors, used in top-level astrophotography rigs.
BTW, not just chroma, but basically all aberrations can be reduced by increasing the focal ratios of single lens objectives. Pretty much every single aberration gets better if you put a piece of paper in front of it, with a hole smaller than the diameter of the lens. But the aperture gets smaller, resolving power gets worse, there's less light captured by the instrument, etc. Tradeoffs...
W.r.t detailed math of single lens chromatic aberrations, see chapter 5.3.1. Chromatic Aberration of a Singlet in 'Telescopes, Eyepieces, Astrographs' by G.H. Smith, R. Ceragioli, R. Berry. I believe that chapter is exactly the answer to your question, complete with working out the math for the aberration as a function of lens parameters.
http://www.willbell.com/tm/TelescopesEyepiecesAstrographs.html
Given your interest in the theory of optical systems, you should read that book.
Also worth mentioning:
Introduction to Lens Design, by J.M. Geary
http://www.willbell.com/tm/lens_design/index.htm
Practical Computer-Aided Lens Design, by G.H. Smith:
http://www.willbell.com/tm/lensdesn.htm
Some good theoretical info, as always, can be found on telescope-optics.net
https://www.telescope-optics.net/chromatic.htm