Short answer.
Great question! Ask yourself what absorbs better, something that is white, or black.Then ask yourself what radiates better. The answer is the same thing. It turns out that absorptivity and emissivity are linked pretty closely.
Kirchov's Law of Thermal Radiation can be stated as:
For an arbitrary body emitting and absorbing thermal radiation in thermodynamic equilibrium, the emissivity is equal to the absorptivity.
If you know that the atmospheric absorption at a given wavelength is 0.01, then you could estimate the emissivity to be 0.01 as well. It doesn't mean it's exactly right, but it's a great start.
If you then say the average temperature of the atmosphere in the region where absorption (and thermal radiation) at 4080 MHz is 230K, then the Noise Equivalent Power (NEP) will be 0.01 time 230 K or 2.3 K.
How can we express power in terms of a temperature?
Wait a minute, thermal radiated power varies as something like temperature to the fourth power! How can we add two temperatures together, or multiply them by 0.01?
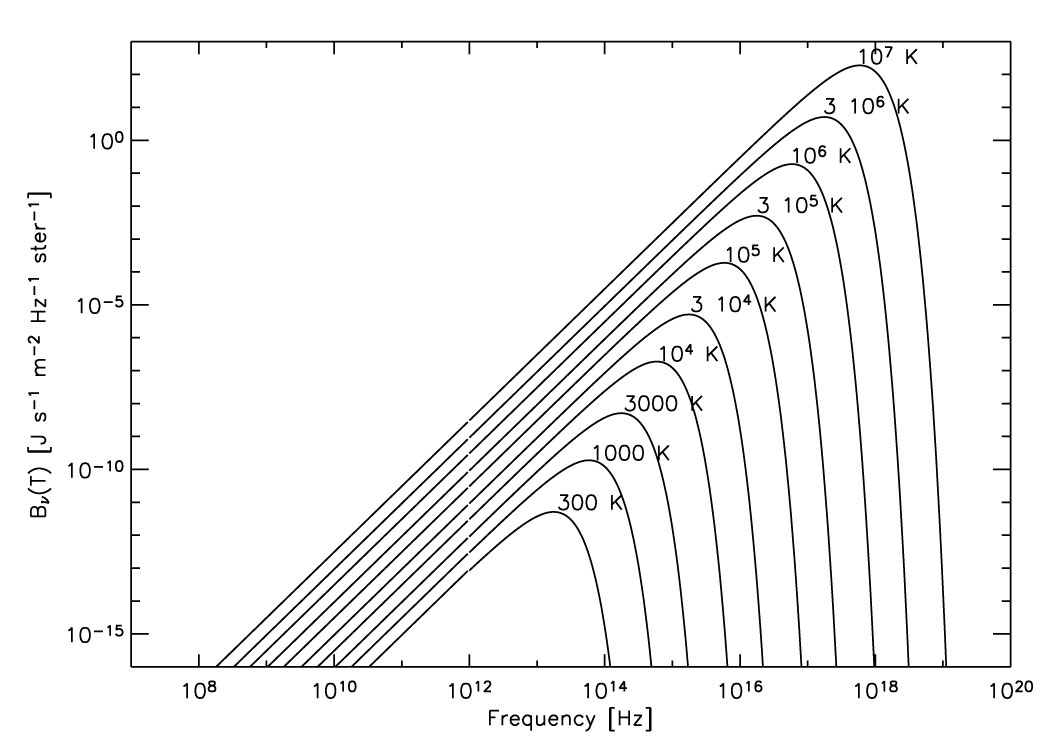
It's because the energy of the photons we're talking about is way down on the left edge of Planck's blackbody distribution. See for example this answer to the question Why doesn't thermal radio emission from a DSN “hot dish” completely swamp the benefits of a cold LNA? Down at photon energies well below $k_B T$ we can use the Rayleigh-Jeans law where the power at a given frequency depends linearly on temperature.
Plotted on a log-log graph, you can see that the peak heights increase three times faster than the straight-line low-frequency segments do, showing the $T^3$ dependence of the spectral density peak height versus the linear behavior in the Rayleigh-Jeans regime. If you integrated the total area under the curve, you'd get the $T^4$ dependence of the total power radiated by a blackbody.
below: From New Jersey Institute of Technology's Dr. Dale Gary's nicely-written Physics 728 Radio Astronomy; Lecture #1 notes: