Why is blue light extincted more strongly than red?

Question

We know that blue light suffers extinction more strongly than red (examples include ISM, atmosphere etc.), but why? Is it a property of the shorter wavelength itself? Can someone provide a conceptual explanation as to why this occurs? TIA

Answer 1

There are two effects causing this:

The relevant quantity for determining whether or not a photon interacts with a particle is the ratio $$ x \equiv \frac{2\pi r}{\lambda}, $$ where $r$ is a size of the scattering agent.

Rayleigh scattering

When $x\ll 1$, we're in the Rayleigh regime where the wavelength is so long compared to the particle that the electric field of the photon causes charges within the particle to oscillate with the same frequency, effectively resulting in dipole scattering. This has a very strong wavelength dependence, with the probability of interaction begin proportional to $\lambda^{-4}$.

Mie scattering

When $x \sim 1$, we're in the Mie regime, where the probability of interaction is largest. So if you had an ensemble of randomly sized particles with an equal amount of any size, and you shone a beam of light consisting of photons with the same distribution of wavelengths, you would scatter all photons with equal probability (unless of course your distribution extended to very small values of $x$).

However, as with many other things in the Universe — e.g. galaxies, stars, cities, lakes, and wealths — dust grains are not equally distributed in size, but has a distribution which declines as a power law from small to large size. In the case of dust grains, this power is very steep, with a power law slope of roughly $-3.5$; that is, the density $n$ of dust grains as a function of size drops as $$ n(r) \propto r^{-3.5}. $$

Hence, there are many more small dust grains available, casing the blue photons to be scattered much more often.

Larger particles / smaller wavelengths

When $x>1$, but less than $\simeq1.8$, the probability of scattering declines, meaning that in this narrow window, red light is scattered more easily than blue. Coincidentally, these values correspond to optical light and the dust on Mars, leading to blue sunsets, as discussed in this answer.

When $x\gg 1$ — i.e. when the particles become very large compared to the wavelength — we have "geometric scattering", all light is scattered equally. In astronomy, we call this "gray dust", and would be caused e.g. by smalles pebbles.

Scattering vs. absorption

The albedo $A$ of the dust grains — i.e. the probability that an interaction result in a scatter rather than an absorption — is also wavelength dependent, but not as strong. Typical values are of the order of $0.5$, but decreases toward zero for long wavelengths (e.g. Pei 1992)

Answer 2

Reddening (or the fact that blue light is more extincted than red, causing objects to appear more red) is due to the interaction between the light and the dust grains and gas molecules it is going through, and is caused by the relative size of the dust grains and of the wavelength. Indeed, dust grains are very effective at scattering light which has a wavelength that is about the same size as the grain.

See this illustration from the university of virginia

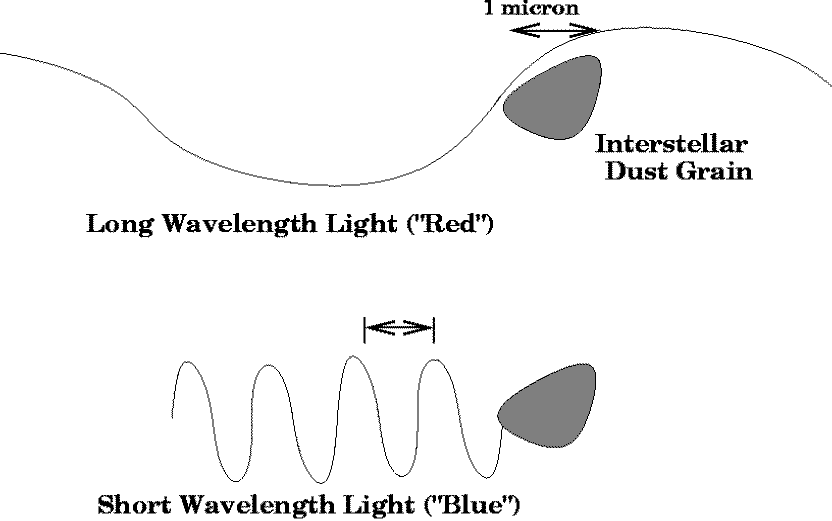
Dust grains in the interstellar medium are about 1 micron in size or less. Because of this, blue light (0.4 $\mu$m) gets absorbed/scattered by the dust, but red light (0.7 $\mu$m) goes through. Since infrared has an even longer wavelength, there are nebulae that obscure the background stars in visible light, but that are transparent in infrared.

The same phenomenon causes sunsets to appear red, and even more so after a volcanic eruption, when there is more dust in the atmosphere.