4
$\begingroup$

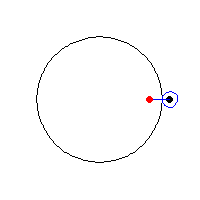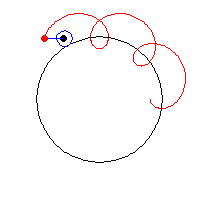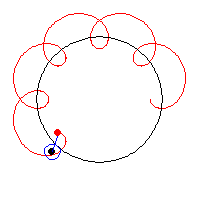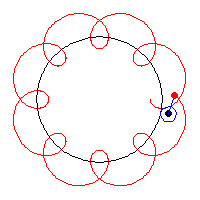
I naively believed that since our Moon orbits Earth, and since Earth orbits the Sun, the path our Moon might take around the Sun would be this type of epitrochoid curve:

enter image description here

I was surprised and delighted to find that our Moon's orbit around the Sun actually forms a convex curve which is really darn close to Earth's orbital path: Moon's orbit around the Sun. Since the Earth's instantaneous velocity around the Sun is much greater than the Moon's instantaneous velocity around the Earth, we don't get any of the closed "curlicues" I expected to see.

Are there any moons in our solar system with epitrochoid paths around the sun that appear to have closed curlicues? If so, what are they and what do the paths look like?

Improve this question
$\endgroup$
1

1 Answer 1

Reset to default
12
$\begingroup$

A moon will describe a path like this if its orbital speed relative to its parent planet is greater than the parent planet's orbital speed about the Sun. (Assuming the moon's orbit about the planet and the planet's orbit about the sun are roughly coplanar; I'll ignore Uranus for the remainder of this discussion.) Going through the planets:

  • Neptune: All moons from Proteus inwards (7 total) orbit Neptune sufficiently fast. However, they're all pretty small; in particular, all are irregularly shaped, since they're all too small to form into spheres under their own gravity.
  • Uranus: (ignored because the paths of the moons are just too baroque)
  • Saturn: All moons from Dione inwards (20 total), along with Dione's trojan moons Helene & Polydeuces, orbit Saturn sufficiently fast. This includes a few large & charismatic "pre-Voyager" moons: Mimas, Tethys, Enceladus, and Dione.
  • Jupiter: All moons from Europa inwards (6 known), including Io, orbit Jupiter sufficiently fast.

It's possible that there are some satellites of dwarf planets that might also make this list, but I didn't spot any immediately. In general, a satellite of a dwarf planet would have to be orbiting its parent extremely closely to make this happen, since the orbital speed of a satellite is roughly proportional to $\sqrt{M/r}$, with $M$ the mass of the parent and $r$ the radius of the satellite's orbit.

Improve this answer
$\endgroup$
5
  • 2
    $\begingroup$ With Uranus, you can pretty much do the same calculation. When Uranus is near its solstices, the axis of the moons' orbits are pointing near the Sun, so you'll get loops, but they'll be near-perpendicular to Uranus' orbital plane. $\endgroup$
    – notovny
    Dec 17, 2020 at 21:14
  • $\begingroup$ +1 Can retrograde orbits be addressed as well, at least in passing? I'm having a hard time visualizing what happens. $\endgroup$
    – uhoh
    Dec 18, 2020 at 1:47
  • 1
    $\begingroup$ @uhoh: If a retrograde moon orbits its planet faster than the planet's orbital speed, its path will form a epitrochoid but with the "loops" pointed outwards instead of inwards. $\endgroup$
    – Michael Seifert
    Dec 18, 2020 at 2:48
  • $\begingroup$ @MichaelSeifert will it then have convex/concave transitions at any orbital speed, or are there also distance constraints? If there are, are they closer or farther from the planet than for prograde moons? $\endgroup$
    – uhoh
    Dec 18, 2020 at 2:53
  • $\begingroup$ +1. I'l add: Mars: orbital velocities about the planet are too small compared with the speed of the solar orbit. Earth: ditto. Need a giant far from the Sun/star for this phenomenon. $\endgroup$
    – Oscar Lanzi
    Sep 4, 2023 at 19:48

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .