2
$\begingroup$

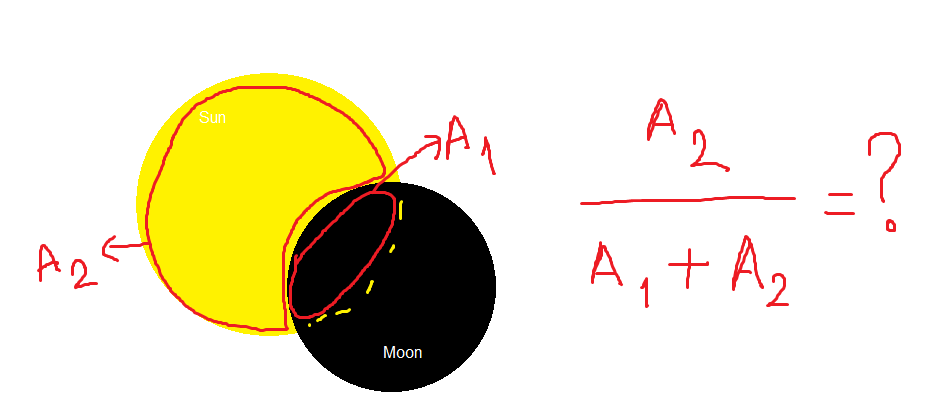
Assume a perfectly spherical Sun and Moon, and ignore atmospheric effects for simplicity. I'm an observer in the origin of my coordinate system, and I know the positions of the centers of the Sun and the Moon. I also know their radii.

At some point during an eclipse, my view of the Moon partially overlaps that of the Sun. Without the eclipse occuring, I could see 100% of the Sun in terms of solid angles. But since the view of Moon now partially blocks the Sun, I can see less. How do I calculate how much of the Sun is still visible to me at that time, as a ratio of solid angles, using the position and radii information?

This is not an observation-based question, since if I was actually observing the event, I could simply shoot a few photos and count the number of pixels and save myself the headache. This is more of a pure geometry question in that sense, to which I haven't found a simple and satisfying answer until now - meaning an (or a set of) equation(s) into which I can plug in the positions and the radii and get the result. To me it looks like that much information is just enough to get the answer, I just can't wrap my head around deriving the maths of it.

enter image description here

Improve this question
$\endgroup$
5
  • 4
    $\begingroup$ Might be useful: mathworld.wolfram.com/Circle-CircleIntersection.html $\endgroup$
    – BowlOfRed
    Commented Jun 4, 2023 at 22:43
  • 2
    $\begingroup$ Some of the geometry you need can be found in this answer of mine: astronomy.stackexchange.com/a/47731/16685 That tells you how to project the spheres to circles. BowlOfRed's link tells you how to get the overlap area. $\endgroup$
    – PM 2Ring
    Commented Jun 5, 2023 at 0:01
  • 1
    $\begingroup$ Do you mean the projected area (2D) or the surface area (3D)? $\endgroup$
    – planetmaker
    Commented Jun 5, 2023 at 10:59
  • 1
    $\begingroup$ @planetmaker My question is about the ratio of solid angles, so I'm asking the projected area (2D). $\endgroup$
    – arda güler
    Commented Jun 5, 2023 at 17:28
  • 1
    $\begingroup$ Ptolemy addressed the issue. You might want to read the Almagest, Book 6. $\endgroup$
    – Pierre Paquette
    Commented Jun 5, 2023 at 22:42

1 Answer 1

Reset to default
2
$\begingroup$

From BowlOfRed's Wolfrom link, the area of the asymmetric lense labeled $A_1$ in the O.P's post is $$A_1 = r^2\cos^{-1}\bigg(\frac{d^2+r^2-R^2}{2dr}\bigg) +R^2\cos^{-1}\bigg(\frac{d^2+R^2-r^2}{2dR}\bigg)- \frac{1}{2}\sqrt{(-d+r+R)(d+r-R)(d-r+R)(d+r+R)}$$

Here, $d$ is the distance between the Sun center and Moon center, $R$ is the apparent radius of the Sun, and $r$ is the apparent radius of the Moon.

Then the visible area of the Sun is simply:

$A_2 = \pi R^2-A_1 $

I suspect it's not possible to calculate this simply as a ratio of solid angles.

Improve this answer
$\endgroup$
1
  • 3
    $\begingroup$ It might be worht including that $r\approx R$ , and this approximation simplifies the formulae somewhat. $\endgroup$
    – James K
    Commented Jun 5, 2023 at 21:04

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .