3
$\begingroup$

For a school project I am currently determining the location of sagittarius A* (the supermassive black hole at the center of the milky way). I have analyzed about 1 million stars and their velocities. My thought was that if I look at the average velocity of all of those stars, it should be looking towards the black hole, since it has the biggest mass in the galaxy. But this isnt the case. Right ascension is off by about 40 minutes and declination is off by almost 35 degrees. I need to find what skews this in that direction or if I have made an error in my assumption. Thanks in advance!

Edit:

Dataset used: AT-HYG 2.4 from Astronomy Nexus https://astronexus.com/hyg/

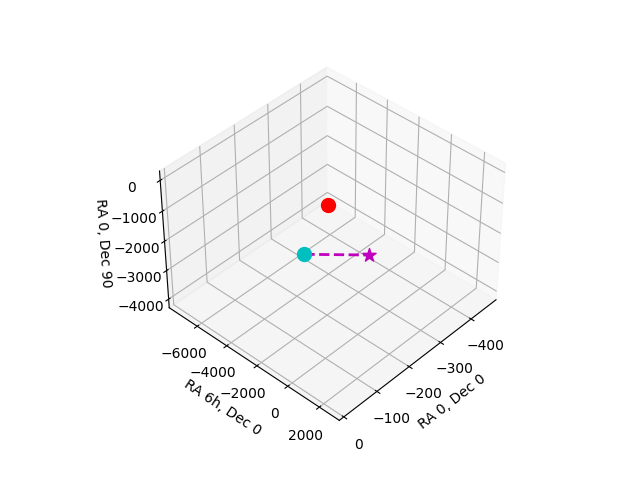
This dataset contains cartesian coordinates in parsecs with the sun being at (0, 0, 0). I have averaged out the coordinates and the velocities every 10 thousand stars. With the use of Python I plotted lines going through the average points and average velocities. Searching for a point where multiple lines come near eachother gave me the rough distance at around 6 thousand parsecs. For RA and Dec i averaged out all the data and converted it from cartesian to equatorial which gave me the result I wrote earlier.

I hope this helps.

Edit 2:

My approach was that if I calculate the average x, y and z coordinate and the x, y and z velocity of each star from the dataset it should point towards the center of the milky way. According to planetmaker it should point towards the rotation of the milky way and not towards the center, which is why I was off by so much.

Plotted: Image of the plot I made with python Red: black hole

Blue: average Coordinate

Purple star: average velocity (multiplied by 5 for visibility)

The code I used is a jumbled mess which I am not proud of but I can share it if nessesary.

From here a new question arises: How do I best factor in the rotation?

Improve this question
$\endgroup$
5
  • 2
    $\begingroup$ You need to explain your sample, your data and method more clearly. $\endgroup$
    – ProfRob
    Commented Aug 4 at 17:38
  • 2
    $\begingroup$ Welcome to Astronomy Stack Exchange! Sampling bias - you can get all kinds of answers depending on which million you used. If they were just some handy sample available from some telescope, then certainly they'll be more of them on our side of the galaxy than the other side. If you have velocities, you should also have distances, so the extent of your sample bias should be straightforward to estimate. But like ProfRob says above, "you need to explain your sample, your data and method more clearly" before a helpful answer can be posted. $\endgroup$
    – uhoh
    Commented Aug 4 at 22:33
  • 1
    $\begingroup$ This looks like an interesting question. However I am not sure I understand your question / methology correctly. I'm not sure what you average. The average of a movement along a circle is not rest at the center, especially not when you don't have an evenly-spaced dataset. If the average velocity of more or less that of nearby stars will not point towards the Galaxy's center, but in roughly orbital direction around the center. I recon you will have to explain to us in great detail how you approach the problem, including your math & code and some plots to explain what you see. $\endgroup$
    – planetmaker
    Commented Aug 5 at 7:47
  • 2
    $\begingroup$ The central supermassive black hole is the most massive single object in our galaxy (that we know of). But its mass is very small compare to the total mass of stars (and gas) in the galaxy, or even just interior to the Sun's orbit. (BH $\sim 4 \times 10^{6}$ solar masses, versus several $\times 10^10$ solar masses for the stars. So unless you select stars very close to the black hole (as people using near-IR adaptive optics on 8-10m telescopes do), the velocities of the stars won't reflect the influence of the black hole. $\endgroup$
    – Peter Erwin
    Commented Aug 5 at 10:29
  • 1
    $\begingroup$ There's a useful online book for getting into the detail of galaxies and I'd suggest it to the OP. It's “Bovy J. Dynamics and Astrophysics of Galaxies. Princeton University Press, Princeton, NJ (in preparation)” $\endgroup$
    – StephenG - Help Ukraine
    Commented Aug 5 at 10:40

1 Answer 1

Reset to default
2
$\begingroup$

Your basic misconception is that the average velocity of the stars should be toward the center. Stars are in orbits around the galaxy. In an orbit, the average radial velocity is zero. The tangential velocity isn't zero.

The radial acceleration is toward the center, but that cannot be measured for stars in the solar neighborhood.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .