Cosmic events as standard candles

Question

Type 1a supernovae are used as standard candles. Are there other high energy cosmic events which can be used as standard candles. For example, collapse of neutron star into a black hole is also similar to type 1a.

In a galaxy, there are far more white dwarfs(candidates of type 1a) compared to neutron stars(I read somewhere that our galaxy contains around 200 million of them). Also, the energy released in neutron star collapse is not in visible spectrum(I don't think so that is the criteria for "standard candles"). Are these the reasons that neutron star collapse is not qualified as another type of standard candle?

Answer 1

_{Intro for the uninformed: A standard candle is an important concept in astronomy, helping to map out distances in the Universe. Since the observed flux $F$ of a light source decreases with distance $r$ by a known factor ($r^2$), if we know its intrinsic luminosity L, we can calculate the distance. For large distances, where bright sources are needed, we usually use supernovae (SNe). But the luminosity of a SN depends on the mass of its progenitor star which is not known in general. However, for a specific type of SNe — "type Ia" — it is known: This type are SNe that explode when a white dwarf accreting mass from a companion star exceed the mass threshold for explosion of $1.4\,M_\odot$.}

---

In addition to the gravitational waves discussed by Rob Jeffries, I can mention the following candidates for standard candles:

Type II supernovae

Type Ia SNe are so similar in luminosity because they all have (almost) the same mass when they go off. But there is also evidence that type II SNe can act as standard candles. As is also to some extent the case with Ia, their lightcurves (how the luminosity changes with time) are not identical, but can be standardized using the so-called Philips relation (see e.g. Kasen & Woosley 2009).

GRB supernovae

Gamma-ray bursts as a whole are too diverse to be used as standard candles, but when an associated SN is detected, it becomes a standard(izable) candle (Li & Hjorth 2014).

Quasars

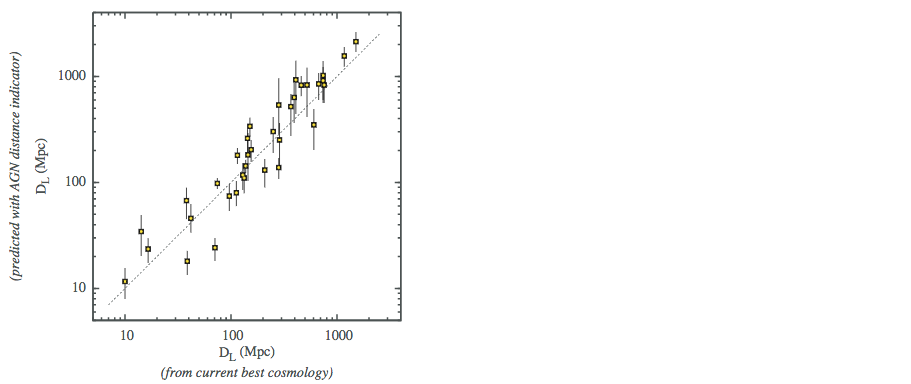
My favorite candidate are quasars. This technique doesn't rely on the Philips relation. Quasars are caused by gas accreting onto a supermassive black hole in the center of galaxies, resulting in an "active galactic nuclus" (AGN) with extreme energy outputs (easily over $10^{12}\,L_\odot$, and even up to $10^{14}$–$10^{15}\,L_\odot$; Ibata et al. 1999). It turns out that there is a correlation between the absolute luminosity of the AGN and the size of its broad-line region (BLR), i.e. the region around the quasar where fast-moving gas clouds absorb the continuum$^1$ of the quasar and emit lines$^2$, e.g. H$\alpha$ (Watson et al. 2011). The reason is that the size of the BLR is determined by the depth that the ionizing radiation from the quasar can penetrate into the BLR, which is proportional to the square root of the luminosity. The figure below (from Watson et al. 2011) shows the relation between distances ($D_L$) determined by this technique and distances obtained from type Ia SNe.

Advantages over supernovae

A huge advantage of quasars over SNe is that they don't disappear after a few weeks, meaning that if e.g. we want to refine some measurement, we can go back and observe it again at any time. Another advantage is that quasars, being so luminonous, can be detected out to much larger distances (roughly to $z\simeq4$) than SNe (which are only observed ut to $z\simeq2$).

Reverberation mapping

Since quasars are so far away, the BLR, being less than a parsec in size, cannot be resolved. But luckily, a technique call "reverberation mapping" allows us to determine the size:

The spectrum of the quasar consists of a continuum with spectral lines. Quasars vary in luminosity on rather short timescales. If we measure a quasar's luminosity regularly over some period of time, we get a so-called "lightcurve". But since the lines are created at a distance from the source, a given "bump" in the lightcurve (i.e. a temporary increase in luminosity) does't show up in the continuum and the lines at the same time. Instead, there is a delay, corresponding to the extra distance that the light had to travel from the quasar to the cloud reflecting$^3$ it.

In the figure below, blue shows the continuum, while red shows the lines. The lines are seen to lag behind the continuum, since they first had to travel from the quasar (black) to the clouds (magenta).

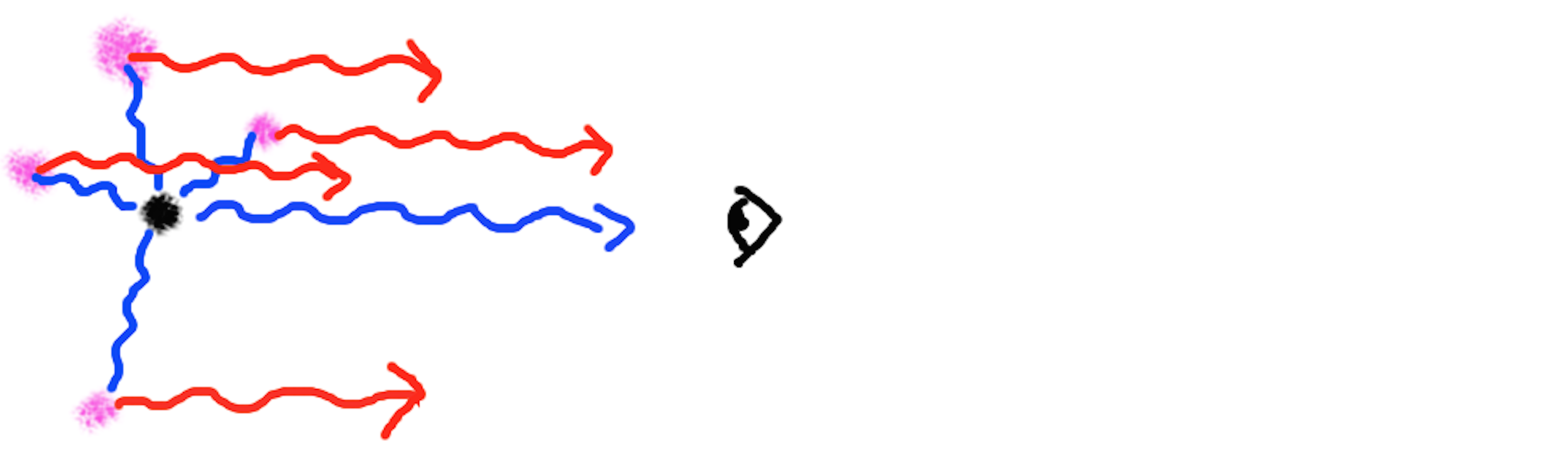
Since the clouds lie at a range of distances, they exhibit different time lags, effectively broadening the line:

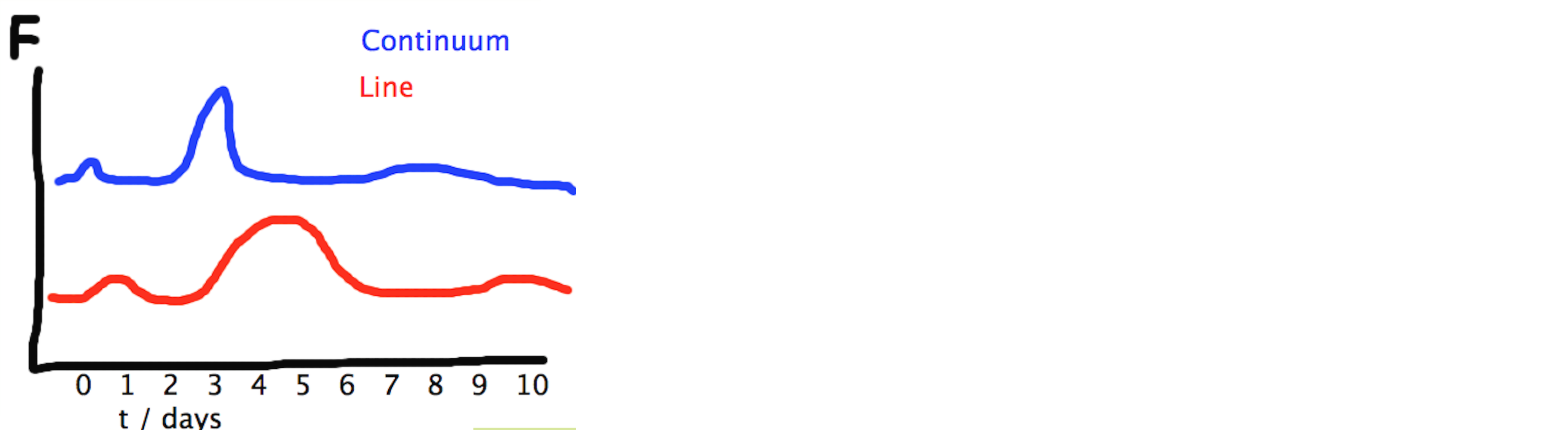
So, in the figure above, showing observed flux as a function of time, the light in the line increased in luminosity roughly 1.5 days later than the light in the continuum. This means that the BLR is roughly 1.5 lightdays (or ~250 AU) in radius.

---

$^1$_{I.e. the continuous and relatively featureless spectrum of many different atomic transitions and physical processes.}

$^2$_{I.e. features in the spectrum resulting from strong atomic transitions. For instance, an eletron falling from the second to the first excited states of a hydrogen atom emits a photon with the wavelength 6563 Å, called "H$\alpha$".}

$^3$_{The light isn't really "reflected". Instead, it is the high-energy photons (UV and X-rays) of the continuum that ionize the atoms in the clouds. When the ions recombine, they emit spectral lines.}

Answer 2

Gravitational wave sources, such as close, compact binaries can be treated as standard candles. Actually a better metaphor would be "standard sirens".

As was recently demonstrated by the recent detection of a gravitational wave signal from a merging black hole binary, the amplitude of the signal, combined with its frequency behaviour give you both the characteristics of the merging black hole and the distance to the source (independently of its redshift).

Such events are the most powerful events in the universe (I think more powerful than Gamma Ray bursts) - in this case $3M_{\odot}$ of mass was converted into gravitational waves in a fraction of a second.

To my knowledge, the collapse of a formed neutron star into a black hole has never been witnessed (at any wavelength).

EDIT: Well, what do I know - it turns out that there are certain classes of Gamma Ray Burst (GRB) events that may be explained by the accretion onto a neutron star in a close binary system, causing it to collapese (see Ruffini et al. 2014). They are often called "binary driven hypernovae". Although there is a possibility that these sources could become standard candles, the understanding of the relevant mechanisms is not sufficient for them to be considered so yet.