An ADS search on refereed astronomical papers including the words "Boötes Void" in the title returns only two papers in this millennium, in 2001 and 2002, and even these do not report any new data, but use data from the beginning of the 1990's. I can't find any newer references for the number density of galaxies in the Boötes Void in particular, but a typical value is roughly one-tenth of the average number density in the Universe.
Theoretical approach
I previously answered the similar question of the number of galaxies in an overdensity. This answer is based on an anlytical fit to (observationally calibrated) simulations of galaxy halo formation, giving the so-called halo mass function, i.e. the number of galaxy halos per halo mass. The total number $N_\mathrm{gal}$ of galaxies in a volume $V$ can be expressed as
$$
N_\mathrm{gal} = n_\mathrm{gal} \times V \times \delta,
$$
where $n_\mathrm{gal}$ is the average number density of galaxies in the Universe, and $\delta$ is the relative density in the volume. As I wrote in the other answer, $n_\mathrm{gal}$ is a number that impels you to define a lower threshold of galaxy size. The reason is that the lower you go, the more there are, and there is no formal threshold for how larger a clump of stars you need before you call it a galaxy. But as in the other answer, for the sake of this discussion we may use Small Magellanic Cloud-sized galaxies as our lower minimum. In that case, with $\delta\sim0.1$ and $V\sim236,\!000\,\mathrm{Mpc}^3$, the total number becomes
$$
N_\mathrm{gal,Böotes} = 0.17\,\mathrm{Mpc}^{-3} \, \times \, 236,\!000\,\mathrm{Mpc}^3 \, \times \, 0.1 \, \simeq \, 4000\,\mathrm{galaxies}.
$$
Units of galaxy number density
The way I write this result also answers your question 3: Galaxy number densities are almost always written in $\mathrm{Mpc}^{-3}$. In theoretical/numerical work, you'll often see the factor $h^3$ in front of the unit. This is simply the Hubble constant divided by 100 (i.e. $h=0.7$ for $H_0 = 70\,\mathrm{km}\,\mathrm{s}^{-1}\,\mathrm{Mpc}^{-1}$), allowing people to compare results more easily without knowing the exact value of $H_0$.
Observational approach
The observations of the Boötes Void are old, and seem to have been carried out on 1m-class telescopes. Hence, they won't be able to observe the smallest galaxies. In addition to telescope specifications, weather, etc., the exact detection limit (in terms of a limiting magnitude $m_\mathrm{lim}$) depends on how long they integrate (i.e. expose). Without reading the old papers in detail, I can't say what this is, but a typical value for such surveys would be, very approximately, $m_\mathrm{lim} \sim 20$ (if someone has a more realistic value than this, please edit). That is, objects fainter (i.e. larger values due to the backward astronomical system) than $m=20$ would be missed.
The distance to the Boötes Void implies a distance modulus of $\mu\sim37$, so the minimum absolute magnitude is
$$
M_\mathrm{lim} = m_\mathrm{lim} - \mu \simeq -17,
$$
which is somewhere between the Small and the Large Magellanic Cloud.
The figure below (from Wyder et al. 2005) shows the local-Universe luminosity function for UV-selected galaxies.
That is, it shows the number density of galaxies at a given magnitude. For instance, it shows (with the green dashed line) that the number density (here called $\Phi$) of galaxies with magnitudes around $M=-17$ is roughly $10^{-2.5}\sim0.003\,\mathrm{Mpc}^{-3}\,\mathrm{mag}^{-1}$.
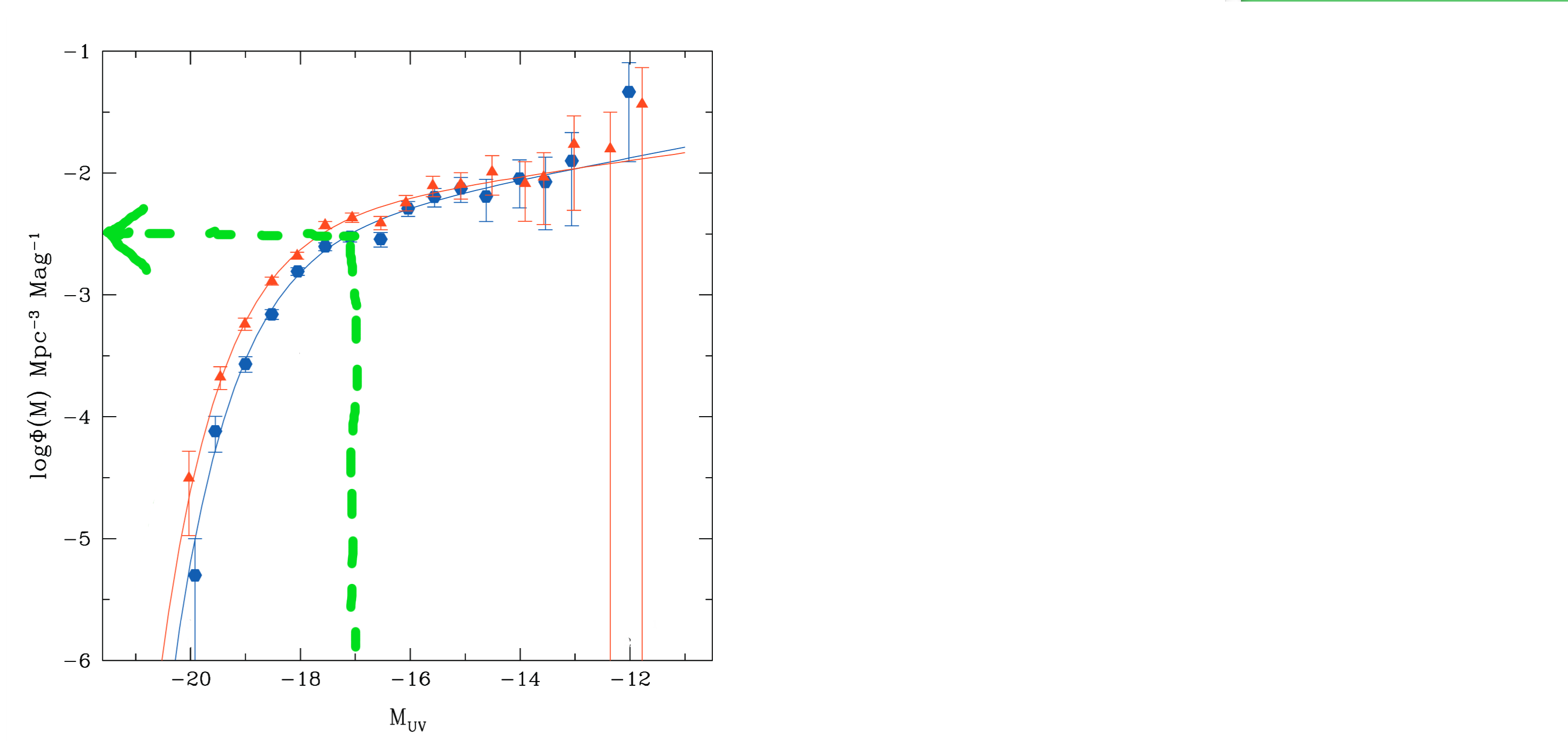
Integrating over magnitudes from the $M=-17$ doesn't change the 0.003 much, since the density of brighter galaxies quckly declines. I get 0.004, i.e. the number density of galaxies at least as bright as $M=-17$ is $0.004\,\mathrm{Mpc}^{-3}$, smaller than the theoretical result above by 1.5 orders of magnitude. Multiplying this by the volume $V$ and the relative density $\delta$ yields $N_\mathrm{gal,Böotes} \simeq 100$ galaxies, not far from the 60 you quote.
To conclude, the number 60 seems in rough accordance with what is expected observationally, but theoretically, we'd expect there to be many more galaxies (although they're very small).
Location of the galaxies
They detected galaxies seem to be lying in a "tube" extending across the void. In general, galaxies and the underlying dark matter mass field tend not to be evenly distributed, but to form knots, sheets and filaments, separated by voids. My guess is that this "tube" is such a filament. Outside this filament, the void is voidier, but not completely void. There will still be galaxies, although few and small.
