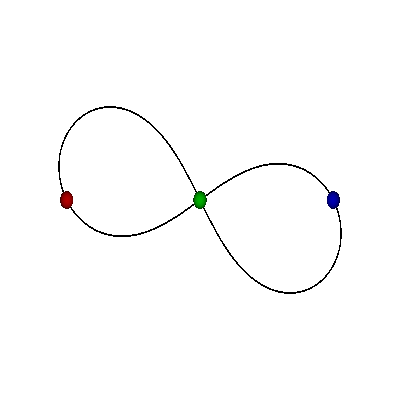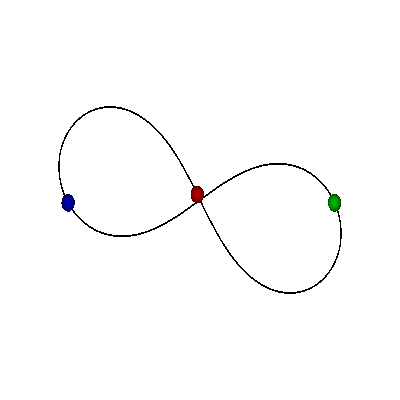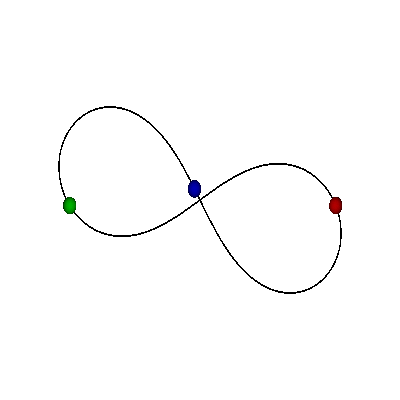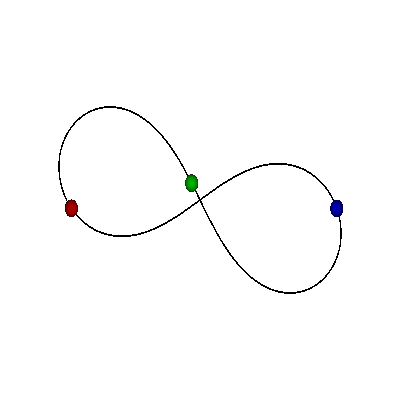
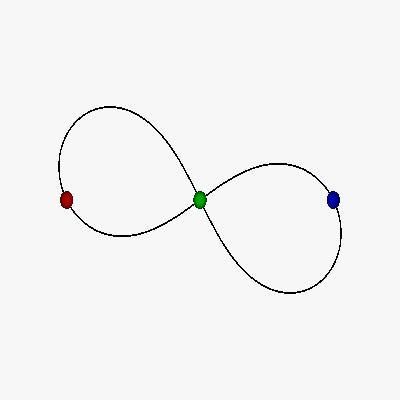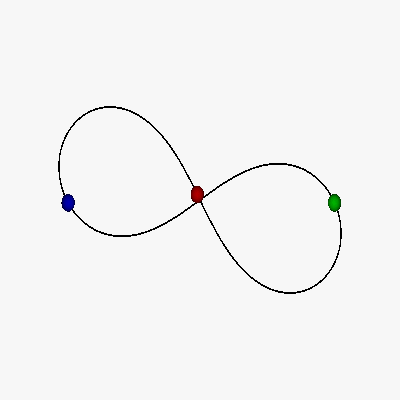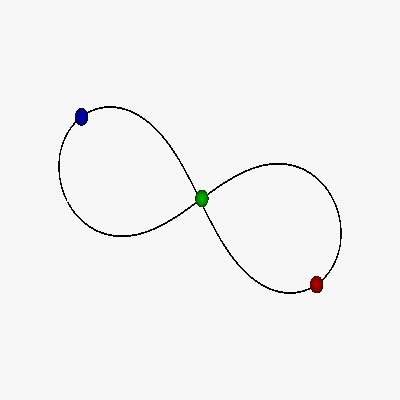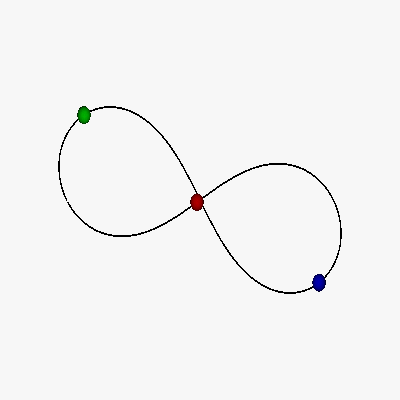
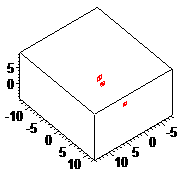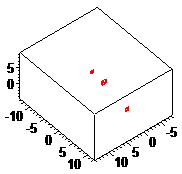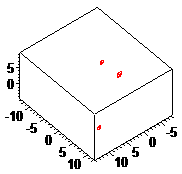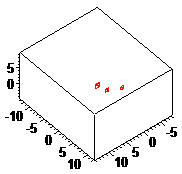
If this is a posible periodic solution for a three-body problem, can anybody tell me where is the center of mass of the system? As we know, in a n-body problem the bodies orbit around their center of mass, but here I don't know where it is. Can anybody help me? Thanks.
There may be some three-body periodic solutions that orbit around a common point, but in general they get a little crazy-looking and may not always contain an immediately obvious center of mass from casual observation. But of course it will always exist.
If you watch closely, you'll see that whenever one body reaches the intersection point, all three are on a straight line with the other two equidistant (symmetric). If you look where this orbit was originally described mathematically, they probably defined the initial conditions with the green one in the middle - so that the y coordinates of all there were zero and the line at that point is horizontal.
At those moments (all six permutations are present) the center of mass is at the intersection point since the masses are equal.
Since there are no external forces, the center of mass doesn't change in between those moments, so the center of mass is always at the intersection point.
If this is a possible periodic solution for a three-body problem?
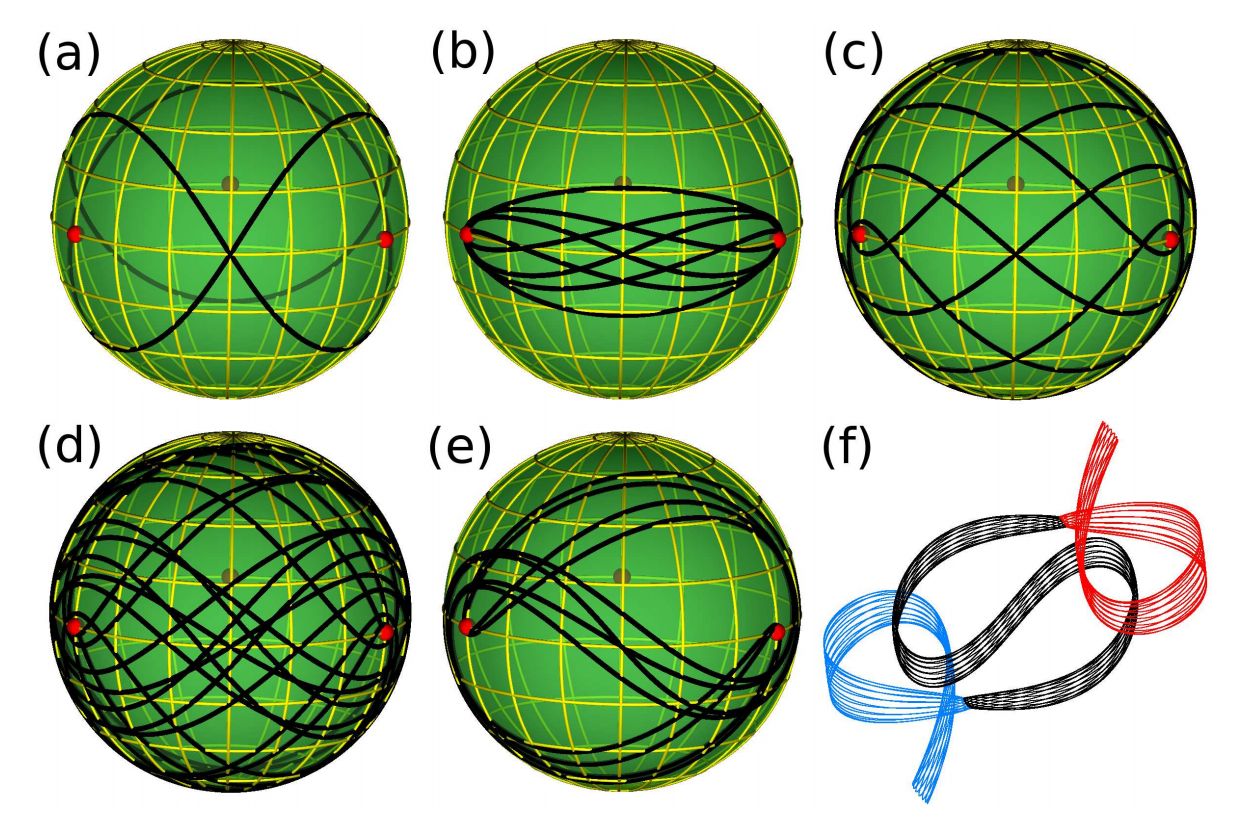
What you've posted here is the classic "figure 8" orbit for a 3 body system. Originally the 3-body system was an unsolvable mathematics problem, until people started using computers to do the math for us. Recently, a set of 13 "stable" 3-body orbits have been found which were published in this paper. A diagram illustrating these orbits (with the accompanying caption) is shown below.
FIG. 1: The (translucent) shape-space sphere, with its backside also visible here. Three two-body collision points (bold red circles) - punctures in the sphere - lie on the equator. (a) The solid black line encircling the shape sphere twice is the figure-8 orbit. (b) Class I.A butterfly I orbit (I.A.1). Note the two reflection symmetry axes. (c) Class I.B moth I orbit (I.B.1) on the shape-space sphere. Note the two reflection symmetry axes. (d) Class II.B yarn orbit (II.B.1) on the shape-space sphere. Note the single-point reflection symmetry. (e) Class II.C yin-yang I orbit (II.C.2) on the shape-space sphere. Note the single-point reflection symmetry. (f) An illustration of a real space orbit, the “yin-yang II” orbit (II.C.3a).
It is hard to tell, but the gif you've posted is represented by (a) above. So I suppose the answer to your question is, yes, the figure 8 is a possible solution for the 3-body problem. However, I should caution that there is no possible 3-body system which is stable for long periods, especially if those three bodies have nearly equal masses. Our Sun/Earth/Moon system is only so stable because $M_{Sun}>>M_{Earth}>>M_{Moon}$ and they all effectively have binary orbits. For three, roughly equal mass stars, your system gets wildly unstable very quickly. Pretty much any perturbation will knock your system out.
Can anybody tell me where is the center of mass of the system?
As uhoh very nicely reasons out, the center of mass is in the center of the figure 8. Note however, that your system appears to show all bodies orbiting in a single plane. Obviously the real universe is 3 dimensions and very likely these objects would be orbiting in 3 dimensions, making the location of the center of mass more complicated. In the image above, you can see that the orbits oscillate up and down periodically, but they will do so in such a way that the center of mass is in the midplane of this oscillation, as well as the center of the figure 8.
As we know, in a n-body problem the bodies orbit around their center of mass, but here I don't know where it is. Can anybody help me? Thanks.
All orbits are n-body systems. There's essentially no perfect 2 body systems anywhere in the universe. Our solar-system is an N-body, not a 2 body, but nearly all the orbits in our solar system approximate 2 body orbits.
Long term stable N-body systems are very similar to 2-body systems. Unstable or chaotic N-body systems like this one on Wikipedia, don't last very long. They turn into stable systems and in the process, usually eject some objects.
While I would be hesitant to try to prove this mathematically, the figure 8 orbit reminds me of balancing a pencil on it's tip. Slight variations tend to grow over time and it destabilizes quickly. (cool video on Pencil-balancing)
