I suppose it is the Saturn system's barycenter which has been located gravitationally. At this level of precision I suppose that the moons', especially Titan's tidal effects must be considered. ..., so I wonder if these too are being considered at the 4 km precision level, and overall exactly how the point located is defined?
Short answer: No.
As pointed out in the comments by @AtmosphericPrisonEscape (Oct 5 '16 at 14:42) and @userLTK (Oct 6 '16 at 9:25) - it is a barycentric measurement.
Other references:
Solid-body tides on the Moon
Is Io's orbit or rotation affected by its volcanism?
The Barycenter coordinates are an average, a mean, small perturbations are smoothed in the calculations by various factors such as: measurement accuracy, use of the International Celestial Reference Frame (ICRF), even the Cholesky whitening (Source: "Measuring the mass of solar system planets using pulsar timing" (21 Aug 2010)) used in some of the calculations.
"In astronomy, barycentric coordinates are non-rotating coordinates with the origin at the center of mass of two or more bodies. The International Celestial Reference System is a barycentric one, based on the barycenter of the Solar System.
...
Relativistic corrections
In classical mechanics, this definition simplifies calculations and introduces no known problems. In general relativity, problems arise because, while it is possible, within reasonable approximations, to define the barycenter, the associated coordinate system does not fully reflect the inequality of clock rates at different locations. Brumberg explains how to set up barycentric coordinates in general relativity in his book "Essential Relativistic Celestial Mechanics".
The coordinate systems involve a world-time, i.e. a global time coordinate that could be set up by telemetry. Individual clocks of similar construction will not agree with this standard, because they are subject to differing gravitational potentials or move at various velocities, so the world-time must be slaved to some ideal clock that is assumed to be very far from the whole self-gravitating system. This time standard is called Barycentric Coordinate Time, or TCB.
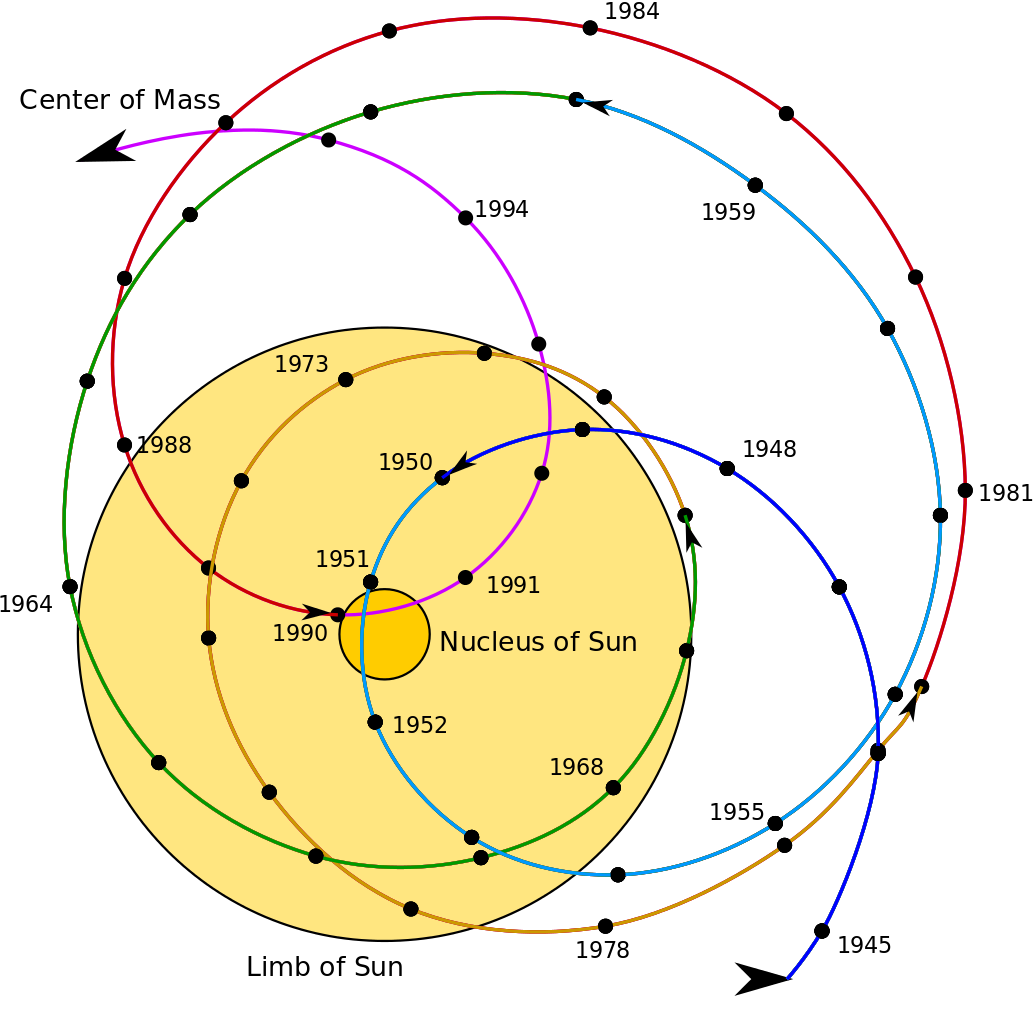
Example: Motion of the barycenter of the Solar System relative to the Sun, 1945–1995.
In the paper "Uncertainties in the JPL Planetary Ephemeris", by Folkner, on page 47, is shown this chart of the uncertainty in right ascension, declination, and distance of the barycenters of the Earth and Saturn systems calculated for the years 1950 to 2050:
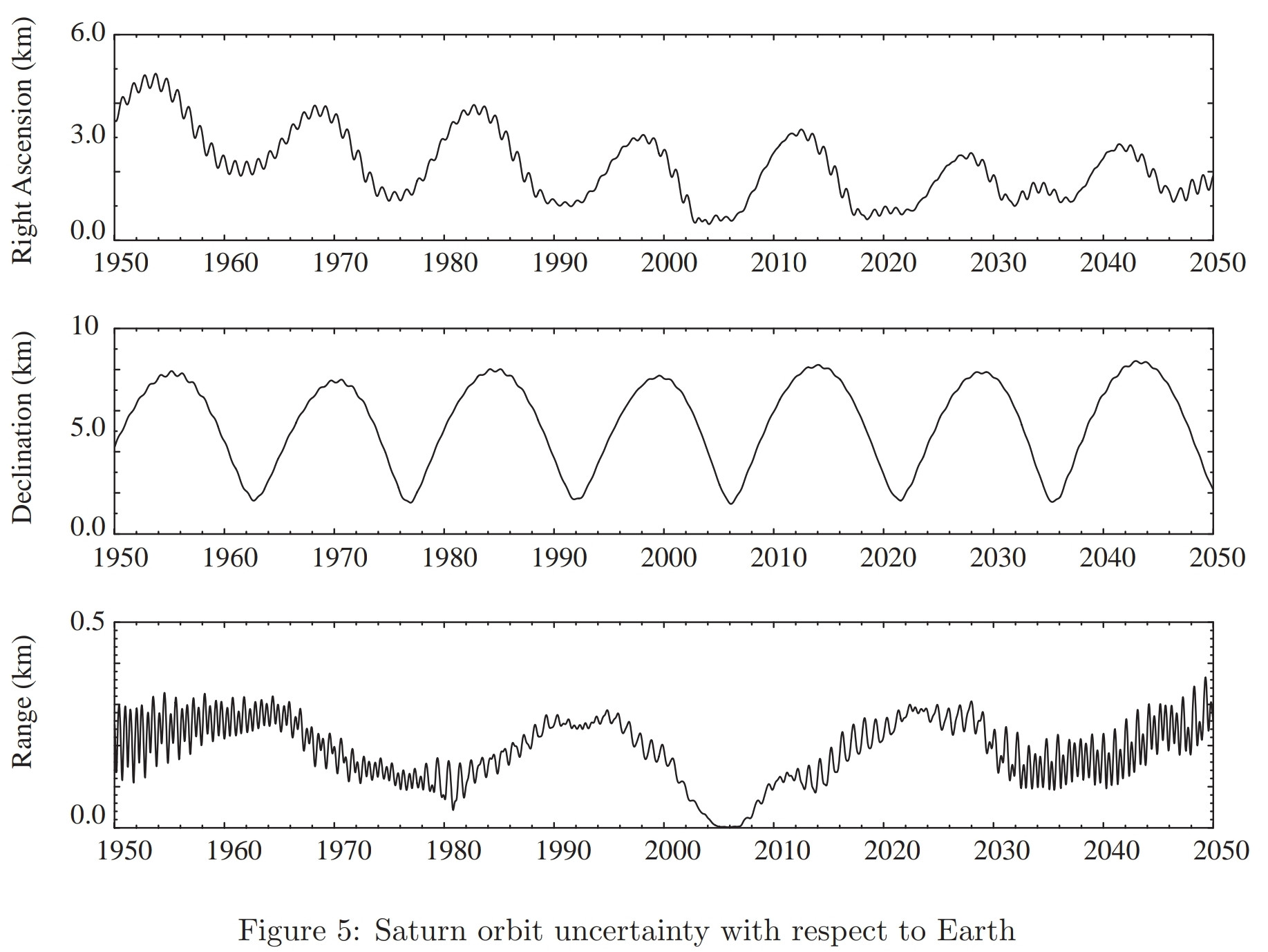
Celestial mechanics and the n-body problem take a long time to solve.
The paper associated with these measurements of Saturn is titled: "VLBA Astrometric Observations of the Cassini Spacecraft at Saturn" (1 Dec 2010), on page 2 it says:
"These observations provide positions for the center of mass of Saturn in the International Celestial Reference Frame (ICRF) with accuracies ∼0.3 milli-arcsecond (1.5 nrad), or about 2 km at the average distance of Saturn.
...
The DE 422 post-fit residuals for Saturn with respect to the VLBA data are generally 0.2 mas, but additional observations are needed to improve the positions of all of our phase reference sources to this level. Over time we expect to be able to improve the accuracy of all three coordinates in the Saturn ephemeris (latitude, longitude, and range) by a factor of at least three. This will represent a significant improvement not just in the Saturn ephemeris but also in the link between the inner and outer solar system ephemeredes and in the link to the inertial ICRF.".
I might return for an edit if there is interest in this Q&A.

