Math behind a uv plot in interferometry?

Question

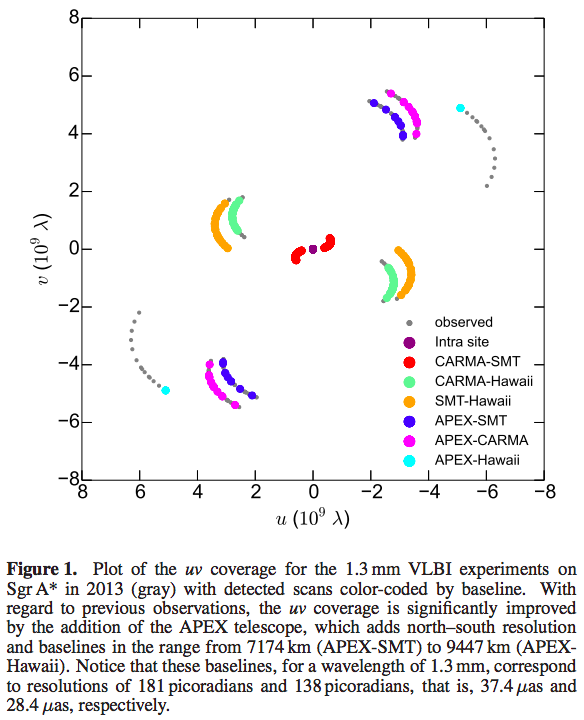
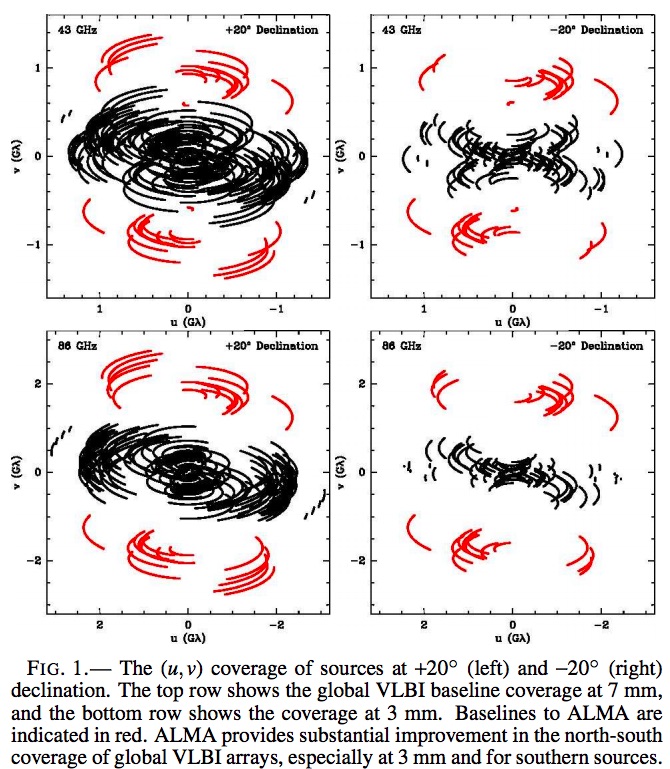
I've seen standard uv plots used discussions of interferometric array designs regularly, and I have a vague idea that each arc may correspond to a pair of elements within the array, and the coordinates $u, v$ in the plot may be something like the path length difference between the source and the two elements projected into some cartesian coordinates, and that the length of the arcs corresponds to the total duration of the observation, but it's all still fuzzy.

Since the elements may be on topography or be so far apart (as in the example below) that the curvature of the Earth is even larger, the formal definition of the $uv$ plane should take this into consideration.

There are several more examples of uv plots and comparisons to point spread functions in the SlidePlayer presentation Array Design and Simulations.

Question: What is the math behind the construction of $uv$ plots in radio astronomy? A source that explains it and shows how one can construct them would be extremely helpful, as will any intuitive hints.

below: From the recent ArXiv preprint Detection of Intrinsic Source Structure at ~3 Schwarzschild Radii with Millimeter-VLBI Observations of Sagittarius A* (also ApJ Open Access.

below: From the 2016 ArXiv preprint High-Angular-Resolution and High-Sensitivity Science Enabled by Beamformed ALMA

Answer 1

Rick Perley gives a lecture about interferometry to a biannual NRAO Synthesis Imaging Workshop. Part 2 of his 2016 talk discusses u,v plane coverage. The 2016 IRAM Millimeter Interferometry School lectures by J. Pety and F. Gueth may also help.

Let $\hat{u}, \hat{v}, \hat{w}$ be orthogonal unit vectors with $\hat{w}$ pointing from Earth to the radio source, $\hat{v}$ oriented northward, and $\hat{u}$ oriented eastward. Let $B_{ij}$ be the baseline vector between antennas $i$ and $j$ in the array. Then $(B_{ij} \cdot \hat{u}, B_{ij} \cdot \hat{v})$ and $(-B_{ij} \cdot \hat{u}, -B_{ij} \cdot \hat{v})$ are points in the u,v plot, and $B_{ij} \cdot \hat{w} / c$ is a time offset between the signals at $i$ and $j$.

If both antennas observe the source for several hours, $B_{ij}$ rotates with the Earth, and the two points in the u,v plot grow into elliptical arcs. The shape of those ellipses depends on the declination of the source, so the same array will have different u,v coverage for sources at different declinations.

The interfering signals from the array are combined in a visibility function $V(u, v)$. The image of the source is an inverse Fourier transform of $V$, typically deconvolved using an algorithm called CLEAN. To minimize image artifacts, a diverse set of baseline lengths and orientations, without large gaps in u,v coverage, is desirable.