What is the escape velocity of our Galaxy relative to?

Question

I've been thinking that everything has a relative escape velocity, if it has Mass, seemingly. You can escape orbit around the earth by accelerating enough. You can escape the orbit of the sun by accelerating enough. Heck, this is kind of a stretch, but even electrons can escape an atom relative to how much electric charge the nucleus holds. What would it be like to escape the orbital velocity of our Galaxy-- and what exactly would it be relative to-- a black hole at the center of the Galaxy?

The quote was:

Solar system's orbital velocity is estimated at roughly 220 km/s, and galactic escape velocity for our vicinity at about 537 km/s. So in the direction of Solar system's velocity vector, velocity required to escape Milky Way is ~ 317 km/s. And much more, if this Solar system's own orbital momentum cannot be used to full extent and a launch in other directions is required. This is of course assuming you can launch on a trajectory that avoids getting too close to gravitational influence of other solar systems.

How in the world do you calculate the escape velocity of other galaxies let alone our own-- do we know what the solar systems in the Milky Way orbit and how they orbit it?

Answer 1

One way to think of the Escape velocity is to imagine it backwards.

Instead of a rocket being fired into space, think of the same rocket, starting at rest relative to the Earth at a great distance (for this thought experiment pretend the sun, doesn't exist and the rest of the universe is empty), then let it fall to the ground. No matter how far away you start, the speed when the rocket hits the Earth will always be 11km/s or less.

So if you start with 11km/s or more at the Earth's surface, you have enough energy to coast forever into space. The escape velocity at the surface is 11km/s, the escape velocity would be different, and lower, if you were already in a high orbit.

We can do the same thought experiment with a galaxy. We know, roughly the distribution of mass in the galaxy (most is in a large blob of dark matter with the visible disc inside it) Now take a rocket ship a very great distance from the galaxy, and allow it to fall. As it passes the sun, the rocket will be moving at 537km/s relative to the centre of the galaxy. (there is a black hole there, but it is tiny compared to the galaxy, so has very little effect on the gravity of the galaxy)

If you know how the mass of a galaxy is distributed we can get a good idea of how fast something would be travelling at any point, if allowed to fall. And by the same argument as above, that gives the escape velocity at that point.

The escape velocity around the sun is about 1/600 of the speed of light. The journey to leave the Milky way is at least 30000 light years long, This means it is going to take about 20 million years to reach the edge of the galaxy, since you will be getting slower as the galaxy's gravity pulls you back. Space (as has been observed) is big.

Answer 2

James K's answer is great, I just want to offer a few definitions:

Any mass $M$ — whether a be point mass like a planet or an extended mass like a galaxy — has an associated gravitational potential $\Phi(\mathbf{x})$. This is defined as the energy needed to bring a unit mass from the point $\mathbf{x} = \{x,y,z\}$ to infinitely far away from $M$.

The escape velocity $v_\mathrm{esc}$ is defined at the point $\mathbf{x}$ to be the velocity an object needs to achieve just enough kinetic energy to overcome the depth of the potential "well" and get infinitely far away from $M$, without having to spend more energy propelling itself.

You ask "What is the escape velocity calculated relative to?" You can thus say that it's calculated from any point you wish, relative to infinitely far away (in practice, just far enough that gravity is no longer dominated by the galaxy, planet, or whatever, but is dominated by other objects).

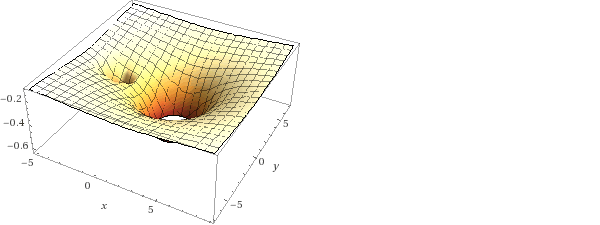
The potential can be a hard concept to visualize, especially in 3D, but you often see it depicted in a 2D analogy as a depression in an otherwise flat surface. You can then think of $v_\mathrm{esc}$ as the kick you need to give a ball to make it roll up the well, without rolling back. Here's an illustration of the combined potential of Earth and Moon (from Wikipedia):

Mathematically, you calculate the escape velocity as $$ v_\mathrm{esc}^2(\mathbf{x}) = 2|\Phi(\mathbf{x})|. $$

Outside of a spherically symmetric object (e.g. Earth), this evaluates to $v_\mathrm{esc} = \sqrt{2GM/r}$, where $r$ is the distance from the center of the mass. For an extended mass (e.g. the Milky Way), the expression becomes more complicated and depends on the density profile $\rho(\mathbf{x})$ of stars, gas, and, in particular, dark matter. That is, the exact distribution of its component matters.

By observing the velocities of various objects (stars or luminous gas clouds) in a galaxy, we get the rotation curve and can then map the density profile. Given $\rho(\mathbf{x})$, we can then calculate the potential by solving Poisson's equation: $$ \nabla^2\Phi = 4\pi G \rho, $$ where $\nabla^2$ is a mathematical description$^\dagger$ of how the steepness of the potential changes from place to place.

For galaxies (or more specifically the gravity-dominating dark matter halo in which the galaxy resides), it often it turns out that, to a good approximation, $\rho(\mathbf{x})$ is given by a so-called NFW profile, but many other profiles are seen as well. The exact density profile can of course only be known if the exact mass and position of every single star, planet, or even gas particle, is known, but on large scales, the average profile is an excellent approximation.

And once you have the potential, you have the escape velocity.

---

$^\dagger$_{$\nabla^2$ (also written $\Delta$) is called the Laplace operator, and is defined as the divergence ($\nabla\cdot$) of the gradient ($\nabla$), where the gradient itself is $\equiv \{\partial/\partial x, \partial/\partial y, \partial/\partial z\}$.}

Answer 3

The above answer by pela is correct. Mathematically, one calculates the escape speed of a point mass as

$V_{esc}(x)=\sqrt{2|Φ(x)|}$, where $\phi(x)$ is the gravitational potential

and for a spherically symmetric object

$V_{esc}(r) = \sqrt{2GM/r}$

will be the value for the escape speed [velocity implies direction but this is an energy question]. What is assumed here is a spherical shape, and the escape speed is desired for a point mass located at the radius r from the sphere's center. The mass M = M(r) here is a function of r. [The hitch/caveat is that our galaxy is not a sphere but is rather pan-cake shaped. So the travel-direction can indeed come into play due to the non-spherical galactic shape.] If the further assumption of isotropy is made, for simplicity, a nice peculiarity arises in that only the galactic mass portion contained in a sphere of radius r is needed. Masses located at radii greater than the radius r of interest will tend to accelerate the point mass if it lies "ahead" of the point mass and masses "in back" [at radii greater than r] will decelerate the point mass in exactly the same amount so as to be make irrelevant any galactic masses that lie at radii greater than r from the galaxy's center.

Answer 4

You are mixing elements in your question. You talk about acceleration in your question...there is no such thing as "escape acceleration"...because, well, any acceleration (so long as you maintain it long enough) would keep you increasing velocity (that is what acceleration is: how fast your velocity changes with time). So a 0.0001 m/s2 acceleration would eventually get you fast enough to escape the gravitational force of a body. You also talk about mass...to be clear, to calculate the escape velocity FROM something, the only mass that matters is the mass of the thing you want to escape (the mass of the object escaping is irrelevant to calculate the escape velocity, obviously the more massive the object is the more energy you will need to get it to the escape velocity, but the escape velocity does not change on the mass of the object escaping). So to calculate the escape velocity of anything, you just need to calculate what exact velocity you will need so you will never fall back...anything faster than that will also keep you from falling back of course. You only need basic calculus to calculate the escape velocity and really the only information you need is the mass of the moon, planet, solar system you are trying to escape...so to calculate the escape velocity from the Milky Way you need to estimate the mass of the Milky Way...notice that unlike Earth (where basically you always start at the surface) you can start inside the galaxy, so you only need to concern yourself with the mass inside the galaxy respect to where you are. Since the solar system is more than half way out... you would need a smaller escape velocity from Earth that you would need if you are near the center of the galaxy, to be able to escape from there you need a velocity large enough to get you to Earth and then you still need velocity to keep going. Astronomers have estimates of the mass of the galaxy and knowing your position on the galaxy you can estimate the interior mass of the galaxy and hence get a pretty good idea of what speed you would need to escape the galaxy.

Once you understand the concept of escape velocity...think about this...if your have a escape velocity from one object...if the object becomes more massive...you need a higher escape velocity, right? (for example to escape Earth you need a higher speed than to escape the Moon)...well...at some point and object is so massive that the escape velocity you would need is the speed of light...since you can't go faster than the speed of light, that also mean that if an object then has even more mass than that...then you would theoretically need a higher than speed of light velocity to escape...but since you can't travel faster than the speed of light...then you can't escape!! And that's what a black hole is...an object so massive that the escape velocity from it theoretically bigger than the speed of light...so nothing can't escape!