I assume you're referring to the recent press release about the quasar J043947.08+163415.7, observed recently using Hubble. The paper about the observations details how the authors measured the distance to the quasar, by calculating its redshift, a quantity that describes how the wavelength of light appears to change based on whether the object is moving relative to an observer. On cosmological scales, redshift can then be converted to distances. We usually see quasars at $z>0.1$.
As is usually the case, the quasar's redshift was determined by looking at spectral lines - in this case, Mg II emission around 21000 angstroms (see in particular the inset):
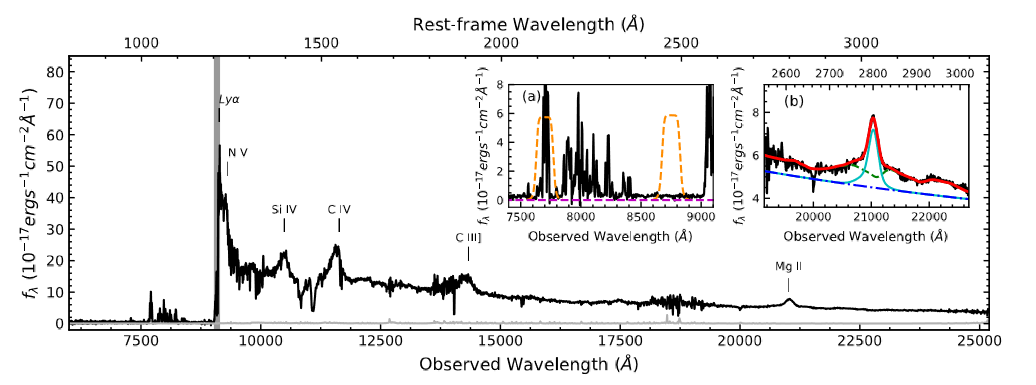
This emission line was observed to have a different wavelength than it would if the quasar was at rest, enabling the astronomers to calculate its redshift: $z=6.511\pm0.003$.
This is the typical process used to determine redshift, and therefore distance - well, not necessarily using that particular Mg II line, of course, but nonetheless the use of spectroscopy to measure how the location of spectral lines changes. The difference between "early" and "late" isn't quite clear, and probably varies depending on who you're talking to. For instance, someone studying the Big Bang might refer to the period before recombination as "early" - although we're seeing the quasar as it existed much later than that; it wasn't around for recombination.
I'd like to note that it's somewhat misleading to call this quasar the brightest (or even brightest known) quasar in the universe, because - as that press release notes - the gravitational lensing by a foreground galaxy, which enabled the object to be discovered, also magnified its brightness. It appears to have a brightness of 600 trillion solar luminosities, but without the lensing, that number is reduced to 11 trillion solar luminosities - a much more modest figure.
