4
$\begingroup$

I'm starting to learn Kepler's Astronomia Nova using William Donahue's translation . In the translator's introduction, it is demonstrated how Kepler did his parallax computation. I failed to understand the following paragraph:

enter image description here

specifically:

1) nonagesimal's definition: there are 2 points of intersection between the ecliptic and the horizon: one in the East, the other in West. another ambiguity is the direction of the $90^\circ$

2) I can't see why the altitude of the nonagesimal is "the same as the angle between the ecliptic and the horizon". It does make sense to me, but I'm missing a kind of "proof" for that.

3) Actually I also failed to understand what follows in that paragraph - but maybe your explanation for the points above, will clarify that for me.

your help is very much appreciated

Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

It looks like the assumption regarding the 90° point is that the amount of the ecliptic visible is 180°. So the 90° direction is the halfway point.

The nonagesimal is on the ecliptic, so the altitude approximates the arc. The wording in the initial part has that correct, but then it goes on to say "the same as the angle" - this seems to be a mistake. The arc is directly related to the angle, yes, but it is not the angle.

Improve this answer
$\endgroup$
2
  • $\begingroup$ thanks!. the thing is the visible ecliptic is not necessary $180^\circ$. but I think we can assume that is indeed the assumption. anyway, not sure I understand you last remark about the angle. unfortunately I still missing something with that paragraph. $\endgroup$
    – d_e
    Commented Feb 14, 2019 at 17:06
  • $\begingroup$ I read further on and now understand what you were saying - yes, the wording in the article is not correct $\endgroup$
    – Rory Alsop
    Commented Feb 14, 2019 at 20:18
1
$\begingroup$

finally I think I grasped this. As a novice, I did not understand some basic definitions that made that much harder for me to understand the paragraph. It might be a good idea to provide here an answer for reference to others that might encounter this issue.

1) parallax in this section refers to geocentric parallax. i.e where one point of observation is the surface of the earth and the second is it's center. Evidently, in the Zenith the geocentric parallax vanishes.

2) the longitude and latitude in this section refer to the lines in the Ecliptic coordinate system - where the ecliptic is of latitude 0 of course. [I'm still not sure as to why they used that system back then, instead of the more natural (at least to me ) Equatorial coordinate system. (maybe it was easier to calculate eclipses and plants transits using the ecliptic system)]

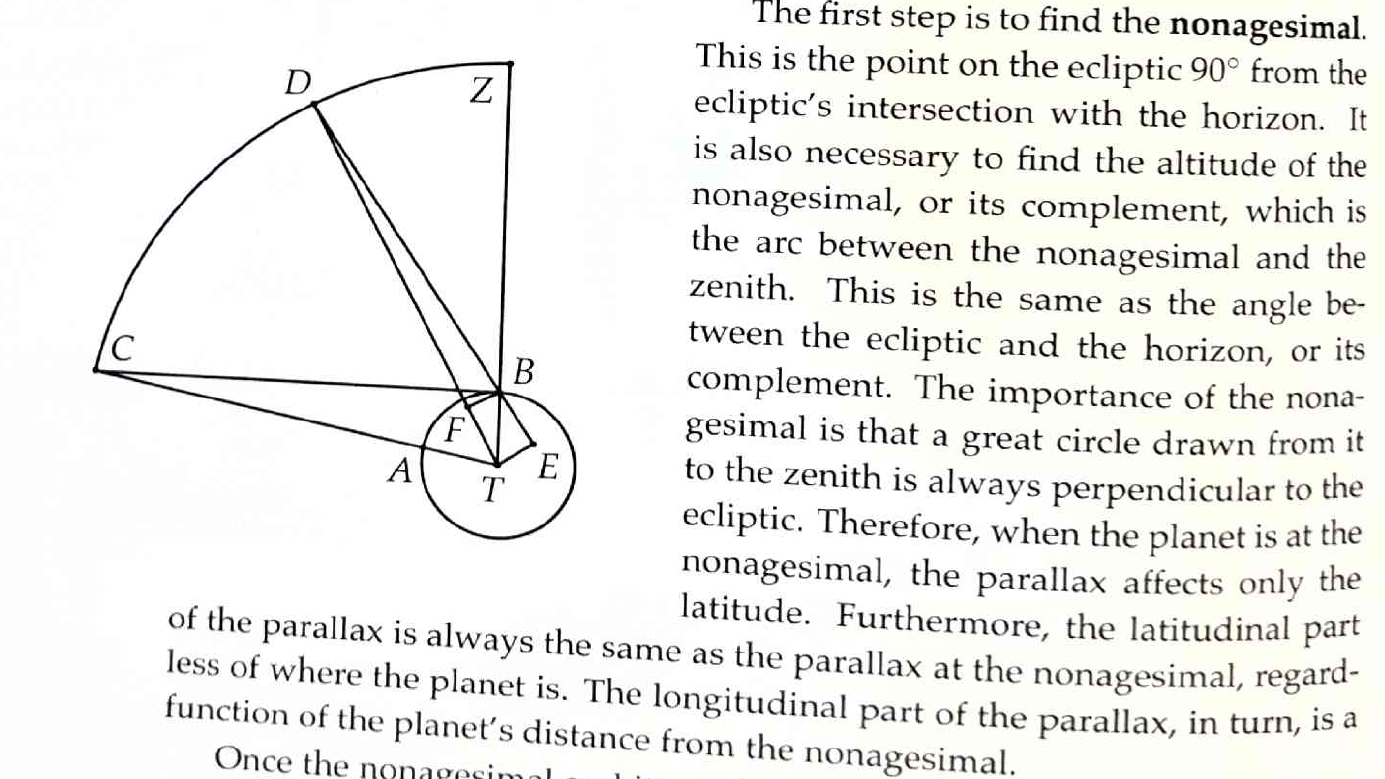
Now, for the nonagesimal - it is simply the highest point (closest to the Zenith) in the ecliptic at any given moment. it might be helpful here to note that nonagesimal is not the Midheaven. Simply because the highest point in the ecliptic does not necessarily intersect the meridian.

Making those definitions clear, the rest in that paragraph follows: the great circle drawn from the Zenith through the nonagesimal is simply a longitude line in the ecliptic coordinate system since it is perpendicular to a latitude line (the ecliptic being latitude 0). so since the the plane being defined by the earth's center, the observer point on the surface and the nonagesimal, is a plane of one longitude, then the parallax could not affect the longitude at all.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .