Trouble with plotting a graph for the Planck function

Question

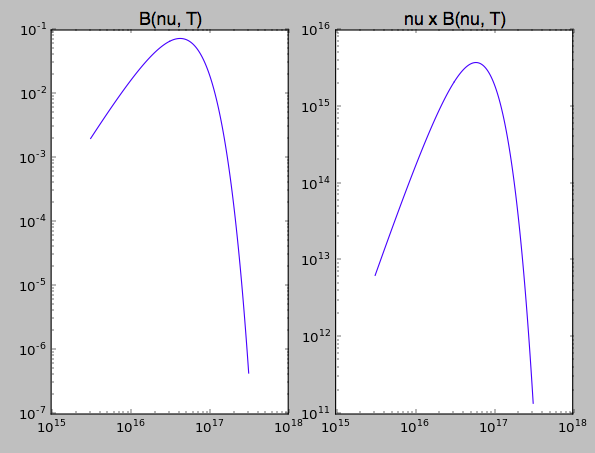
Here is my problem statement:

For a temperature of $7.3 \times 10^5 \ \text{K}$, make a graph of the Plank function (Eq. 3.24), plotting $\log_{10}{\nu} B_\nu(T)$ vs $\log_{10} \nu$ for $\log_{10} \nu$ between 15.5 and 17.5. How does the behaviour of your graph of a blackbody compare with that of Fig. 28.14 for the continuous spectrum of the quasar 3C 273?

Here is the equation 3.24

\begin{equation} B_\nu (T) = \frac{2h \nu^3/c^2}{e^{h\nu/kT}-1}. \end{equation}

I have put together an excel spreadsheet and created a graph of $\log_{10}({\nu} B_\nu(T))$ vs $\log_{10} (\nu)$, however, it is just a straight line (which is what I would have expected although the graph in the book is significantly different). I am wondering if I am misunderstanding the problem statement. What I did to get my data points for $\log_{10}{\nu} B_\nu(T)$ is I first computed $\nu$ values from the given range of $\log_{10} \nu$ values, then I computed B(T) values with the above-given equation. Then I substituted the product into logarithm : $\log_{10}({\nu} B_\nu(T))$, thus obtaining my $y = f(x)$ values. and then I plotted it against $x = \log_{10} \nu$. Am I doing things wrong or is this fine?

Answer 1

Here's what I got for $\nu B_{\nu}(T)$ using Python, which is easy enough to read that maybe you can check against your Excel calculation. I also plotted just $B_{\nu}(T)$ for comparison.

Neither looks like a straight line and they shouldn't. If you print out $\log_{10}(k_B T / c)$ you get about 16.2 which is right in the middle of your range. They've specified a range of frequencies centered roughly on the peak of the Planck distribution.

def Bnu(nu, T):
    top = 2 * h * nu**3 / c**2
    bot = np.exp(h * nu / (kB * T)) - 1.
    return top/bot

import numpy as np
import matplotlib.pyplot as plt

h  = 6.6261E-34 # m^2 kg / s
c  = 2.9979E+08 # m / s
kB = 1.3806E-23 # m^2 kg / s^2 K

T   = 7.3E+05  # K

print "log10(kB T / h): ", np.log10(kB * T / h)

nu  = np.logspace(15.5, 17.5, 201) # Hz

lam = c / nu

print nu.min(), nu.max(), 'Hz'
print 1E+09*lam.min(), 1E+09*lam.max(), 'nm'

B = Bnu(nu, T)

plt.figure()

plt.subplot(1, 2, 1)
plt.title('B(nu, T)', fontsize=16)
plt.plot(nu, B)
plt.yscale('log')
plt.xscale('log')

plt.subplot(1, 2, 2)
plt.title('nu x B(nu, T)', fontsize=16)
plt.plot(nu, nu * B)
plt.yscale('log')
plt.xscale('log')

plt.show()