Power spectrum of matter in the Universe

Question

I have a picture of a power spectrum of matter in the Universe. What is the connection to Lyman alpha? Please describe it for me (horizontal and vertical and shape).

Answer 1

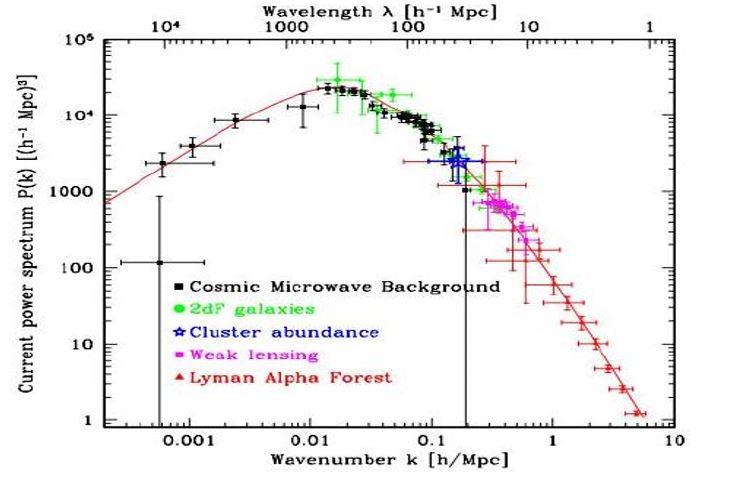
Power spectrum

The distribution of matter in the Universe is not uniform$^\dagger$. Rather, it has clumped together by gravity to form the structure we see, from planets and stars, to galaxies, to groups and clusters of galaxies. Exactly how much the Universe clumps on the various scales can be described statistically by the two-point correlation function $\xi(r)$, which gives the excess probability of finding a clump of matter at a certain distance $r$ from another clump, relative to a random, Poisson-distributed matter.

The Fourier transform of $\xi(\mathbf{r})$ is called the matter power spectrum $P(k)$, where $k = 2\pi/r$ is the wavenumber. The power spectrum thus shows readily how much structure there is on various physical scales, i.e. a high value means much structure. It evolves (increases in amplitude) with time or, correspondingly, redshift $z$, and so may be written $P(k,z)$.

The power spectrum can be written as the product of the primordial power spectrum $P_*(k) \propto k^{n_s-1}$ originating from cosmic inflation, and a transfer function $T(k,z)$ (squared) describing what happens at a later epoch. A good description is given by Peebles (1980).

The figure

The figure you show is taken from Tegmark & Zaldarriaga (2002). It shows the power spectrum as a function of wavenumber, but on the top $y$ axis you see the corresponding spatial scale. This scale is expressed in comoving megaparsecs (Mpc) (where 1 parsec equals 3.26 light-years). This factors out the size of the expanding Universe — or, equivalently, the epoch at which an observation is made — such that observations at different epochs in the history of the Universe can be readily compared. A distance $r$ at redshift $z$ will expand to $r(1+z)$ at redshift $z=0$. Using comoving coordinates, no matter at what value of $z$ we observe $P(k,z)$, we "extrapolate" to the current power spectrum $P(k)$.

Moreover, the distance is divided by the dimensionless Hubble constant, $h \equiv H_0\,/\,100\,\mathrm{km}\,\mathrm{s}^{-1}\,\mathrm{Mpc}^{-1} \simeq 0.7$. The reason for this is that different observations may have made different assumptions about the exact value of $H_0$; in general inferred distances will scale as $H_0$, so measuring distances in $h^{-1}\mathrm{Mpc}$ allows for direct comparison.

In other words, $1 h^{-1}\mathrm{Mpc} \simeq 4.7\times10^6$ light-years at redshift $z=0$, but at redshift, say, $z=4$ — when the Universe was five times smaller in all directions — we had that $1 h^{-1}\mathrm{Mpc} \simeq 9.3\times10^5$ light-years.

Observational probes

The power spectrum depends on various cosmological parameters, mostly on the matter density parameter $\Omega_\mathrm{m}$ and the expension rate $H_0$, but also the baryons ($\Omega_\mathrm{b}$) and dark energy ($\Omega_\Lambda$). Given these cosmological parameters, the transfer function and the resulting matter power spectrum can be calculated. In the plot, the red line shows $P(k)$ as calculated theoretically from linear perturbation theory. For details on the calculations, see Tegmark & Zaldarriaga (2002). It is compared to constraints from various observational probes of the cosmological parameters:

• Black: Anisotropies in the CMB shows how matter clumped in the very early Universe, and its polarization pattern, caused by subsequent scattering, gives constraints on matter distribution in later epochs, on the largest scales.
• Green: Clustering of galaxies (here from the 2dF survey) shows how matter has formed structure on (comoving) scales roughly from a few tens of Mpc (a bit larger than the typical distance between galaxies) to several hundred Mpc (above which the Universe is homogeneous).
• Blue: Abundances of galaxy clusters. Here, the error bars reflect the spread in the literature.
• Magenta: Weak lensing measures photons that have traveled through fluctuations in the metric caused by intervening matter; this technique probes the power spectrum on $\sim10\,\mathrm{Mpc}$ scales. These data are from Hoekstra et al. (2002).
• Red: The smallest scales (largest wavenumbers) are probed by the Lyman $\alpha$ forest (LAF). Whereas all the previous techniques measure light, the LAF is the absence of light, namely absorption lines in a bright background source, caused by the neutral hydrogen in all the diffuse clouds and filaments of gas lying between the source and us. The background source is usually a quasar, but may also be, e.g., a gamma-ray burst. As light blueward of the quasar's own Ly$\alpha$ emission line travels through the Universe, it is gradually redshifted toward the rest wavelength of Ly$\alpha$ (1216 Å), so whenever a parcel of hydrogen gas is present along the line of sight, it will cause an absorption line at the corresponding wavelength, creating a "forest" of absorption lines in the blue part of the quasar's spectrum. These data are (primarily) from Croft et al. (2002).

---

$^\dagger$_{This is fortunate, since otherwise you wouldn't be here to ask the question.}