This image from Wikipedia of the SE Lagrange points and the Hill Spheres suggests that the SEL points are outside the Earth's Hill Sphere. (The Hill spheres are the circular regions surrounding the two large masses.) Is this correct?
But I thought I've read that both the Hill Sphere and L1 & 2 are 1.5 million kilometers from the Earth.
I'm going to answer this in a definition-based manner.
Here is the definition of a Hill Sphere according to Wikipedia:
The Hill sphere or Roche sphere of an astronomical body is the region in which it dominates the attraction of satellites.
Here is the definition of a Lagrange point according to Wikipedia:
In celestial mechanics, the Lagrangian points are the points near two large bodies in orbit where a smaller object will maintain its position relative to the large orbiting bodies.
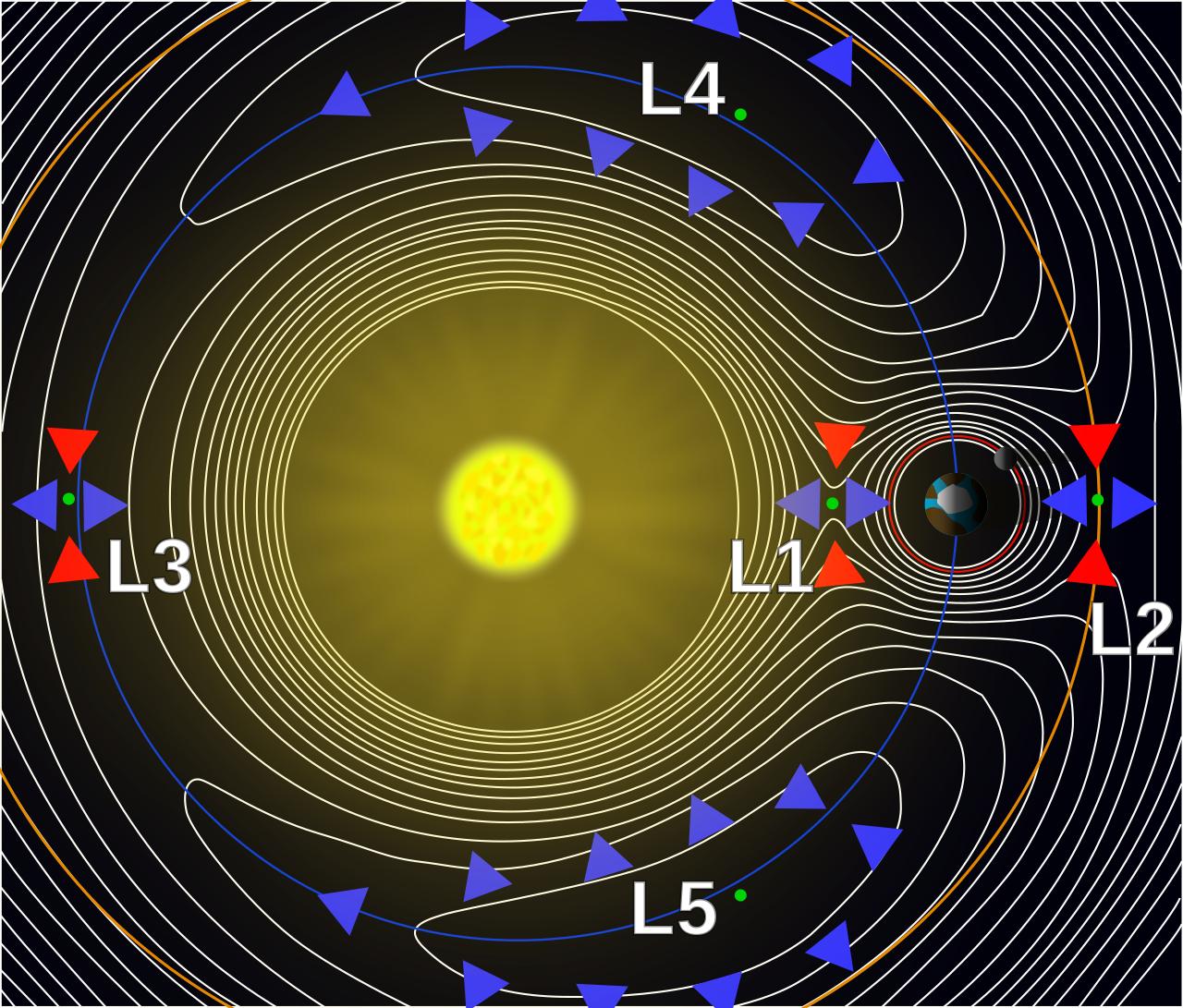
So, if you think about it - L1 and L2 are on the edges of Earth's Hill Sphere - technically, neither inside nor outside, because neither of the two objects dominate gravitational attraction. This is stated on the Wikipedia page for the Hill sphere - and userTLK alluded to this in his comment as well:
In the example to the right, Earth's Hill sphere extends between the Lagrangian points L1 and L2, which lie along the line of centers of the two bodies.
The Lagrange points are where the Sun's and Earth's gravitational influences have no net effect on an object. So, if an object at L1 moved ever so slightly towards the Sun, then the Sun would dominate the attraction of that object, meaning it would be inside the Hill sphere of the Sun. The same goes for the Earth, just in the opposite direction. (The conditions for L2 are different, but detailing them likely isn't relevant to the answer.)
