4
$\begingroup$

Why is the EdS model still taught and used to predict the age of the universe if ours is not an EdS Universe. Does this model have any pros?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
10
$\begingroup$

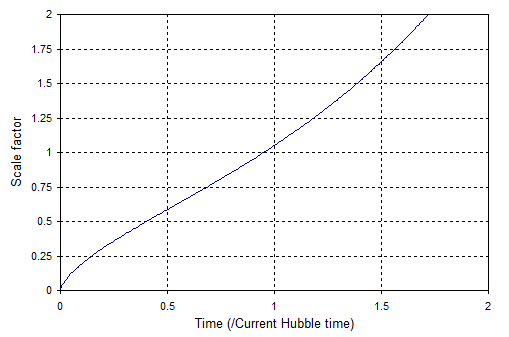
The scale factor of the universe depends on the matter and energy density in a way that depends on which component is dominant. This is discussed in the question How does the Hubble parameter change with the age of the universe? on the Physics Stack Exchange, and from that I have taken this graph showing how the scale factor changes with time for our universe:

Scale factor time

For a (cold) matter dominated universe we get a dependence:

$$ a(t) \propto t^{2/3} $$

and for a dark energy dominated universe we get an exponential dependence of $a(t)$ on $t$. Just by looking at the graph you can see that the $t^{2/3}$ dependence was good up to about half the current age of the universe and after that the exponential growth becomes increasingly dominant.

So you are quite correct that the Einstein de Sitter model is not a good description of our universe right now, but it is a good description of the first half of the lifetime of the universe. Furthermore, because when we view distance regions of the universe we are viewing them as they were in the past it turns out that all the parts of the universe we can see beyond a redshift of about $Z = 2$ are still well described by an Einstein de Sitter model.

I would take issue with your statement that the Einstein de Sitter model is still taught and used to calculate the age of the universe. The most precise calculations of the age of the universe come from the Planck observations of the cosmic microwave background, and these most certainly do not use the Einstein de Sitter model. As for being taught, if you do a general relativity course you will be taught the FLRW metric and Friedmann equations. The Einstein de Sitter model is just a special case of the more general result.

Improve this answer
$\endgroup$
3
$\begingroup$

EdS universe represents a matter-dominated universe. Since the universe evolving towards lambda dominated and recently it was matter dominated, its a good approximation to use the EdS model to calculate the age of the universe.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ The universe has been lambda dominated for several billion years now. $\endgroup$
    – Sir Cumference
    Commented Mar 13, 2020 at 14:08
  • $\begingroup$ @SirCumference well yes but its not like $a(t) \propto e^{Ht}$. I mean that $\endgroup$
    – seVenVo1d
    Commented Mar 13, 2020 at 14:23

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .