How did Kepler come to the conclusion, that exactly the square of the period and the third potency of the great semi-axis of the ellipse is proportional? Why is only square divided by cubic = constant? Why do other dimensions not work? Kepler certainly did not simply try all potencies. Isn't this a similar problem to Fermat's last theorem?
-
1$\begingroup$ Fermat's last theorem is related to (positive) integer solutions of the equation $a^n + b^n = c^n$. Since planetary orbital periods and distances are not constrained to be integers, Fermat's last theorem isn't applicable. $\endgroup$– user24157Commented Jul 10, 2020 at 9:30
-
$\begingroup$ Yes, makes sense thanks! But do you know how Kepler comes to ^2 and ^3 and not any other dimension? $\endgroup$– iwabCommented Jul 10, 2020 at 10:29
-
$\begingroup$ I wonder if answers to How did Kepler “guess” his third law from data? are helpful? $\endgroup$– uhohCommented Jul 10, 2020 at 10:30
1 Answer
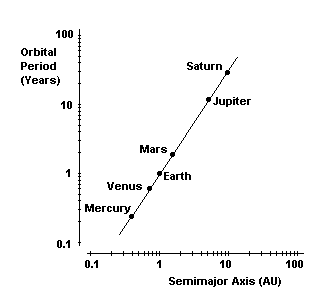
If you plot the log of the period against the log of the semi-major axis then it is obvious that $P^2 \propto a^3$. Any other power law relationship simply wouldn't fit.
The following passage (from https://www.mathpages.com/rr/s8-01/8-01.htm ) seems relevant:
Is it just coincidental that John Napier's "Mirifici Logarithmorum Canonis Descripto" (published in 1614) was first seen by Kepler towards the end of the year 1616? We know that Kepler was immediately enthusiastic about logarithms, which is not surprising, considering the masses of computation involved in preparing the Rudolphine Tables. Indeed, he even wrote a book of his own on the subject in 1621. It's also interesting that Kepler initially described his "Third Law" in terms of a 1.5 ratio of proportions, exactly as it would appear in a log-log plot, rather than in the more familiar terms of squared periods and cubed distances. It seems as if a purely mathematical invention, namely logarithms, whose intent was simply to ease the burden of manual arithmetical computations, may have led directly to the discovery/formulation of an important physical law, i.e., Kepler's third law of planetary motion. (Ironically, Kepler's academic mentor, Michael Maestlin, chided him − perhaps in jest? − for even taking an interest in logarithms, remarking that "it is not seemly for a professor of mathematics to be childishly pleased about any shortening of the calculations".) By the 18th of May, 1618, Kepler had fully grasped the logarithmic pattern in the planetary orbits:
-
$\begingroup$ As shown with modern data, a slope of 1.5 in the log-log plot is clearly the best fit. But I wonder how precisely the numbers (esp. semi major axes) were known at the time, and whether they would exclude, say, slopes of 1.4 or 1.6. If not, then to some extent the question is also about confidence in / preference for simple integer ratios in the power law. $\endgroup$ Commented Jul 10, 2020 at 12:42
-
$\begingroup$ @ELNJ I think they were known very precisely,. That is one of the things that Kepler did. As for a preference for integer ratios that would have been seen as in the "harmonic" nature of things and I doubt more complicated relationships would even have been considered. $\endgroup$– ProfRobCommented Jul 10, 2020 at 12:49
-
$\begingroup$ @ELNJ Kepler was working off of the 20-some years worth of data bequeathed to him by Tycho Brahe. At the time, Kepler had the best set of observational data available to any astronomer up to that point in human history and at the limit of the technological tools of the day. $\endgroup$– zephyrCommented Jul 10, 2020 at 20:29