The Earth's surface which is visible when you look at the planet from a certain distance is a spherical cap in terms of geometry. Here it is, in blue:
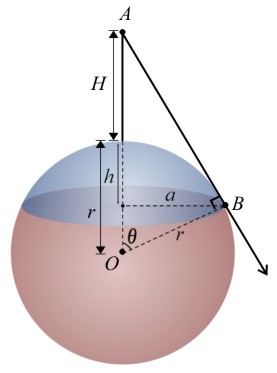
$A$ is the position of the observer,
$H$ is the distance from the observer to the surface of the sphere,
$O$ is the center of the sphere,
$r$ is the radius of the sphere,
$AB$ is the distance to the true horizon,
$\angle ABO = 90°$,
$\angle \theta$ is the angle between the rays from the center of the sphere to the apex of the cap (the pole) and the edge of the disk forming the base of the cap.
The area of the cap $A_c$ can be found according to this formula:
$$A_c=2 \pi r^2(1-\cos \theta)$$
$\cos \theta$ in the right triangle $ABO$ is the ratio of the adjacent cathetus $OB = r$ to the hypotenuse $OA = r+H$, that is, $\cos \theta = \frac{r}{r+H}$, so
$$A_c=2 \pi r^2(1-\frac{r}{r+H})$$
The percentage ratio $R_\%$ of the visible area $A_c$ to the full area of the sphere $A_s$ is $$R_\%=\frac{A_c}{A_s}\times 100\%$$
Since the full area of the sphere is $A_s = 4\pi r^2$, we have:
$$R_\%=\frac{2 \pi r^2(1-\frac{r}{r+H})}{4\pi r^2}\times 100\%$$
$2 \pi r^2$ above and below the line are canceled, so the final formula is like this:
$$\bbox[7px,border:2px solid red]{R_\%=\frac{1}{2} \times (1-\frac{r}{r+H}) \times 100\%}$$
Answering your question, from the altitude of 3,000 mi (4,800 km) one can see $21.4842\% \approx 21.5\%$ of the Earth's surface.
I have typed it in the Google calculator here, so you can use it, just substitute the Earth radius of 6371 km and the altitude 4800 km for the numbers you would like.
Also, here is a graph of how the visible area of the Earth ($r=6371 km$) changes with the altitude, scale and drag the graph with the mouse.
As for finding the altitude from which the given percentage of the Earth's surface is visible, here it is, it is just a transformation my final formula so that $H$ is on one side of the $=$ and all the rest is on the other, only $R$ here is just a ratio, no percent, so you will put 0.38, not 38% into the formula:
$$H=2r/(\frac{1}{R} - 2)$$
The calculator says that to see 38% of the Earth's surface you need to be 20,174.83 km (12,536 mi) above the surface.
