Has GAIA learned anything about General Relativity looking near Jupiter? (Gerry Gilmore: "oblate rotating mass moving in a deeper (Solar) potential")

Question

From Gerry Gilmore (2018) Gaia: 3-dimensional census of the Milky Way Galaxy

4.4 Fundamental physics

Relativistic effects are highly significant for Gaia measurement accuracy, with tests of General Relativity being a significant driver from the very start of the project. This established tight constraints on the mission. For example, sufficient modelling of Newtonian aberration requires that the spacecraft orbit (Lissajous orbit around L2) is quantified with a velocity accuracy of 1 mm/s. Finite light velocity effects lead to position-dependent propagation delays in the field of view which must be accounted for. Monopole light deflection (the famous 1.75arcsec solar limb effect first verified by Eddington & Dyson in 1919) exceed the microarcsec level all-sky for the Sun, and up to 90 deg from Jupiter, significantly complicating the computational effort. Quadrupole light bending is 240µas at the Jupiter limb, and is 1µas at 8 Jupiter radii. This allows a special Gaia experiment – to quantify light bending by Jupiter, this test involving an oblate rotating mass moving in a deeper (Solar) potential.

The Gaia mission (also here):

Another possible experiment is to explore light bending of star images close to the limb of Jupiter to measure the quadrupole moment of the gravitational field of the giant planet.

Question(s):

1. Is looking so close to something as bright as Jupiter and still doing precision astrometry using GAIA possible? Has this been tried? Did it work?
2. Did they see "quadrupole light bending due to an "oblate rotating mass moving in a deeper (Solar) potential"?
3. GR tells us that the light will appear deflected near Jupiter and Jupiter's mass distribution is oblate because it rotates fast, so the deflection will be expected to have a quadrupole moment. But is any of that deflection due directly to the rotation, or would it be about the same for a static, non-rotating but oblate Jupiter? How far down the GR rabbit hole does this go?

Answer 1

Gaia is the follow-up to the Hipparcos mission, which was deactivated in 1993. So Gaia's results are decades in the making. The word "Gaia" was originally an acronym for "Global Astrometric Interferometer for Astrophysics" but as the scope of the science goals of the mission grew greatly, this became antiquated and is now obsolete.

The topic of microlensing with Jupiter has long anticipated Gaia datasets, for example this seminal paper. In Gaia data processing, the bending of light is accounted for with the post-Newtonian approximation, see here. They state: "First, the light propagation from the source to the location of Gaia is modelled in the BCRS in full details required to reach the required numerical accuracy of about 0.1 μas. In this process, the influence of the gravitational field of the solar-system is taken into account. This includes the gravitational light-bending due to the Sun, the major planets and the Moon."

I cannot find any predecessor to Gaia that had the capability to constrain the bending of light around Jupiter. This is a reason why Gaia is so unique: it is the only space-based active mission (as far as I can find, I could be wrong!) that attempts an all-sky survey for high-precision astrometry. Thus, it's (perhaps) the only one that we have currently to probe the bending of light by Jupiter.

Now I'll try to answer your questions directly:

Is looking so close to something as bright as Jupiter and still doing precision astrometry using GAIA possible? Has this been tried? Did it work?

EDIT: Short answers: Yes, it is possible (and increasingly more plausible!); it has not been tried with full rigor to the best of my knowledge; it is strongly expected to work, since microlensing with Jupiter has been successful for discovering, for example, exoplanets.

Indeed, Jupiter is bright, but there are systematic ways of handling this in Gaia photometry. Gaia will observe over 350,000 objects in our solar system. The vast majority are main-belt asteroids, but this includes moons of other planets, implying to accomplish this they would have to account for the brightness of the planets themselves.

This site describes the detectability of stars near Jupiter (relative to Gaia's view), where "the detectability is a function of the star angular position with respect to Jupiter." The image at this site is helpful, 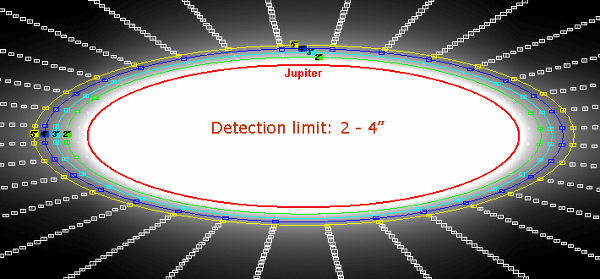which shows the detection limits "for a star of magnitude G = 13.5 as it gets close to Jupiter in a wide range of relative orientations... Jupiter has an elongated shape because the Gaia pixels are rectangular, with a size three times smaller in the x-axis direction. The detection limits found for a G=13.5 star close to Jupiter are between 2" to 4"."

The Gaia SOC calibration team developed calibration tools with GEREQ (which is an acronym for "Gaia Relativistic Experiment on Jupiter's Quadrupole," the device aboard Gaia making these measurements) being the prototypical case.

Did they see "quadrupole light bending due to an "oblate rotating mass moving in a deeper (Solar) potential"?

EDIT: Short answer: No, not yet :)

I've checked both data releases, here and here, and do not see results published on this, but I'm not intimately familiar with it (nor their data archive).

This is an active area of research. For example, this paper presents recent developments in an estimation and calibration framework, where they demonstrate the sub$\mu$as-level stability "of a local reference frame composed of a few tens of comparison stars surrounding the bright target star that is expected to show a large value for the relativistic light deflection due to its proximity to Jupiter’s limb." The authors conclude by stating they plan next to apply the methodology "to actual observations of events in the GAREQ experiment."

GR tells us that the light will appear deflected near Jupiter and Jupiter's mass distribution is oblate because it rotates fast, so the deflection will be expected to have a quadrupole moment. But is any of that deflection due directly to the rotation, or would it be about the same for a static, non-rotating but oblate Jupiter? How far down the GR rabbit hole does this go?

In principle, the extra bending is due to the shape of the lens, so it's directly due to the oblateness. But the oblateness in this case is itself due to the rotation of the planet. So, if Jupiter is non-rotating, then why would it be oblate?

Okay, if this is purely a thought experiment, then the non-sphericity of the lens, which is not rotating, would be similar in principle to galactic light bending, where the lens is some non-spherical galaxy. The non-spherical nature of a lens can complicate the bending of the light, resulting in partial arcs around the lens instead of complete images. How to handle non-sphericity is an open question in general, and different fields have developed various methods to account for it that are usually context specific. In the field of microlensing, this paper shows how it can be handled for close binary star-systems. For how to compute the Einstein angle in microlensing events, see here. Keep in mind that even if there is non-sphericity in the lens, if the lens is aligned with the source then the amplification of the source is maximal.

In the specific case of microlensing with Jupiter and Gaia, the seminal paper also cited above explains quite nicely: starting from the geodesic equation, they arrive at equations 10-12 which give the deflection vector containing the usual monopole term and the relevant quadrupole terms. These are simplified in the case of "near-grazing rays as, unlike with the solar deflection, the effect is too small to be observed at large angle from the planet" into equations 13 and 14, which depend on the orientation of the spin axis of the planet (i.e., the inner products with the spin vector in the $z$ direction). In equation 13, the monopole term is the first term that is independent of $J_2$ (the dimensionless coefficient of the second zonal harmonic), and the terms that subtract from it are quadrupole terms. Equation 14 is due to quadrupole. Clearly the quadrupole terms depend on $z$, implying that this is directly due to the oblateness of Jupiter...

EDIT: .... which the oblateness ITSELF is due to the planet's rotation. In principle, a non-rotating planet would still cause light bending (b/c the Schwarzschild solution does), and a non-spherical mass distribution of the planet could cause extra light bending due to a mass-quadrupole moment of the non-sphericity, but this would be more similar to an effect of an extended body in general relativity, rather than spin, which is what is modeled in the case of Jupiter. So, specifically in the case of Jupiter, one is not wrong in saying that the oblateness directly causes more bending, but it comes with the nuance that the oblateness itself is due to the rotation: this is shown in the equations 13 and 14 above, by the quantity $J_2$ appearing everywhere there is spin. This paper explains this more technically.

BONUS: Jupiter is not alone in this possibility. As stated above, Gaia will see hundreds of thousands of solar system bodies. This means that there are potentially more than thousands of possible microlensing events. Using the DR2 of Gaia, there are about 100 microlensing events predicted already! I've not yet seen improved predictions, since they were anticipating to improve the uncertainties of their predictions using DR3 data, which was delayed due to the pandemic.

DOUBLE BONUS: here's a neat proposal for a new space-based mission to test post-Newtonian gravity in the vicinity of Earth!