What does the Reddening line represents in this U-B vs B-V plot?

Question

The plot is from this paper (pg-8).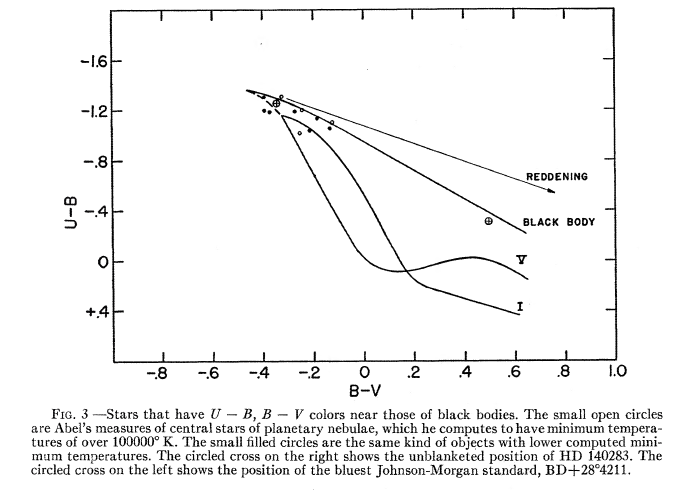

They have also talked(in the same paper) about how central stars from galactic longitude range $20^\circ-80^\circ$ will have a very unusual Reddening line (pg-9). The current plot is for central stars from the galactic longitude range $200^\circ-300^\circ$

The group of planetary nuclei plotted here are all those occurring in galactic longitudes 200o -300°. In the longitude range from 20° to 80° the points fall to the right of the reddening line in Figure 3 (not plotted). If the latter energy-curves are well-behaved, a very unusual reddening line would be needed to explain their position in the diagram. This is a puzzling result which is unexplained at present.

My interpretation of Reddening line: It is showing that if we plot real star data where the sample contains all the stars from bluer end to redder end on this U-B vs B-V plot, we will get the Reddening line on the plot.

Is this interpretation correct?

Answer 1

The reddening line is the locus that a star would move along in a colour-colour diagram as the interstellar reddening towards it increases.

i.e. It is an equation on the colour-colour plot of the form (for this colour-colour plot) $$E(U-B) = f(E(B-V)) + c\ ,$$ where $E(U-B)$ is the colour excess in $U-B$ due to interstellar reddening, $E(B-V)$ is the colour excess in $B-V$ and $c$ is a constant chosen so that the locus would go through a star of the same spectral type but with no interstellar reddening.

Typically, for low reddening and a typical interstellar medium $$ E(U-B) = 0.72 E(B-V) + 0.05 (E(B-V))^2\ ,$$ which I imagine is the line drawn on the plot you show.

To establish the reddening to a particular star you can follow it back from its observed position in the colour-colour plot, along a reddening line, until it intersects the main sequence locus (if it is a main sequence star). The intersection would give its unreddened, intrinsic colours and the x-axis increment through which you traced it back would give its $E(B-V)$.