2
$\begingroup$

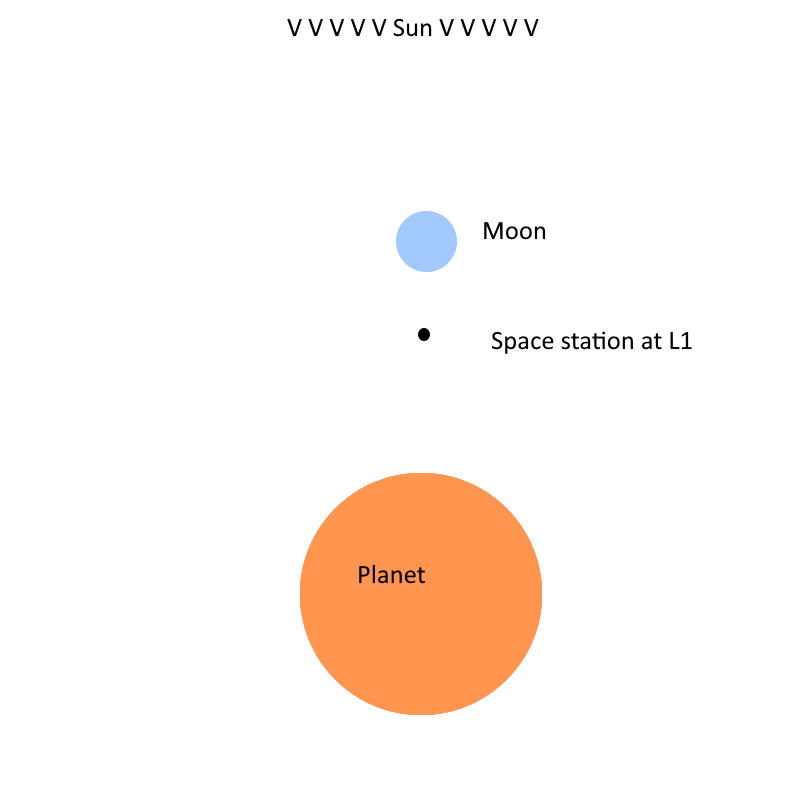
diagram of the situation

The situation I am asking about is as depicted in the picture. Supposing I have a space station staying perpetually at the L1 point, the moon will completely block the space station from all sunlight as it moves between the space station and the sun. Given the distances between all the objects and the sizes of all the objects, how long (percentage of the moon's orbital period is fine) will the space station be in complete darkness?

I think that unlike the situation on the other side of the planet, where the planet is blocking the sun (i.e. a normal lunar eclipse), this situation is complicated by the special positioning of the space station of it always being directly "behind" the moon (due to it being parked on L1).

Here is the numerical data for all the objects in question.

The star:

  • Radius = 585 000 (84% that of the Sun)

The planet:

  • Radius = 87 500 km (125% that of Jupiter)
  • Semi major axis = 1.4 AU = 210 000 000 km

The moon:

  • Radius = 3400 km
  • Semi major axis = 911 000 km

The space station:

  • Located at L1 point, which I have calculated to be about 28 000 km from the moon.

However, just in case I have messed up the calculation, here are the masses of the moon and the planet as well:

  • Mass of planet = 3.8 x 10^27 kg (two Jupiter masses)
  • Mass of moon = 3.4 x 10^23 kg
Improve this question
$\endgroup$
2
  • $\begingroup$ I've written an answer, but note that this kind of "hypotentical orbital mechanics" is rather borderline for "Astronomy". Worldbuilding or perhaps Space Exploration might be better $\endgroup$
    – James K
    Commented Aug 15, 2021 at 14:34
  • $\begingroup$ Ah, I was wondering if there might have been a better place to post this question. Thank you, next time I will post such questions on the space exploration board. $\endgroup$
    – Celibate Hetaerism
    Commented Aug 15, 2021 at 14:52

1 Answer 1

Reset to default
1
$\begingroup$

The orbital period of the moon is $$2\pi \sqrt{\frac{911000000^3}{G\times3.8 × 10^{27}}}=343000\text{s}$$ or 95.3 hours.

I've found, by drawing, that assuming everything lines up (the moon orbits exactly in the plane of the sun, etc) that for 14/360 degrees of the orbit, the satellite will be in the moon's shadow. I carefully drew the orbits and the shadows using GeoGebra I assumed circular orbits, everything coplanar, and sun at infinity (it makes a negligible difference at this scale)

So that gives 3.7 hours.

There is a lot of "reality" that would change this. The satellite would probably actually be in a halo orbit around the L1 position, The moon would probably orbit around the planet's equator, not in the plane of the sun. So reality is more complicated.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Can you elaborate on the process for how you got that the satellite will be in shadow for 14/360 of the orbit? $\endgroup$
    – Celibate Hetaerism
    Commented Aug 15, 2021 at 14:49
  • $\begingroup$ I carefully drew the orbits and the shadows using geogebra. geogebra.org/classic/kb3xfjfj I assumed circular orbits, everyting coplanar, and sun at infinity (it makes negligible difference on this scale) $\endgroup$
    – James K
    Commented Aug 15, 2021 at 14:51
  • $\begingroup$ What do you think about a geometric analysis like this? We can calculate that the angular diameter of the moon as seen from the space station is about 13.9 degrees desmos.com/calculator/28pasavfdo. Then assuming a frame of reference where the station is stationary, we see that it clearly takes p = one orbital period for the moon to return to the same spot in the sky as seen from the station . Thus, the amount of time it takes for the moon to pass over the sun is its angular diameter multiplied by the 1/360 of its orbital period = 14/360 times p. $\endgroup$
    – Celibate Hetaerism
    Commented Aug 15, 2021 at 15:01
  • $\begingroup$ You seem to have an answer, I suspect your 13.9 is the same as my 14 degrees, to a reasonable limit of accuracy. $\endgroup$
    – James K
    Commented Aug 15, 2021 at 15:04

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .