If a spacecraft follows literally the exact orbit of Earth at a different speed to Earth then why doesn't it hit Earth at some point? I'm specifically thinking about this in relation to these spacecraft but I'm also interested in it more generally: STEREO, 2006, https://en.wikipedia.org/wiki/STEREO Helios, 1974, https://en.wikipedia.org/wiki/Helios_(spacecraft) Thanks :)
5
-
5$\begingroup$ This may or may not belong on Space SE. $\endgroup$– WarpPrimeCommented Oct 5, 2021 at 15:59
-
5$\begingroup$ Since the STEREO and Helios satellites are solar observatory missions, I think this question is nominally on topic in Astro SE. $\endgroup$– Connor Garcia ♦Commented Oct 5, 2021 at 16:46
-
$\begingroup$ What Connor said. Also, celestial mechanics questions related to spacecraft are on-topic here if they're also relevant to the orbits of natural bodies. $\endgroup$– PM 2RingCommented Oct 5, 2021 at 16:54
-
$\begingroup$ I don't understand what the example of the Helios sondes has to do with your question. Their orbits are totally different from Earth's orbit, if I understand correctly. $\endgroup$– uUnwYCommented Oct 5, 2021 at 20:37
-
$\begingroup$ According to the linked Wikipedia article, the STERO satellites were not in "the exact orbit of the earth". But rather one was slightly outside and the other slightly inside of the earth's orbit. (I use past tense because one was lost) $\endgroup$– RBarryYoungCommented Oct 7, 2021 at 13:44
Add a comment
|
5 Answers
$\begingroup$
$\endgroup$
11
An orbit is defined by an instantaneous position and velocity. An object in the same position as Earth but with a different velocity does not have the "exact same orbit" as Earth. The STEREO probes were designed to pull ahead or fall behind Earth, so clearly they do not match Earth's velocity exactly. They therefore do not share the exact same orbit as Earth.
Treating Earth's orbit as a perfect circle, it is defined by a radius of 1 AU and an orbital speed of 29.8 km/s. It is not possible to have a circular orbit around the Sun at a radius of 1 AU with a speed anything other than 29.8 km/s. If you accelerate in the direction of travel your orbit will get larger, and if you decelerate, your orbit will become smaller. A spacecraft cannot speed up or slow down and maintain the same orbit like a car changing speed on a circular track. If two objects share the same orbit, they must have the same velocity at the same positions - it's not possible for a satellite to "rear end" another by having the same orbit but different velocities (like two cars on a circular track moving at different speeds). If the velocities are different, so are the orbits (if the cars are moving at different speeds, they cannot be on the same track).
The only way objects with different orbits can collide is if the orbits actually cross one another, and the objects happen to be in the same place at the same time. Given the vastness of space, this is extremely unlikely, and can be actively avoided through proper planning and maneuvering. If two objects really do share the exact same orbit, they must be moving at the same speeds at the same positions, and have an average relative velocity of zero - they will never collide.
-
10$\begingroup$ I like this answer, but I think this part of it is backwards: If you're going faster, the orbit will be larger, and if you're going slower, the orbit will be smaller. $\endgroup$– Connor Garcia ♦Commented Oct 5, 2021 at 16:50
-
16$\begingroup$ @ConnorGarcia You're right, I could have worded that better - I meant that accelerating in the direction of travel will put you into a larger orbit, although the forward acceleration will counterintuitively result in a decrease in average orbital speed. $\endgroup$ Commented Oct 5, 2021 at 18:53
-
2$\begingroup$ I've edited to use something like the wording in that comment. $\endgroup$– James KCommented Oct 5, 2021 at 19:20
-
3$\begingroup$ I think it would be nice if this included a short discussion about Lagrange point L3, which could be interpreted as "following Earth", even though half a year apart in period and position. $\endgroup$ Commented Oct 7, 2021 at 9:52
-
1$\begingroup$ @Stilez I meant that if you know an object's position and velocity at a single point in time, that is sufficient to define the entire orbit of a free-falling object. If two objects have the same velocity at the same position (at any point in their orbits), their entire orbits must be identical. I'm commenting on instantaneous position/velocity, and not trying to imply anything about mean distance/velocity. My point is also only true for velocity, not speed. An instantaneous velocity and position define an orbit. $\endgroup$ Commented Oct 8, 2021 at 12:37
$\begingroup$
$\endgroup$
4
Whats missing in other answers, is that a specific orbit, also requires a specific speed
You cant have just any speed in any orbit. If your objects orbital speed is too high (or low) to maintain its current orbit, then it will drift to an orbit with a (larger/smaller) average distance, where that speed allows it to remain in orbit. The new orbit will have a different eccentricity, as well, seeeing as it evolved out of an inward/outward drift.
So if an object is in earths orbit, it will have the same speed as earth, not be faster or slower.
I want to clarify the difference between this and Nuclear Hoagie's answer, which states that "An orbit is defined by a position and velocity". That answer suggests you get a free choice of both, independent of each other. Thats not actually the case. It's more like, the orbital velocity is a requirement of what it takes, to be in orbit at a given (mean/average) distance.
Once you specify the Sun's mass, and the mean/average orbit distance from the Sun, that sets the speed you have to be orbiting for an orbit to not just become a spiral in or out. Similarly, once you specify the Sun's mass and an orbital speed, that sets the mean/average distance your object will orbit at.
Relevant Wikipedia article on orbital speed
There's 2 cautions on that, however:
Orbits arent usually circular (although often close to it)
Orbits are almost always elliptical. So an object following "exactly" another object's orbit would have to be in the same ellipse, too. Not just same speed and distance. That said, inner planet orbits are usually close enough to elliptical for this to be a minor technicality.
What this does mean, is that there will also be many ellipses (technically an infinite number) that can be orbits at a given orbital speed. So a more thorough answer is that, if you pick any 3 of the 4 out of mean/average orbital speed, eccentricity of the ellipse, size of ellipse, and the mass of the central body (eg our Sun), there will always be a way to choose the 4th that would be a valid orbit.
2 objects in the same/similar/close orbits,or which come close, will affect each others orbits
So 2 objects in the same orbit or close to each other may attract and over time alter orbits and collide (earth/moon formation), modify each others orbits (Uranus/Neptune/Pluto), or prevent planetary formation (?asteroid belt)
Also be aware objects in orbit precess (the ellipse gradually moves around). This was famously used as a test of General Relativity.
-
1$\begingroup$ "... that sets the speed you have to be orbiting for an orbit to not just become a spiral in or out" - going faster or slower than the speed required for a circular orbit at a specific radius doesn't turn the trajectory into a spiral, it just becomes an ellipse (or parabolic/hyperbolic trajectory). Free-fall orbits are never spirals (assuming constant mass). $\endgroup$ Commented Oct 8, 2021 at 13:01
-
$\begingroup$ True, but i wanted to capture the notion it will gradually incrrase/decrease distance to settle in its new elliptical orbit. But yes, not a spiral. $\endgroup$– StilezCommented Oct 8, 2021 at 13:08
-
$\begingroup$ Specifically, the orbit of a two-body system obeys the vis viva equation, $$v^2 = \mu\left(\frac2r - \frac1a\right)$$ where $v$ is the current relative speed of the bodies, $\mu = G(M+m)$ is the sum of their standard gravitational parameters, $r$ is the current distance between them, and $a$ is the semi-major axis of the orbit. See astronomy.stackexchange.com/a/36292/16685 Note that en.wikipedia.org/wiki/Vis-viva_equation ignores the mass of the smaller body, but en.wikipedia.org/wiki/Specific_orbital_energy uses the sum of the masses. $\endgroup$– PM 2RingCommented Oct 8, 2021 at 14:07
-
$\begingroup$ You do "get a free choice of orbit and position." In the Keplerian/Newtonian two-body sense, any combination of position and velocity that's lower than the local escape velocity immediately puts you in an elliptical orbit. $\endgroup$– notovnyCommented Oct 9, 2021 at 9:59
$\begingroup$
$\endgroup$
While other answers are not wrong, what still needs to be mentioned is Lagrange Points.
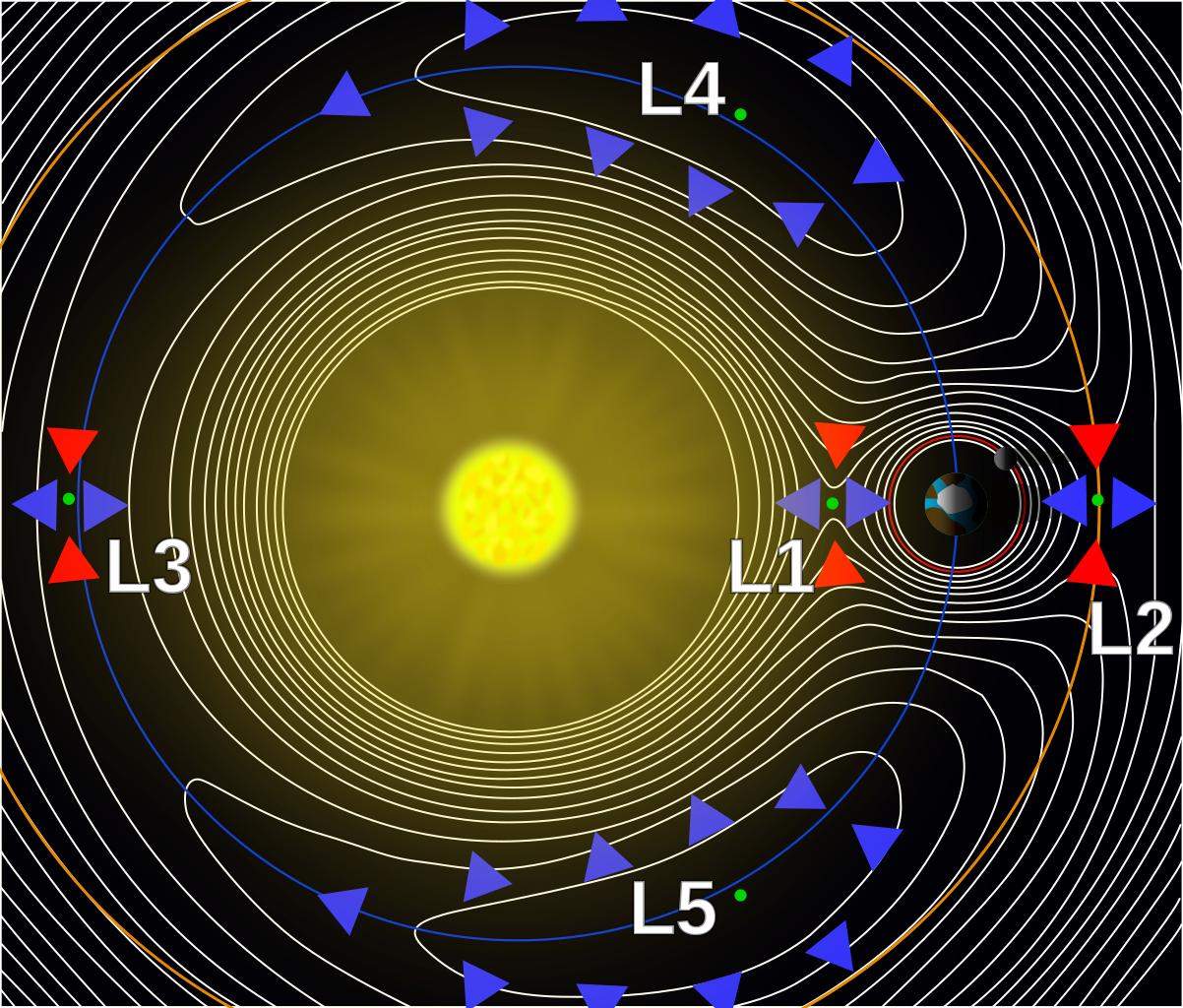These points are invoked when considering the 3 body problem. One example of such system is the Sun-Earth-JWST (L2 point). Another could be Earth-Moon-some Radio Telescope.
The interaction forces from two massive bodies causes a few points in space where these forces form a valley (L4, and L5) or a saddle point (L1, L2, L3). The valleys of larger planets are where we observe collection of space rocks and dust (Jupiter with its Trojans). No energy is required to stay inside this valley. These points act like virtual masses that other objects can orbit. The saddles are semi-stable points where over time, drift would still lead to a divergent orbit, but only small corrections are needed to maintain stable orbit around that Lagrange point.
Therefore, objects placed on L4 and L5 would never hit the Earth, even though they would share the same orbital path.
$\begingroup$
$\endgroup$
3
Theoretically, an object in a solar orbit identical to the earth's is either leading or lagging the earth on the same orbital path. Hence there will be no collision.
In reality, small variations in orbital parameters between the object and earth may cause a collision eventually.
-
2$\begingroup$ It would hit the atmosphere at 11.2 km/s minimum, which would fall under "sufficiently large values of 'fairly gentle'" $\endgroup$– notovnyCommented Oct 6, 2021 at 21:28
-
7$\begingroup$ Orbits are reversible in time - if a collision between the earth and any free-falling impacting body could be "gentle", it would imply that you could do the reverse. That would mean you could launch a rocket "gently", and not require an enormous explosion that accelerates the payload to several km/s. You're not getting off earth without going several km/s, so you can't arrive slower than that. $\endgroup$ Commented Oct 7, 2021 at 13:30
-
$\begingroup$ @NuclearHoagie however, you can launch a projectile on an orbit close to Earth's orbit such that it takes arbitrarily long time to reach you from the other side. $\endgroup$ Commented Oct 8, 2021 at 11:17
$\begingroup$
$\endgroup$
3
There are examples of this in around Jupiter and/or Saturn; I forget the names.
Two bodies in the "same" orbit will attract each other over time, and as they get closer the leading one is slowing down and the trailing one is speeding up, thus, they are now in slightly different orbits. They switch places as the trailing one passes -- the change in speed also causes them to raise/lower so they don't crash into each other.
Then the process begins again.
More generally, with one body much larger than the other, this results in the tiny one moving back and forth along the shared orbit, appearing to an observer on the larger body to be going in a horse-shoe shape.
Thanks to Ramon Snir for finding Co-orbital Configuration in Wikipedia, which describes both of these cases.
-
$\begingroup$ You're referring to the Trojans: en.wikipedia.org/wiki/Trojan_(celestial_body) but STEREO isn't at the Lagrange points. $\endgroup$ Commented Oct 7, 2021 at 16:23
-
1$\begingroup$ No, I'm not. I'm referring to two equal-sized moons that share an orbit, or a tiny body and a large planet where they are not in a stable Lagrange point. $\endgroup$– JDługoszCommented Oct 7, 2021 at 16:34
-
1$\begingroup$ Co-orbital moons? en.wikipedia.org/wiki/Co-orbital_configuration#Co-orbital_moons $\endgroup$ Commented Oct 7, 2021 at 17:52