And:
These are from Wikipedia on Schwarzschild metric, Derivation of the Schwarzschild metric and the last is from Science Direct, Schwarzschild metric.
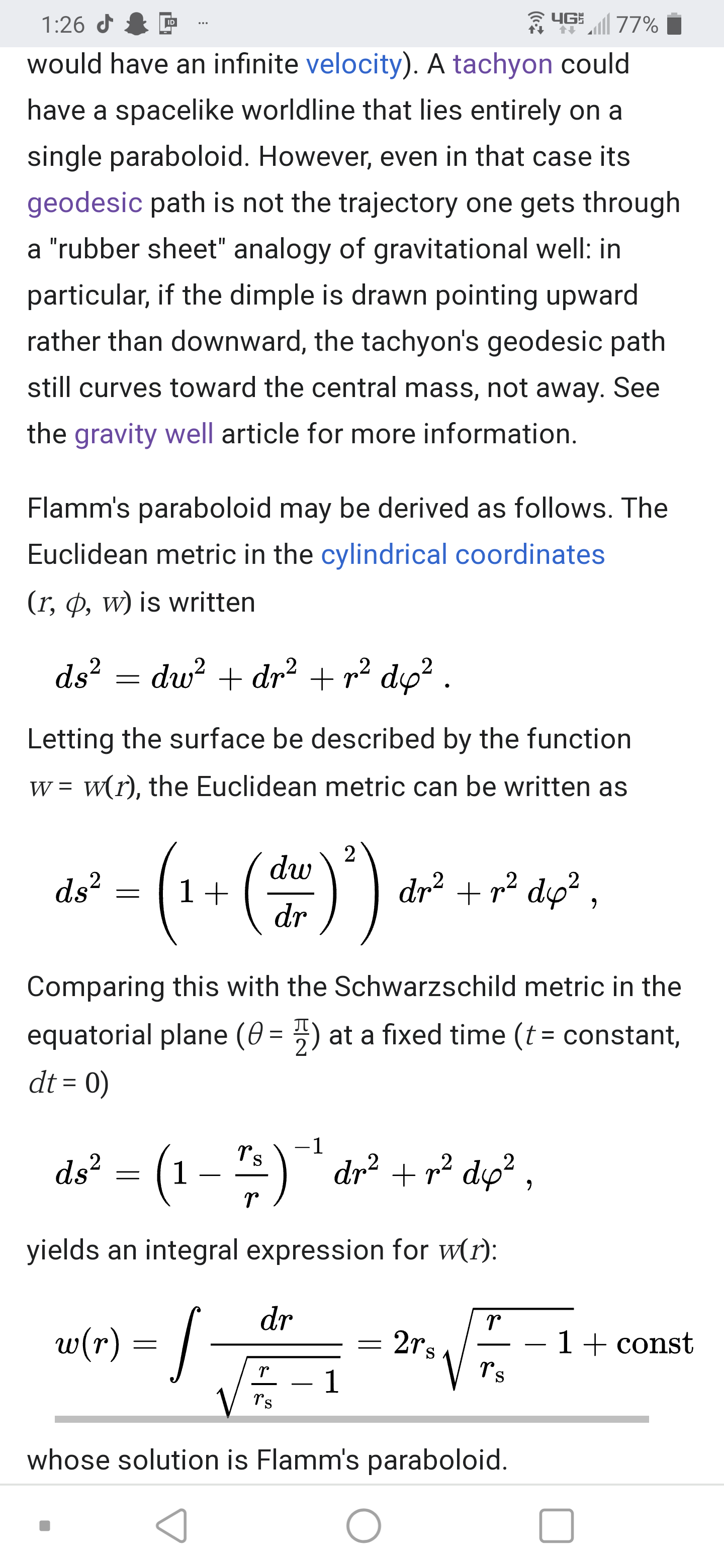
And:
These are from Wikipedia on Schwarzschild metric, Derivation of the Schwarzschild metric and the last is from Science Direct, Schwarzschild metric.

ds refers to a distance in spacetime (this includes time). Just as, in classical physics, you see $$ds^2=dx^2+dy^2+dz^2$$ for any given line element, in spacetime you get similar things going on. For flat spacetime, this gives some good intuitive understanding. Depending on your signature, an element in flat (Minkowski) spacetime is $$ds^2=dt^2-dx^2-dy^2-dz^2$$ or $$ds^2=-dt^2+dx^2+dy^2+dz^2$$ Since the whole point of general relativity is the curvature of spacetime, a line element in curved space will be different. So for the Schwarzschild metric we see what you're seeing in the pictures you provided, except instead of cartesian spatial coordinates, spherical coordinates are used and since the Schwarzschild metric is non-rotating and spherically symmetric, we do not see any angular elements in the metric.