At what point are orbital resonances no longer "ordered" but "chaotic?"

Question

Orbital resonances are typically in small valued integer ratios, like 2:1, 3:2, or 4:7. However, there are some resonances whose ratios have large reduced values, including the 73:69 Naiad:Thalassa resonance. So I am wondering, at what point is a resonance "no longer" one?

By that I mean when the ratios have such large terms (but nearly exact) that it is no longer considered a resonance (for example 581:137), or will this just be reduced to a simpler but less accurate resonance (from 581:137 to 17:4 with an inaccuracy of 0.01)?

Answer 1

Consider a child on a stationary swing. The fastest way to get them going is to push once every time they swing (a 1:1 resonance). If you push 581 times for every 137 swings, the pushes will mostly average out and the child won't get very high on the swing.

Similarly, there are infinitely many orbital resonances possible, but when the integers in the ratios get higher, the effects of the resonance on the orbits are smaller. We don't typically describe orbital resonances as "ordered" or "chaotic," but instead describe them as "stable" or "unstable." A good example of this can be found in the asteroid belt. Orbital resonances with Jupiter that are unstable formed gaps in the belt, and orbital resonances that are stable formed concentrations. Credit Britannica

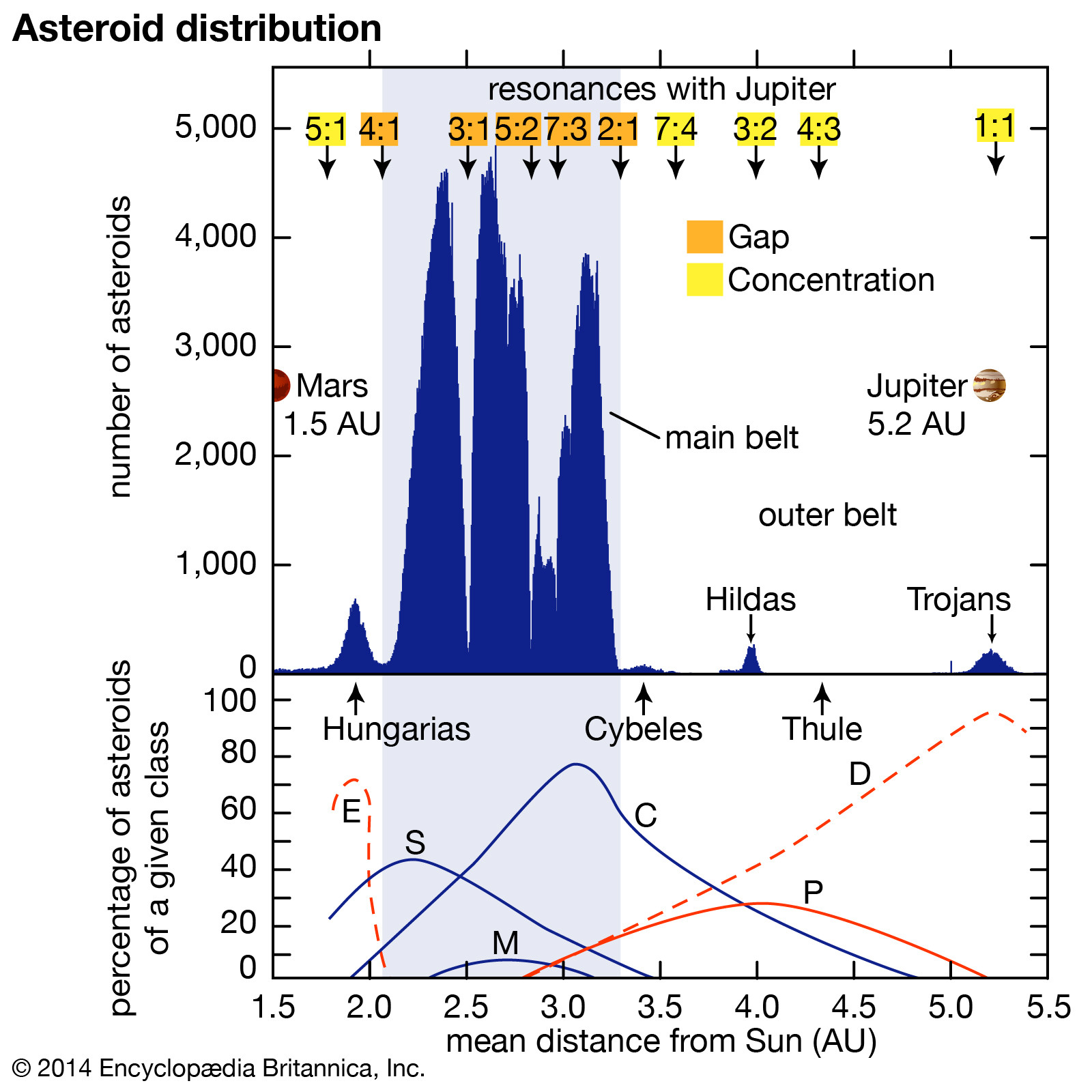
While there are an infinite number of other resonances possible here, practically we only care about the low integer value resonances since they greatly affect the asteroid orbits.

Large integer values for stable orbital resonances (like the 73:69 Naiad:Thalassa resonance) occur increasingly rarely in nature because they have less of a mutual effect on relative orbits than small integer value resonances.

Answer 2

I don't think you are correct. If the resonance can be calculated exactly, then it's a resonance. Chaotic orbits cannot be calculated exactly, as they depend critically on initial conditions.

Perhaps if the calculated fraction had an irrational num or denom, then you could claim "chaos" because the fraction itself can't be evaluated exactly.