No, deuteron production doesn't directly release a photon. Of course, when the positron annihilates with a nearby electron, that creates some gamma photons.
As David Hammen mentions, the proton-neutron conversion is a weak interaction (so it's mediated by virtual W bosons), not an electromagnetic interaction.
Annihilation cannot create a single photon, since that wouldn't conserve momentum. In the centre of mass frame of the electron & positron the total momentum is zero, but a photon must have momentum, so the momenta of the photons produced by annihilation must be balanced. Typically, 2 or 3 photons are produced, depending on the relative spins of the electron & positron (since angular momentum must also be conserved), but larger numbers are possible, although the probabilities are smaller for larger numbers. Please see the Wikipedia article on positronium for further details.
Here's a diagram from the Wikipedia article on the proton-proton chain, showing the main p-p I branch:
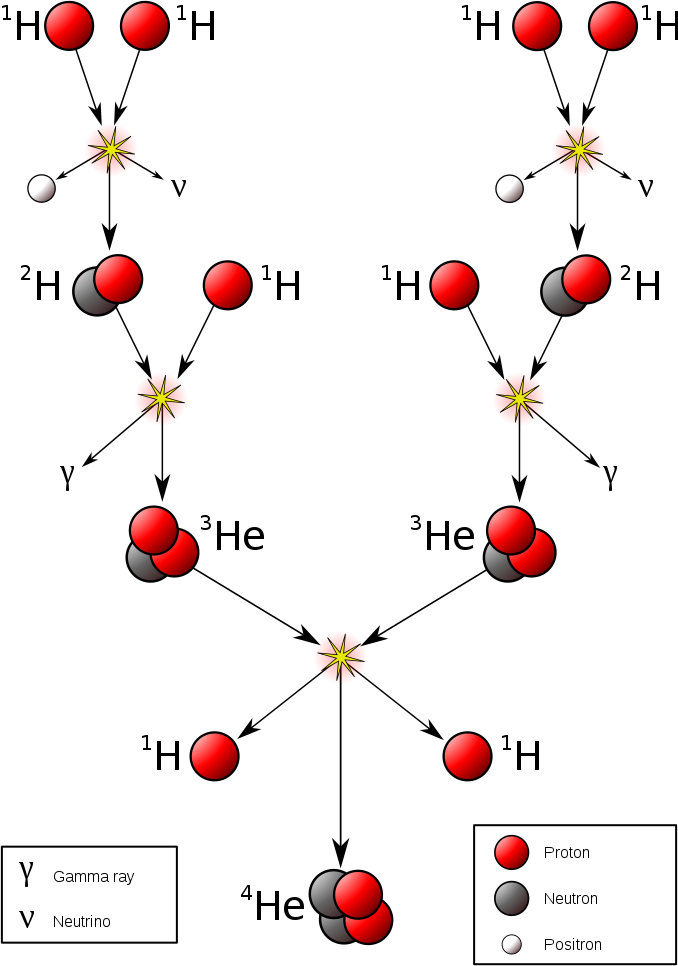
That article gives this reaction (quoted from Iliadis, Christian (2007), Nuclear Physics of Stars) for the deuteron production, including the electron-positron annihilation:
$$\rm p + p + e^- \longrightarrow {}^2_1D + \nu_e + 1.442\,MeV$$
The annihilation releases $\rm 1.022\,MeV$, so the proton-proton fusion itself only produces $\rm 0.42\,MeV$. That energy is released as the kinetic energy of the reaction products, i.e., the deuteron, positron, and neutrino. (Of course, the two protons also provide some KE). Because of momentum conservation, most of the KE goes to the leptons (the positron & neutrino) because the deuteron is almost 1900 times more massive than they are. As is typical in $\beta$ decay events, the energy and momentum are randomly distributed between the leptons. I can't find a diagram for the energy spectrum, but I expect that it is similar in shape to that of neutron decay, which is shown in this Physics.SE answer. Also see this question about the difference between the spectra of $\beta^+$ and $\beta^-$ decay.
The total mean energy released by p-p fusion, averaged over the various branches, is
$\rm 26.73\,MeV$, so the
$\rm 0.84\,MeV$ released in creating the two deuterons is only ~$3.14\%$ of the total.
How often do two protons join up and they BOTH become neutrons? And then decay, putting them back at square one? Every ten octillion × ten octillion collisions, or $10^{56}$?
Yes, that's correct. The probability of both protons converting to neutrons is approximately equal to the square of one of them converting. I don't know the source of your 1 in ten octillion probability, but that sounds reasonable. The usual figure that I've seen for diproton to deuteron conversion is ~$10^{-26}$, which I used in this answer. To put that into perspective, you have to mash around 1 Earth mass of solar core protons to make 1 gram of deuterons, and we can expect 1 pair of those protons to do the double neutron conversion. As I said in the linked answer,
plain hydrogen will never be a practical fuel for an artificial fusion reactor.
I should mention that nuclear reactions involving only the strong force tend to have the highest probability. Reactions that also involve the electromagnetic force (eg, several steps of the CNO cycle) have lower probability, and thus tend to be bottlenecks in stellar nucleosynthesis. Weak reactions have a much smaller probability, and are severe bottlenecks. This is a good thing, because it gives stars a long main sequence lifetime.
