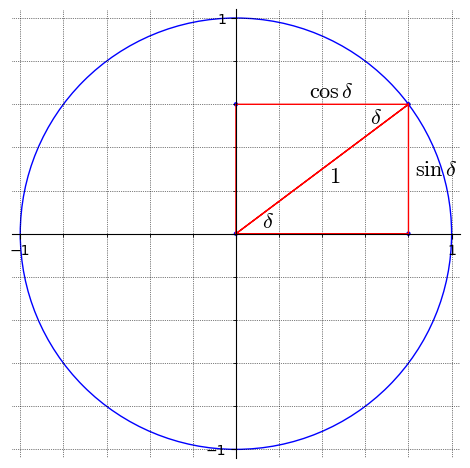
The angular speed of stars in the sky is constant but the apparent angular speed is not, due to the star moving faster on the celestial equator and slower near the celestial pole. The apparent angular speed, modified with $\cos(\delta)$, where $\delta$ is declination. The full equation: $$\frac{0.25^{\circ}}{min}\cos(\delta)$$ How is this equation derived? Resolving the speed on the celestial equator yields the same equation, but the method doesn't seem to be very convincing to me.
2 Answers
As you mention, the angular speed of the celestial sphere on the equator is 0.25° per minute. The speed on a declination circle is proportional to the circumference of that circle, relative to the circumference of the celestial equator.
Of course, the circumference of a circle is proportional to its radius. It's convenient to use a unit sphere for the celestial sphere, so the radius of a declination circle is simply the cosine of the declination angle. This leads us to the speed formula given in the question, $\cos(\delta) × 0.25°$ per minute.
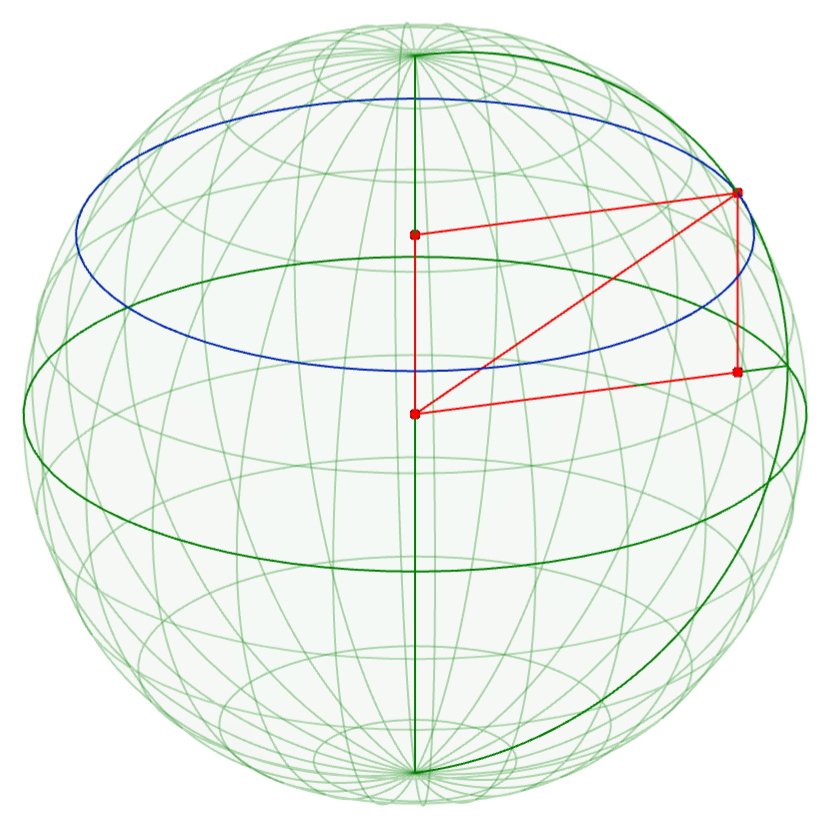
These diagrams may be helpful.
Here's a 3D version:
It's easier to see what's going on in the interactive version.
The Earth rotates approximately $ 15^\circ$ per hour. Or $ .25^\circ $ per minute. So, multiplying $\frac{.25^\circ}{min}$by the cosine of the declination would give you the resulting angular speed in deg/min.
I would like to point out that this equation is off a bit. The $15^\circ$ deg/hour is based on a 24 hour rotational period of the Earth. However, the actual rotation is called a Sidereal day, and is 23 hours, 56 minutes, and ~ 4 seconds. It won't add up to much over the course of a minute or two, but it becomes obvious over the course of several hours, and does trip a lot of people up.