2
$\begingroup$

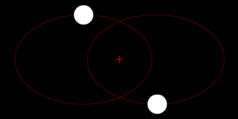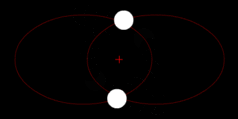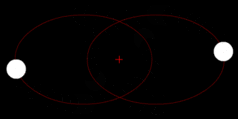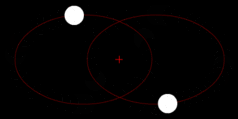
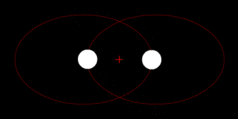
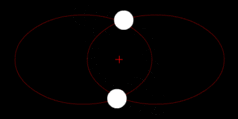
Consider the following image of a barycentric orbit of a binary star system.

Binary Star System

I could draw their relative orbits by drawing a line connecting both bodies at each point in their rotation.

In this system, it is possible that the distances between the two stars are the same when they are opposite to each other both vertically and horizontally in the image. So would that mean that there are three periapside points, and thus three lines of apsides, two of them not going through the barycenter?

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ Kind of need another picture to illustrate what you're asking, but if if I'm reading your question correctly, the situation you describe doesn't happen with Keplerian-Newtonian two-body orbits; both orbits wind up as ellipses of the same eccentricity that share a single line of apsides and have a common focus at the barycenter, whose apoapses and periapses are opposite the barycenter from each other. $\endgroup$
    – notovny
    Commented Oct 4, 2023 at 16:38
  • $\begingroup$ @notovny James K has posted the right images in his answer. I understood how they have the same eccentricity and and that the apoapses and periapses are opposite to one another through the barycenter. However, being elliptical orbits of same eccentricity, can't they intersect in such a way that the horizontal distances are same as the vertical distances - leading to three periapside points (top, middle, bottom) for each body? $\endgroup$
    – Jyothish Kumar
    Commented Oct 5, 2023 at 1:23
  • $\begingroup$ If that happens, either the two orbits you have drawn are not ellipses (the closest point on the boundary of an ellipse to either of its foci is always colinear with them both), or the two ellipses you have drawn do not share a focus at the barycenter. $\endgroup$
    – notovny
    Commented Oct 5, 2023 at 1:40
  • $\begingroup$ @notovny now it makes sense! I forgot that the locus of an ellipse is for which the sum of distances from both foci are a constant! $\endgroup$
    – Jyothish Kumar
    Commented Oct 5, 2023 at 3:09

1 Answer 1

Reset to default
1
$\begingroup$

In the picture, and in any two-body orbit, the stars are closest together at a single periapse, and there is a single line of apsides.

In the picture, the stars are closest together when they are aligned horizontally. They are further away when aligned vertically

enter image description here enter image description here

This will always be the case in any two-body system

Improve this answer
$\endgroup$
3
  • $\begingroup$ Sure, not in this picture, but can you be sure that it will always be the case? Can't two ellipses be brought closer together until the distances match horizontally and vertically? $\endgroup$
    – Jyothish Kumar
    Commented Oct 5, 2023 at 1:17
  • $\begingroup$ No. This is not possible. The proof of this is geometric. But you can also consider the physics: At the periapse, the kinetic energy is at a maximum. As it passes periapse, kinetic energy is converted to gravitational energy so the stars move apart, they'll continue to move apart until kinetic energy is at a minium, at the antaapse. $\endgroup$
    – James K
    Commented Oct 5, 2023 at 1:49
  • $\begingroup$ Makes sense now. I first thought that they come togethor - move apart - come together again - move apart again - come together once more - and move apart -- all in a single revolution. That was when I just sketched it without considering the physics. Thinking of it again makes it seem absurd. Also @notovny's comment on my question cleared up the geometric part for me - it makes more sense now. $\endgroup$
    – Jyothish Kumar
    Commented Oct 5, 2023 at 3:05

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .