Speaking as the author of Robbins (2019 [I always get the date messed up since 2018 was when it was available, but technically the volume it's in is a 2019 year), you are seeing artifacts of how the database was constructed.
All craters in the database were traced manually, by hand. Tiny, imperceptible biases in the exact hand motions are not perceptible for a few craters, but they are noticeable when you have hundreds of thousands of features. I tend to start tracing rims near the 7:00 position and go clockwise, and I'm left-handed, and so there are slight ~1 pixel biases to be elongated along a roughly - but not quite! - N/S direction. For this reason, you can't trust ellipticities smaller than a certain threshold, and you can't trust the ellipticities of craters smaller than a certain size (more on that below).
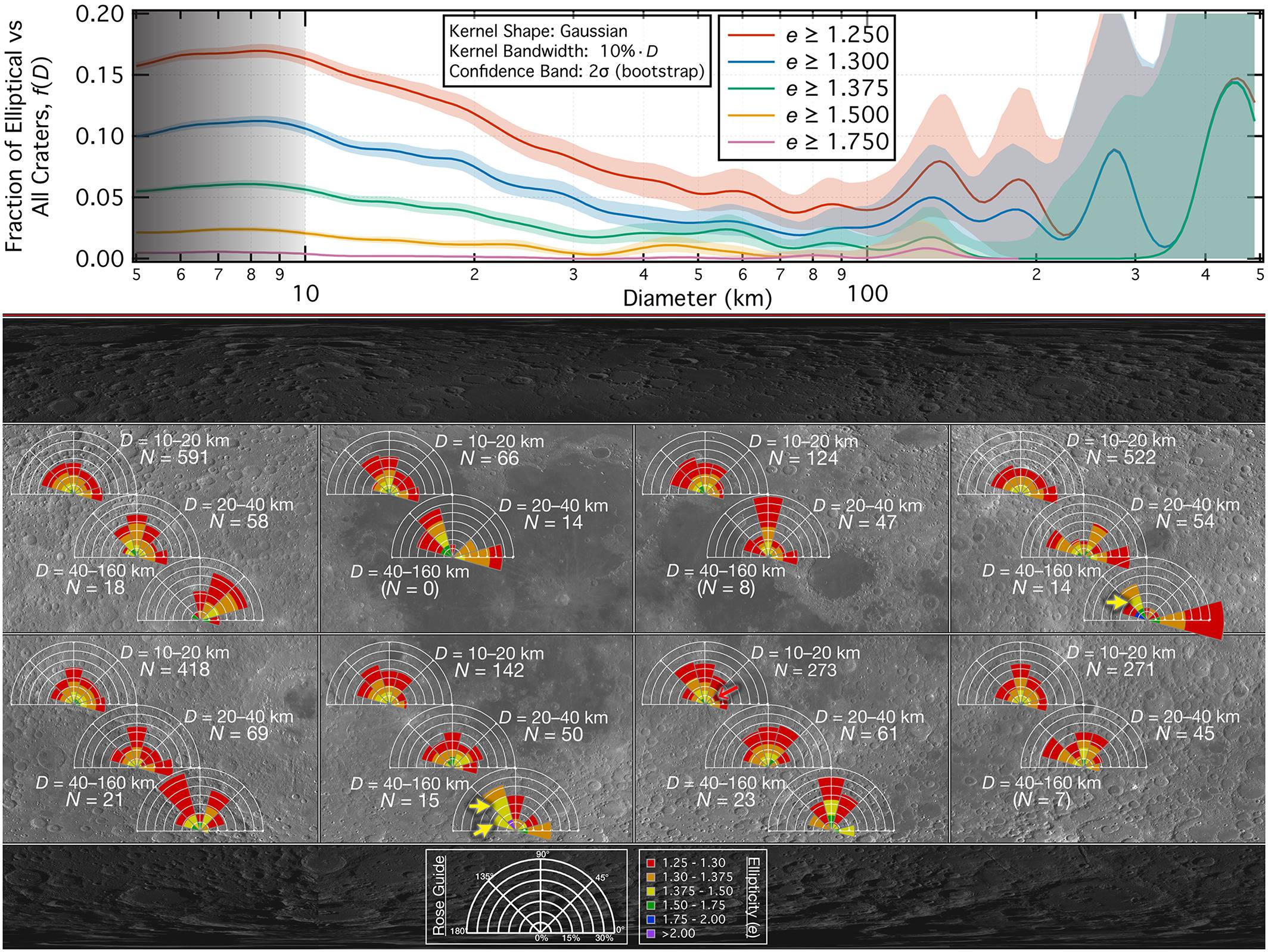In the paper, I did a fairly robust test to determine if there were any trends in ellipticity across the surface as a function of size for ellipticities and crater sizes I trust (more on that next). Figure 6 from the paper (reproduced above) shows the results of those tests, and the gist is that the directionality was independent from randomness. So, no N/S trend.
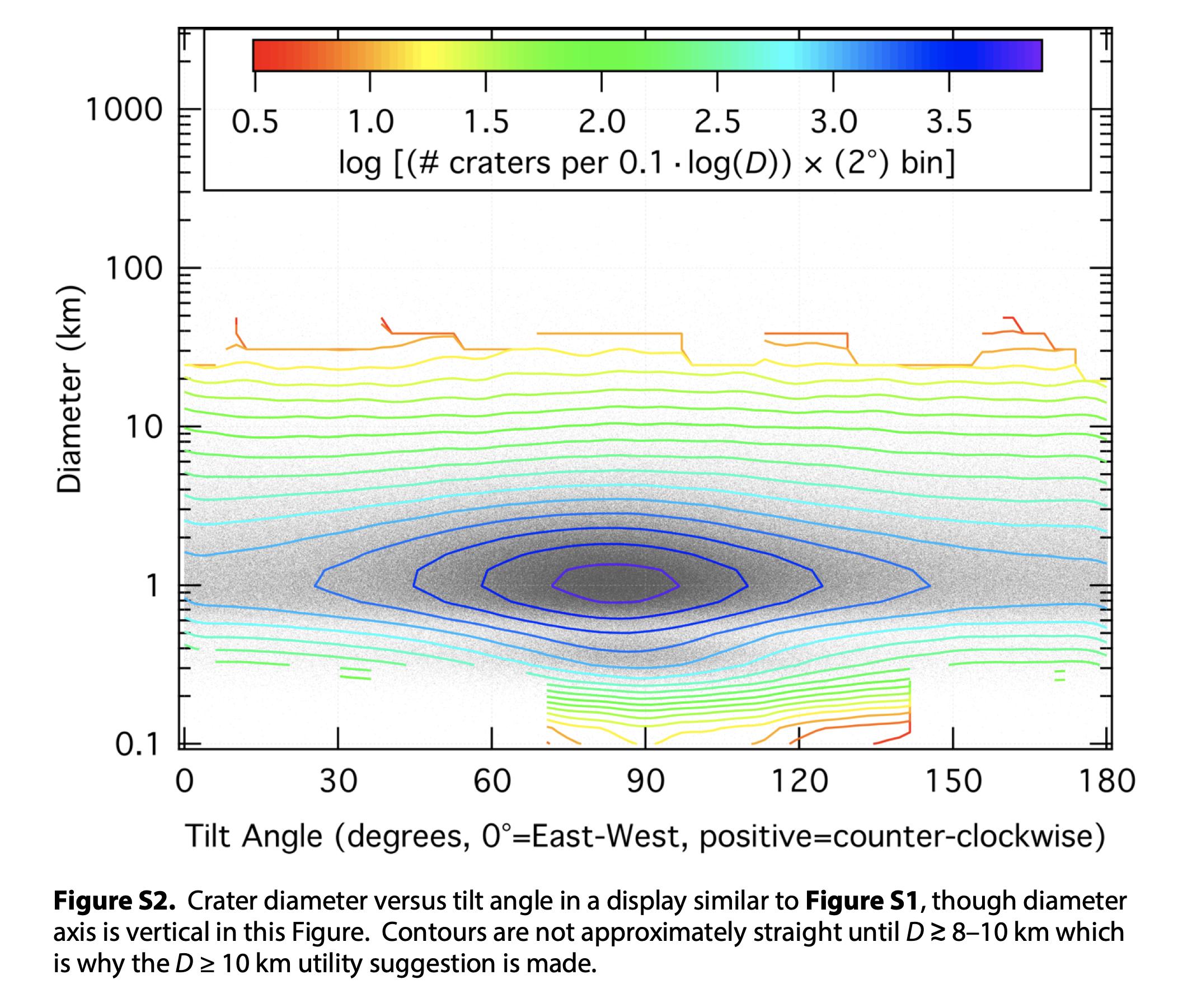Another test I did was relegated to the supplemental material and is the figure S2 copied above. You can ignore the colored contours for the moment and focus on the points. They show what you noticed, that there is a concentration of craters with a tilt angle close to 90°, but not quite. There's the offset due to exactly my biases when tracing rims. They are most noticeable at the smallest diameters -- 1 pixel offset doesn't affect a 100 km crater nearly as much as a 1 km crater. Now, looking at the contours, they start to go flat as you get to diameters of about >~8 km. Therefore, I don't trust most smaller ellipticities for craters <~8 km. Now, if an ellipticity is particularly large, like 1.5 (the major axis is 50% larger than the minor axis), and the crater is ≈1 km average diameter, then that's probably trustworthy. But not an ellipticity of 1.1 or even 1.2 at that size.
Hope that answers your question, let me know if something wasn't clear and I can edit my answer. Given that it's my database, I'm probably the best person to answer questions about it!
