A couple of questions on Hawking radiation

Question

1. Black holes progressively evaporate due to the emission of Hawking radiation just outside their event horizon primarily in the form of photons. However, there is a small probability that they emit massive particles like protons. But can there be some situations or some types of black holes that evaporate only emitting particles with mass (like protons, electrons, cosmic rays...)? Perhaps some kind of a charged black hole that could only emit Hawking radiation in form of charged particles?

2. Also, the cosmological horizon of an accelerating expanding universe would also radiate in some similar process to the Hawking radiation. Is there a non-zero probability that it radiates a particle with mass (like a proton or a cosmic ray) instead of only photons?

Answer 1

warning: Hawking radiation is not fully understood. Most calculations involving it are done in a semi-classical framework, where you combine quantum field theory with a classical black hole. That being said, we do think the broad strokes of Hawking radiation are well established. Any deviations from the semi-classical picture will come from the black hole being treated as a quantum object.

The hawking radiation spectrum is very nearly thermal with a temperature of $$T = \frac{\hbar c^3}{8\pi k_B G M},$$ which scales inversely with the mass. So more massive black holes are colder. All currently known astrophysical black holes have masses greater than $1$ solar mass ($M_\odot$), and that means all known black holes are colder than $6\times 10^{-8}$ K. At the current state of the universe these black holes absorb more CMB radiation ($\sim3$ K) than they emit.

The characteristic thermal energy of a system is given by $k_B T$. If $k_B T$ of the black hole is much less than the rest energy $mc^2$ of a particle there is basically no chance of emitting that particle. If $k_B T$ is much greater than than $mc^2$, then the emitted particle's dynamics will be dominated by their kinetic energy (KE). In this case a zero mass approximation for the particle is good. For example if $k_B T \sim 20$ MeV, nearly no muons will be emitted ($m_\mu = 106$ MeV/$c^2$), and electrons and positrons can be approximated as massless, charged, spin 1/2 particles ($m_e = 0.511$ MeV/$c^2$). There is one complication, that we don't know the exact neutrino masses. At least two are non-zero, and their sum is less than about $0.1$ eV/$c^2$, but we don't know precisely how small they are. The electron is the smallest non-neutrino particle.

$k_B T \approx 5\times 10^{-12}$ eV for an $M_\odot$ black hole. So it's emission will be dominated by massless particles and possibly neutrinos.

To get $k_B T$ equal to an electron's rest energy you'd need a $10^{-17}\,M_\odot$ or $10^{16}$ g black hole.

One of the original papers on Hawking radiation (Page 1976) calculates the emission rate of different massless particles from a Schwarzschild black hole. He works with spin $\frac{1}{2}$, $1$, and $2$: neutrinos, photons, and gravitons. To reiterate, we've since determined that neutrinos are not massless, so the emission rates he calculates are not correct, but the result is still illustrative. This model should be pretty good for $k_B T \approx 1$ keV where we don't get anything heavier than neutrinos and the neutrinos are KE dominated.

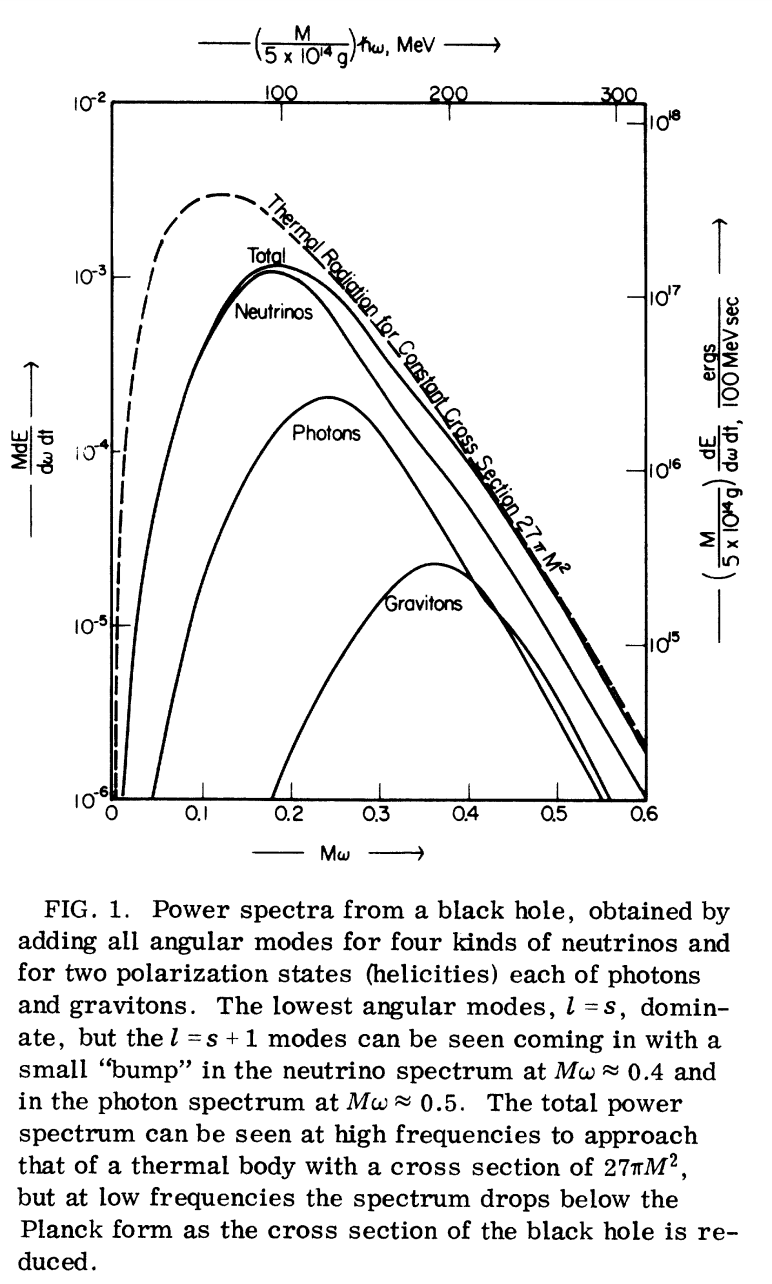(I don't know why he uses four neutrinos, and not the usual three)

Integrating each curve, he concludes that about 81% of the emission is neutrinos, 17% photons and 2% for gravitons. Page also considers black holes with $k_B T$ between the rest energy of electrons and muons. He states without much backing it up that about half of the 81% "neutrinos" would actually be electrons and positrons (i.e. 45%) in that case.

As we go to higher temperature black holes more massive particles can be emitted. In the KE dominated regime fermions (like protons and neutrons) will behave like massless spin 1/2 neutrinos, but bosons (like pions) will behave like photons.

If you want a deeper look, there are two other papers in this series working out emission from spinning black holes (Page 1976b) and the emission of charged leptons from black holes (Page 1977). The later aims to apply the results to massive electrons and muons. It concludes that black holes of the right masses will emit prolific amounts of massive particles, while maintaining the same rate of emission of photons and gravitons. There is also a good deal of more recent literature on the topic.