2
$\begingroup$

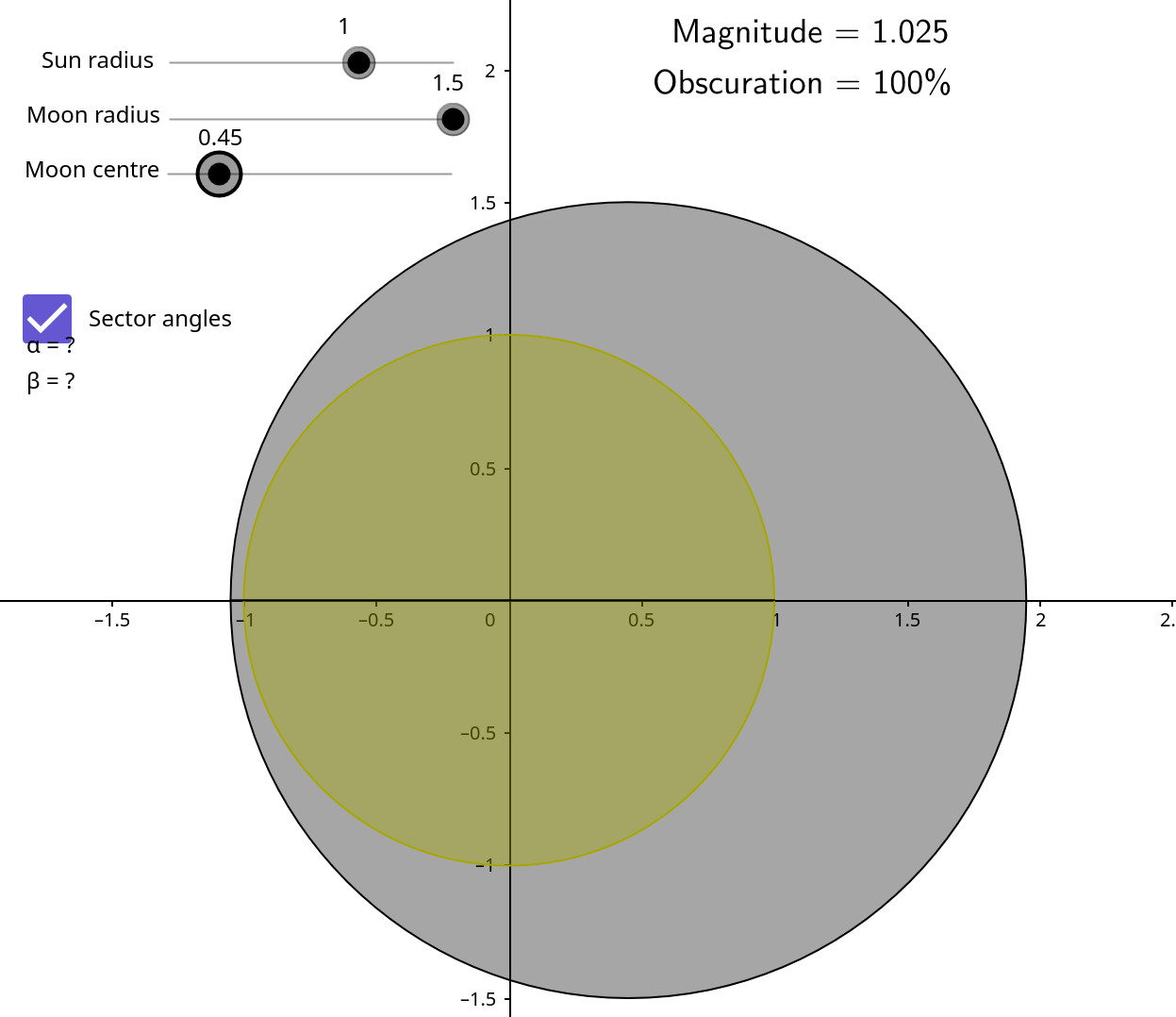
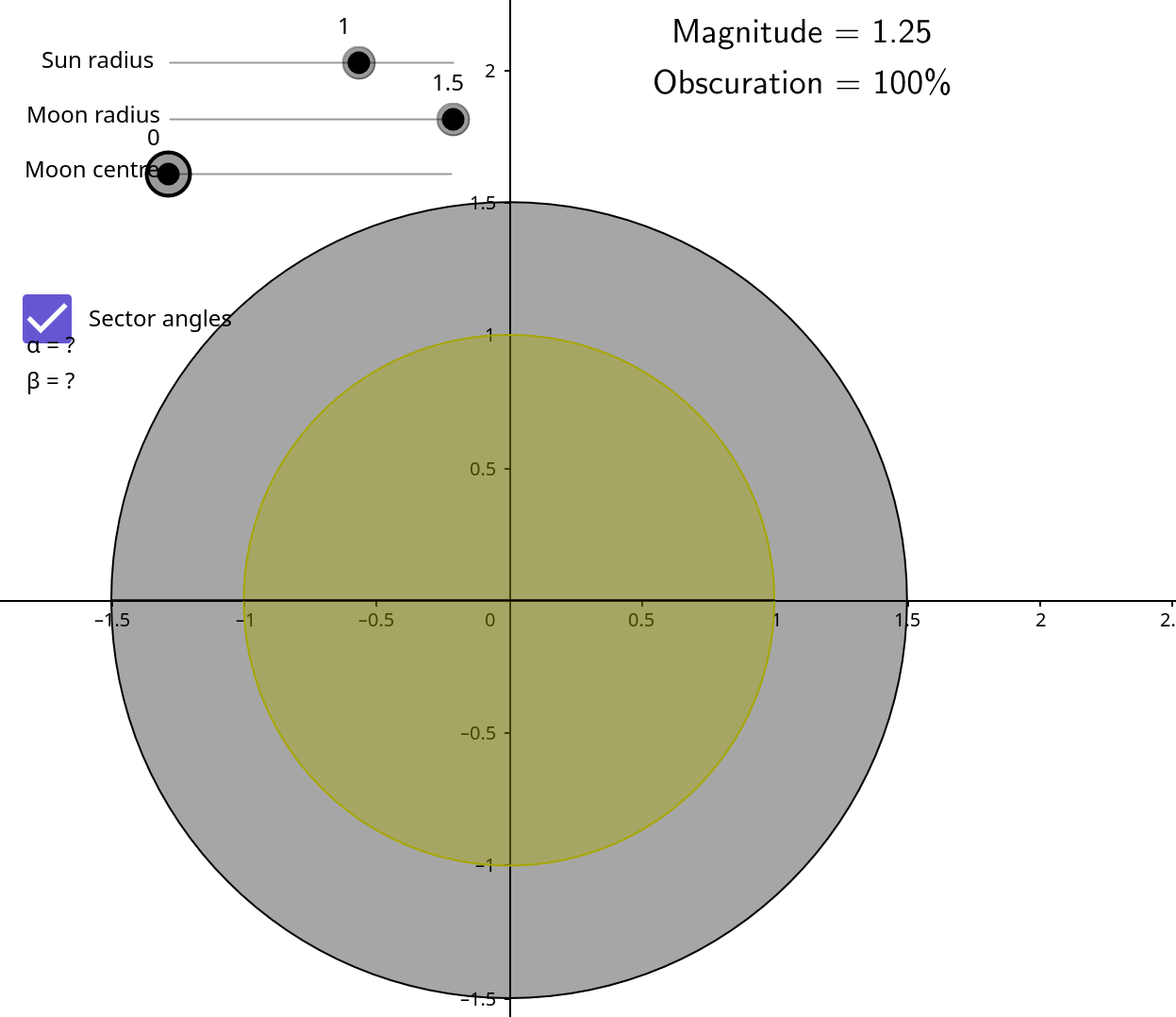
I have found 2 definitions of Eclipse Magnitude in total eclipse:

Solar eclipse for example, below we define $r_1$ as the radius of the Sun, $r_2$ as the radius of the Moon's Shadow, $d$ as the distance of Sun's center and Moon's center

if it is a partial eclipse, the Eclipse Magnitude have no objection:

$$ \epsilon = \frac{r_1 + r_2 - d}{2r_1} $$

Definition 1:

After the Sun is totally blocked, the Eclipse Magnitude jumps from $1$ to $r_2/r_1$, which is the ratio of Moon and Sun's diameter

http://www.jgiesen.de/eclipse/

https://www.oxfordreference.com/display/10.1093/oi/authority.20110803100126148

Definition 2:

After the Sun is totally blocked, the Eclipse Magnitude is still:

$$ \epsilon = \frac{r_1 + r_2 - d}{2r_1} > 1,\quad 0 \leq d \leq r_2 - r_1 $$

only if the Sun and Moon's center overlap, will $d = 0$, and $\epsilon = \epsilon_{max} = \dfrac{r_1 + r_2}{2r_1}$, but this will still be less than $r_2/r_1$

This provide a continuous definition of eclipse magnitude, on the other hand, in an annular solar eclipse, the Eclipse Magnitude is always $r_2/r_1$, having nothing to do with $d$, but this is continuous

https://www.geogebra.org/m/SnZ7QGTJ

Which one is right? I didn't find any books or professional articles defines the calculation of Eclipse Magnitude, Thanks!

The core question I think is: a total eclipse, especially lunar total eclipse, while the earth's umbra is much larger than the moon.

Then the magnitude will be larger if the center of the Moon and the center of the Earth's umbra are closer together See Bottom Figure

(comparing if the moon is close to the edge of the umbra See Top Figure) if using Definition II.

In Definition I, the magnitude will have nothing to do with center distance, as long as the moon is all in the earth's shadow.

enter image description here

enter image description here

Improve this question
$\endgroup$
8
  • 1
    $\begingroup$ Fred Espenak says "the eclipse magnitude is defined as the fraction of the Sun's diameter occulted by the Moon at the geographic location and instant of Greatest Eclipse". eclipsewise.com/solar/SEhelp/SEcatkey.html I think most modern writers would agree with Fred that this ratio of (angular) diameters is the most sensible measure of solar eclipse magnitude. $\endgroup$
    – PM 2Ring
    Commented Nov 5 at 19:05
  • $\begingroup$ I can't quote the equations easily, but the Explanatory Supplement gives equations similar to what PM 2Ring says above. The equations in your question resemble those the ESAA gives for lunar eclipses. $\endgroup$
    – Greg Miller
    Commented Nov 5 at 22:54
  • $\begingroup$ @GregMiller where is the Explanatory Supplement? $\endgroup$
    – Firestar-Reimu
    Commented Nov 6 at 3:28
  • $\begingroup$ @Firestar-Reimu The Explanatory Supplement to the Astronomical Almanac, typically called the Explanatory Supplement for short. It's not a cheap book. $\endgroup$
    – David Hammen
    Commented Nov 6 at 11:50
  • $\begingroup$ The first edition (which has a slightly different title: The Explanatory Supplement to the Astronomical Ephemeris 1961) will probably be the most useful if you're interested in eclipses, as it has worked numerical examples. It can usually be had for cheaper from used sources. $\endgroup$
    – Greg Miller
    Commented Nov 6 at 17:12

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .