I have found 2 definitions of Eclipse Magnitude in total eclipse:

Solar eclipse for example, below we define $r_1$ as the radius of the Sun, $r_2$ as the radius of the Moon's Shadow, $d$ as the distance of Sun's center and Moon's center

if it is a partial eclipse, the Eclipse Magnitude have no objection:

$$ \epsilon = \frac{r_1 + r_2 - d}{2r_1} $$

Definition 1:

After the Sun is totally blocked, the Eclipse Magnitude jumps from $1$ to $r_2/r_1$, which is the ratio of Moon and Sun's diameter

http://www.jgiesen.de/eclipse/

https://www.oxfordreference.com/display/10.1093/oi/authority.20110803100126148

Definition 2:

After the Sun is totally blocked, the Eclipse Magnitude is still:

$$ \epsilon = \frac{r_1 + r_2 - d}{2r_1} > 1,\quad 0 \leq d \leq r_2 - r_1 $$

only if the Sun and Moon's center overlap, will $d = 0$, and $\epsilon = \epsilon_{max} = \dfrac{r_1 + r_2}{2r_1}$, but this will still be less than $r_2/r_1$

This provide a continuous definition of eclipse magnitude, on the other hand, in an annular solar eclipse, the Eclipse Magnitude is always $r_2/r_1$, having nothing to do with $d$, but this is continuous

https://www.geogebra.org/m/SnZ7QGTJ

Which one is right? I didn't find any books or professional articles defines the calculation of Eclipse Magnitude, Thanks!

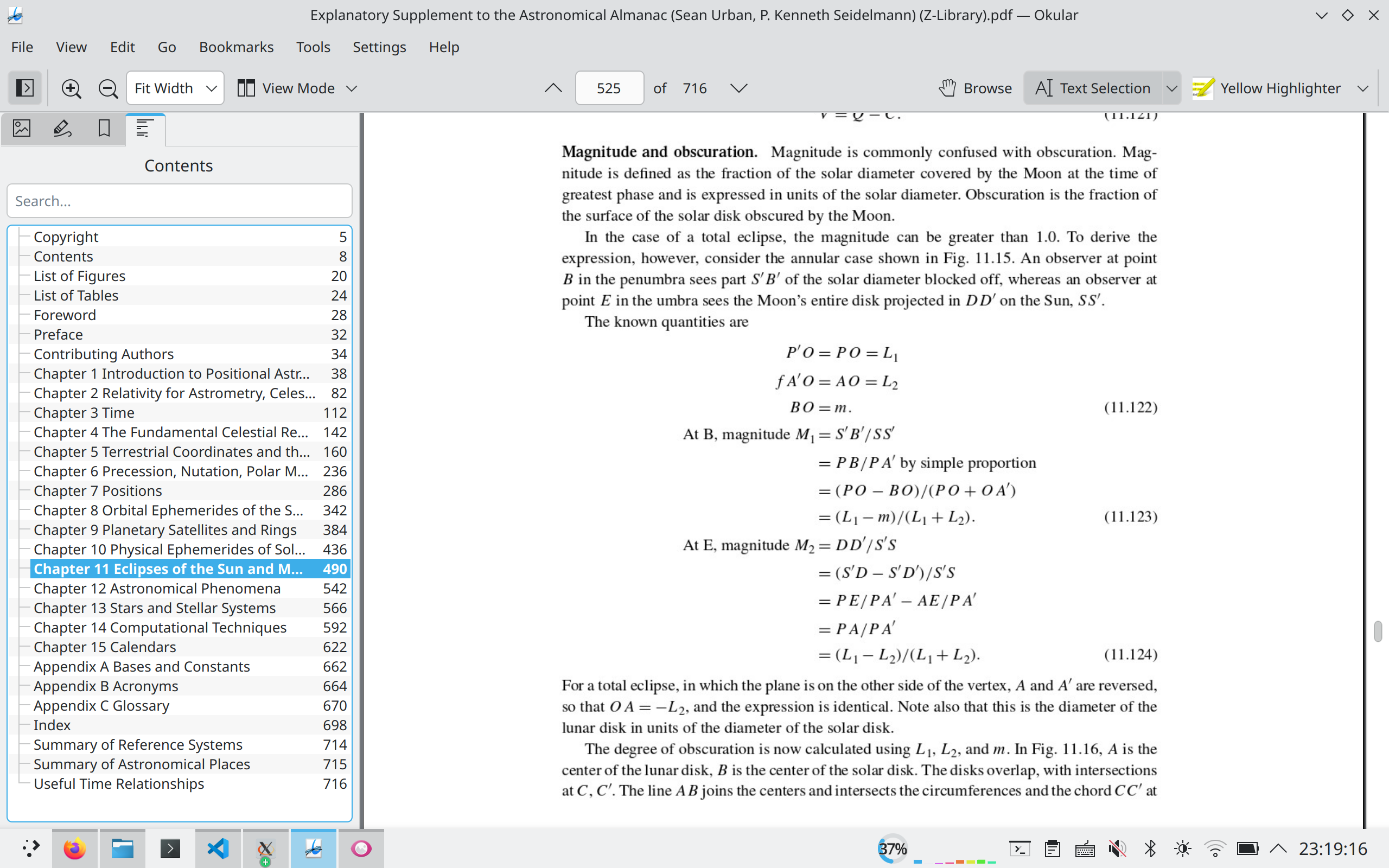
I found this in The Explanatory Supplement to the Astronomical Almanac 3rd edition

but it do not give the total eclipse part that I want

It said ambiguously $OA = -L_2$, but it did not say I should use the in B $(L_1 - m)/(L_1 + L_2)$ or in E $(L_1 - L_2)/(L_1 + L_2)$