0
$\begingroup$

The speed of objects orbiting in a binary system, like Pluto and Charon, is the speed and direction of the objects smooth, or, do the objects make adjustments that are in some ways like a "tug of war" and measurable, showing up as changes in speed or periodic adjustments of direction?

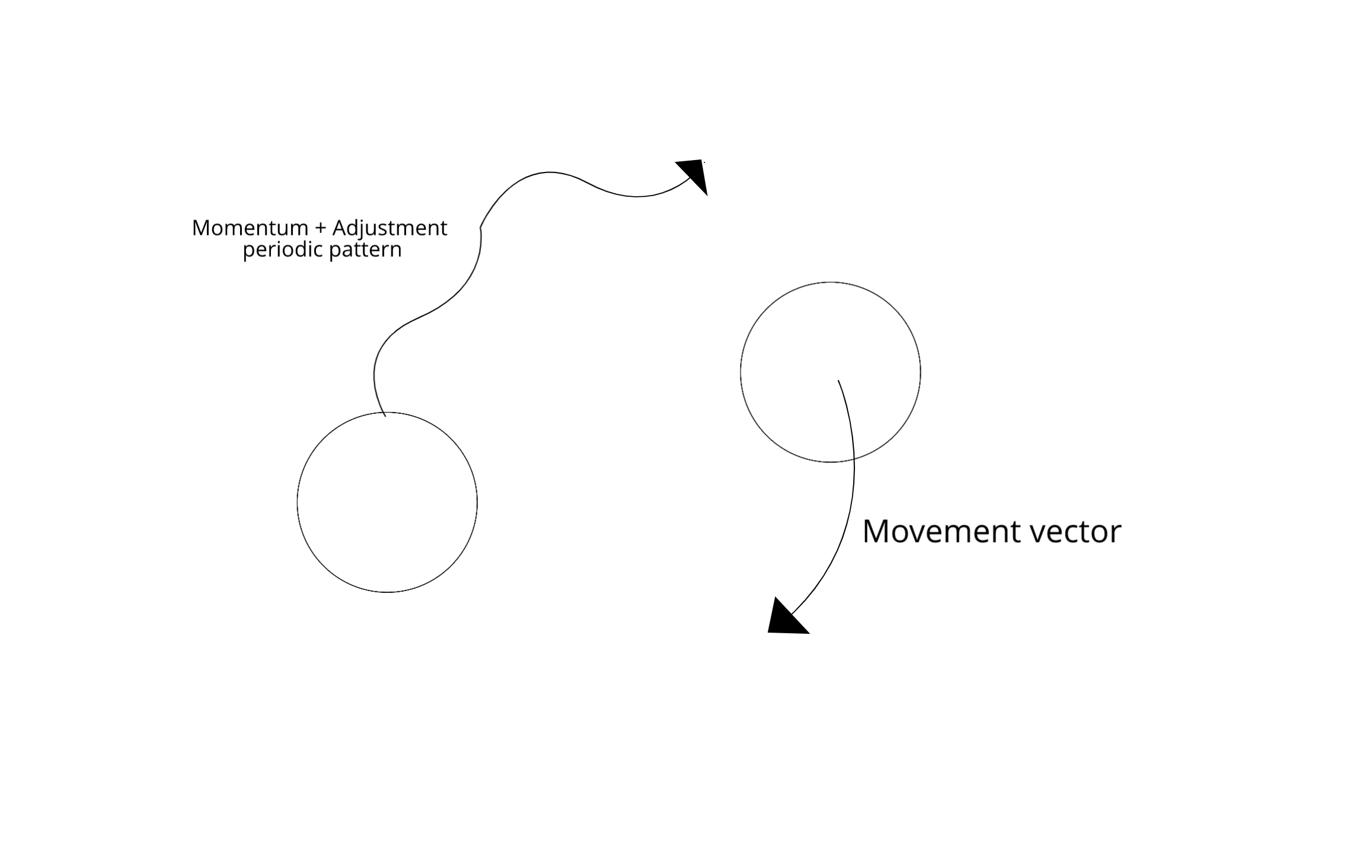
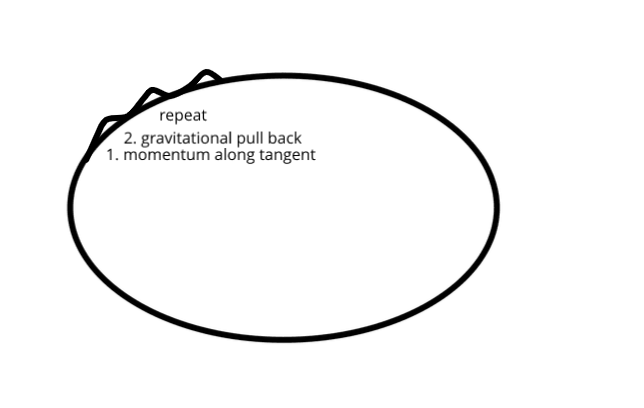
Reasoning: object has momentum, as other objects moves, that momentum remains, while gravitational pull direction changes. Two possible models, either smooth orbit, or, periodic adjustments a bit like a "tug of war".

A poor illustration of the concept of momentum + adjustments showing a periodicity.

enter image description here

Even a smooth and continuous manner down to smallest possible scale must have some wave-like pattern to it. And, based on clockwork harmony, that pattern should repeat itself.

Other drawing, greatly exaggerated,

enter image description here

Improve this question
$\endgroup$
10
  • $\begingroup$ Have you read the Wikipedia article about elliptic orbits? Does it answer your question? $\endgroup$
    – user24157
    Commented Dec 14, 2019 at 15:39
  • $\begingroup$ As far as priorities go, I think this question comes pretty far down the list. So would guess it is pretty hard to find answer to, as models tend to generalize. $\endgroup$
    – Kornelia
    Commented Dec 14, 2019 at 15:42
  • $\begingroup$ It is pretty easy, actually. Terms like "wobble" or "tug of war" or colloquial. Kepler's laws of orbital motion clarify speed differences of objects in elliptical orbits. In this case, the barycentre of charon and Pluto lies between them, but closer to Charon. As they orbit around the barycentre it may look like they "wobble". Wikipedia page on Charon has an animation. Do you mean that ? $\endgroup$
    – user31179
    Commented Dec 14, 2019 at 16:14
  • $\begingroup$ No I mean, literally, as each object has momentum and the other object moves, do they adjust to that in a smooth way, or are there periodic "tugs" that can be measured. $\endgroup$
    – Kornelia
    Commented Dec 14, 2019 at 16:23
  • 1
    $\begingroup$ The answer is no. $\endgroup$
    – ProfRob
    Commented Dec 15, 2019 at 9:32

1 Answer 1

Reset to default
1
$\begingroup$

The speed and direction of objects in a binary system continuously changes over time and are governed by the net gravitational force exerted on them. The closer the objects are the greater the gravitational forces.

In a purely binary system where the gravitational effects of other bodies are insignificant the bodies would normally fall into a regular pattern orbiting each other around the centre of mass. If the orbits are circular the orbital speed will be constant and the direction will change at a regular rate.

As the orbit becomes more elliptical and eccentric the speed of the objects will become increasingly variable with the fastest speed occurring at the closest approach and the slowest at the furthest distance. At the most extreme the planets will approach so closely that they collide.

If one of the objects is significantly larger than the other the smaller one will be accelerated to a greater extent.

Although speed and direction vary they do so in a smooth and continuous manner. There are no sudden jerks or discontinuities.

Improve this answer
$\endgroup$
11
  • $\begingroup$ What about a very very tiny periodicity, but still noticeable? Even a smooth and continuous manner down to smallest possible scale must have some wave-like pattern to it. And, based on clockwork harmony, that pattern should repeat itself. I'm thinking something like hundreds of periods per orbit. Not sudden jerks, but still if looking with enough detail "tugs". $\endgroup$
    – Kornelia
    Commented Dec 14, 2019 at 17:24
  • $\begingroup$ It is not negociable :-) But it can be calculated with arbitrary precision. en.wikipedia.org/wiki/Gravitational_two-body_problem $\endgroup$
    – user31179
    Commented Dec 14, 2019 at 17:44
  • $\begingroup$ Whether or not it is negotiable is irrelevant, what is relevant is what is objectively true. Those calculations deal with generalizations of binary orbits. If there is any periodicity to adjustments in orbits, is not what it addresses. Even a smooth and continuous manner down to smallest possible scale (like that article does but with different focus) must have some wave-like pattern to it. $\endgroup$
    – Kornelia
    Commented Dec 14, 2019 at 17:47
  • 1
    $\begingroup$ Nowhere in natural science appears the term "truth". In contrary, we have verifiability, reproducability, peer review and for the hard core freaks falsifiability. I only hope this is not about a form of geocentrism, the sketch reminds me of ptolemy's attempts to explain opposition loops. As has been stated, nothing jerks in orbits. It is a smooth continuous motion if undisturbed. Perturbations can be induced from outside on a body, but are taken into account when orbits are being calculated, e.g. for times and heights of tides and much more. This is daywork, really. $\endgroup$
    – user31179
    Commented Dec 14, 2019 at 18:49
  • 1
    $\begingroup$ I think we are drifting off topic here $\endgroup$
    – Slarty
    Commented Dec 14, 2019 at 20:42

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .