0
$\begingroup$

According to Wikipedia, Distance measures (cosmology),

Comoving distance: $${\displaystyle d_{C}(z)=d_{H}\int _{0}^{z}{\frac {dz'}{E(z')}}}$$

Light-travel distance: $${\displaystyle d_{T}(z)=d_{H}\int _{0}^{z}{\frac {dz'}{(1+z')E(z')}}}$$

What is with the 'Hubble distance' ($d_H$) before the integration symbol? Do you multiply it by the result of integration?

Also, how do you take the derivative of $z$, $z'$, if $z$ is a constant? $z$ is the (current) redshift for that particular object, right?

I am confused....

From 'Distance measures (cosmology)' on Wikipedia:

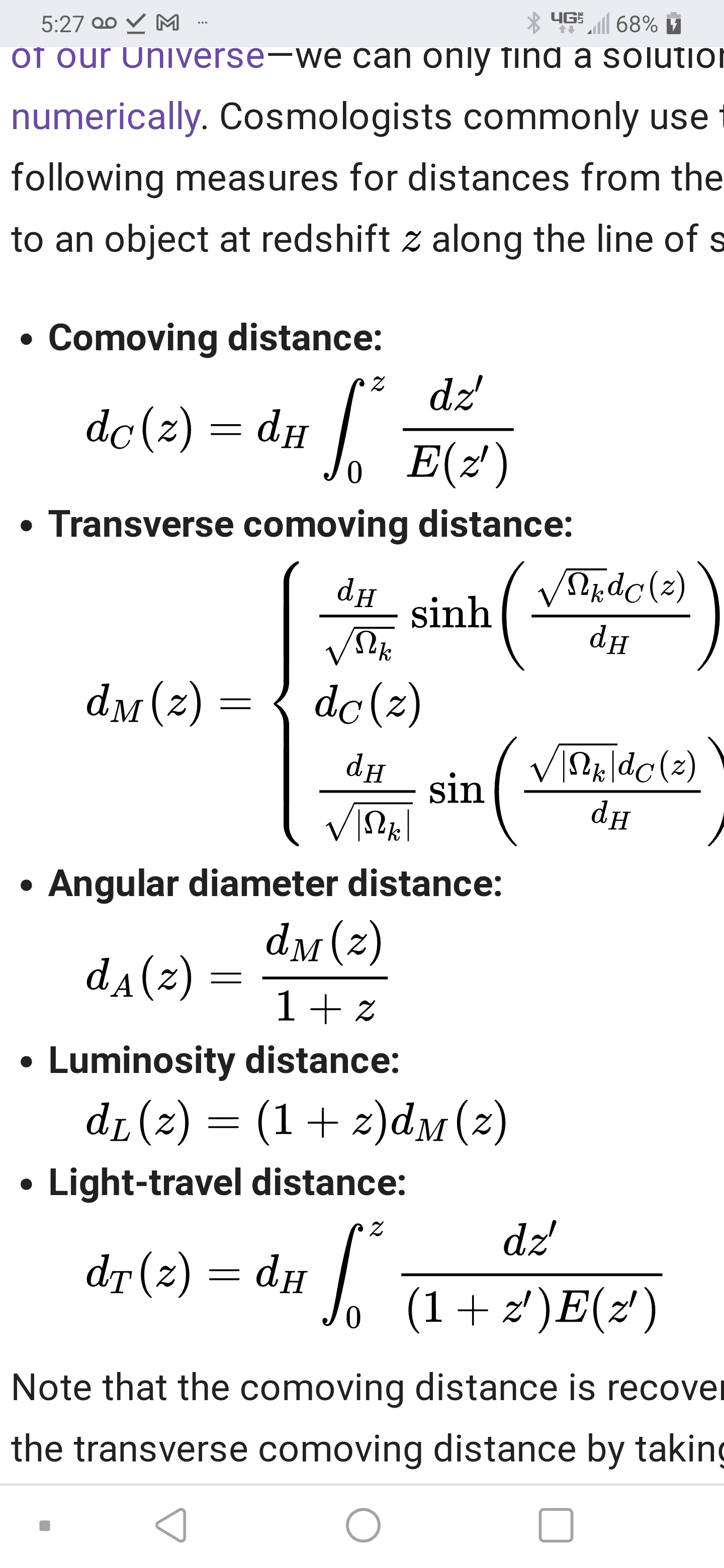
Wikipedia excerpt

Is there an example on the web somewhere of these two things, comoving and travel distance, being calculated? That might help....

Improve this question
$\endgroup$
3
  • $\begingroup$ Thank you for the helpful edit.... I am both stupid and lazy when it comes to LaTeX and MathJax... I am just starting to learn more MathJax, and refresh my LaTeX.... $\endgroup$
    – Kurt Hikes
    Commented Feb 5, 2021 at 0:24
  • 2
    $\begingroup$ In those equations, $z'$ is not the derivative of $z$. $z'$ is the variable that we're doing the integration over, and $z$ is a particular value of that variable. Here's a simple example (using a familiar function) to illustrate this concept. The volume of a sphere of radius $r$ is $V = \frac43\pi r^3$, which we can get by integrating the surface area, $$V = \int_0^r 4\pi r'^2 dr'$$ So $r'$ is the variable radius, and $r$ is a particular radius of a sphere whose volume we want to calculate. $\endgroup$
    – PM 2Ring
    Commented Feb 5, 2021 at 5:38
  • $\begingroup$ Thanks! I just want to find a specific example(s) somewhere, or maybe a 'comoving distance calculator' or 'travel-distance calculator',.... There are several 'redshift to parsecs' calculators on the web.... $\endgroup$
    – Kurt Hikes
    Commented Feb 5, 2021 at 20:27

1 Answer 1

Reset to default
2
$\begingroup$

Yes, you multiply those integrals by the Hubble distance. It's like a cosmological base distance.

You generally can't calculate those integrals by algebra, you have to use a numerical method, like Simpson's rule. The tricky part is choosing a set of $\Omega$ (unitless) density parameters to plug into this equation:

$$E(z) = \sqrt{\Omega_r(1+z)^4 + \Omega_m(1+z)^3 + \Omega_k(1+z)^2 + \Omega_\Lambda}$$

Note that $\Omega_m = \Omega_b + \Omega_c$, where b is baryonic matter and c is cold dark matter. The radiation density term $\Omega_r$ is really the relativistic particle density, since it incorporates the photon density and the (hot) neutrino density. But its value is quite small compared to the other terms, so it's only significant with very large redshift $z$.

Also,

$$\Omega_r + \Omega_m + \Omega_k + \Omega_\Lambda = \Omega_{Total} = \Omega$$

We're pretty sure that $\Omega_{Total} = 1$, and that's why that Wikipedia page on distance measures calculates the curvature term $\Omega_k$ using

$$\Omega_k = 1 - \Omega_r - \Omega_m - \Omega_\Lambda$$

To obtain actual values for those $\Omega$ density parameters, you have two main options, the WMAP data, and the data from the Planck collaboration. Each of those sources provides results derived from several data sets, interpreted according to various cosmological models.

Here's the relevant link for WMAP. Click the "View Matrix" link near the top of the page, which takes you to a page of ~50 sets of parameters.

There's more recent data from the Planck collaboration, but there are various disagreements with the WMAP data. Notably, they disagree on the value of the Hubble distance. Also, you won't find a value for radiation density $\Omega_r$ in the Planck data, but the answers here explain how it can be calculated from other tabulated values. The simplest way is to use

$$\Omega_r = \Omega_m / (z_{eq}+1)$$

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .