Is the closest planet to another planet always the innermost planet?

Question

In our Solar system Mercury is not only the closest planet to Earth on average but also, for the same reason, the closest planet on average to all other planets (Jupiter, Neptune, etc...).

Does that always apply for any planetary system? For instance, in a binary system, is the planet closest to a given circumbinary planet always the innermost planet of one of the two stars? (or can it be the outermost planet, or even another circumbinary planet?)

From Venus is not Earth’s closest neighbor:

Calculations and simulations confirm that on average, Mercury is the nearest planet to Earth—and to every other planet in the solar system.

But this only applies to our solar system's planets, I'm interested in a broader generalization.

Answer 1

For a planet with another planet at their Lagrange L4 or L5 point, those would be each others' closest neighbours; as you can see from the simulations, Mercury has an average distance a few % more than the average distance between the Sun and the planet (e.g. 1.04 AU for Earth), and the Lagrange points form equilateral triangles with the largest planet and the Sun, so for Earth that would be at a (constant) distance of 1 AU.

Of course, whether the smallest of the two would count as a planet is debatable. They could be quite large, e.g. Jupiter (317.8 Earth masses) could have a Trojan weighing 317.8 / 24.96 = 12.7 Earth masses.

Answer 2

As has been mentioned earlier, the fully general version of this question requires rather tricky analysis to deal with orbits that aren't circular and coplanar. It's even trickier if you want to include the gravitational interactions between the planets, although in most cases those small perturbations won't be large enough to affect which planet has the smallest mean distance to a given planet.

Fortunately, for our Solar System, we can use JPL Horizons to find distances between the planets. I'll show some results derived from Horizons for the mean distance between Earth & Mercury and Earth & Venus later in this answer. But first, I'd like to give a brief analysis of the simple coplanar circular orbits version of this problem.

In our simple flat circular solar system, the planets all orbit the sun S in the same direction. The orbit of our "home" planet H has radius 1 and period 1. The planets obey Kepler's laws, so they move around S with constant orbital speed. A planet P with an orbital radius of $r$ has a period of $T=r^{3/2}$.

Relative to H, P has a synodic period of $$T_s = \frac T{|T-1|}$$

Let the angle at HSP be $\theta$. Then $$\theta=\frac{2\pi t}{T_s}$$ where $t$ is time. So to find the mean distance between H & P we need to average over the synodic period.

Let HP be $s$. Then by the cosine rule, $$s^2 = 1 + r^2 - 2r\cos\theta$$ Therefore, the mean distance $m$ between H & P is $$m = \frac1{2\pi}\int_0^{2\pi} \sqrt{1 + r^2 - 2r\cos\theta}\,d\theta$$

That's not an easy integral, but it can be converted to a complete elliptical integral of the second kind.

Using Sage, we can plot $s$ and compute $m$ for a few values of $r$, over two synodic cycles. The blue dotted horizontal line shows the mean distance.

When $r=0$, $m$ has its minimum value of 1, and $m$ grows as $r$ does. For small $r$, the graph of $s$ is almost a sine wave ranging from $1-r$ to $1+r$. But as $r$ grows, we see that the lower part of the graph is decidedly more "pointy" than the upper part, which shifts the mean value upwards. The pointiness reaches a peak at $r=1$, after which it gradually diminishes. (Note that $r=1$ means that H & P are in the same orbit, thus $T_s$ is infinite, so the $r=1$ frame isn't really possible, it's just the limiting case).

You can use this script to generate a static plot for smallish values of $r$.

Here's a plot of $m$ vs $r$, computed using elliptic integrals.

And here's the plotting script

---

As promised, here's a Horizons plot of the Earth-Mercury distance, over 12 synodic cycles. The starting & ending points are close to local maxima. The time interval is divided into 1390 equal steps (which are slightly less than 1 day).

	Julian day number	Date & time
From:	2458159.229166667	A.D. 2018-Feb-09 17:30:00.0000
To:	2459549.125000000	A.D. 2021-Nov-30 15:00:00.0000

Mean distance = 154762296 km

And here's the Earth-Venus distance, over 12 synodic cycles. The time interval is divided into 879 equal steps (slightly less than four days).

	Julian day number	Date & time
From:	2456953.250000000	A.D. 2014-Oct-22 18:00:00.0000
To:	2460466.604166667	A.D. 2024-Jun-05 02:30:00.0000

Mean distance = 170272478 km

Mercury's orbit is rather eccentric, so there's quite a variation in the heights of the maxima & minima, and in the length of the synodic cycle. To get a more accurate value for the mean distance we should really average over a lot more cycles.

The eccentricity of Venus's orbit is quite low, so its graph is much closer to the simple circular orbit plots above.

Although the mean distance of Mercury is certainly smaller than the mean distance of Venus, by 15510182 km, clearly Venus has the lower minimum distance to Earth.

---

Those last two plots were created using this Horizons Sage / Python script. You can use it to create general distance plots. Any body that Horizons knows about can be given as the target, although only major bodies can be given as the center. There's an earlier version of the script here, along with brief instructions.

The script uses the Horizons range data (& its time derivative) to compute a series of cubic Bézier curves passing through the data points. It determines the mean distance by exact integration of the Bézier curves.

Answer 3

Not for double planets, for which each body is always the nearest planet to the other.

Pluto and Charon disputably fit the bill. This 2006 International Astronomical Union report states

Q: Is Pluto a planet?

A: Yes. In fact, Pluto’s large companion named Charon is also large enough and massive enough to satisfy the definition of “planet”. Because Pluto and Charon are gravitationally bound together, they are actually now considered to be a “double planet.”

Even if one doesn't consider them planets, an Earth-Theia-like collision in another system could conceivably produce a pair with a barycenter outside each body.

The wiki also states

...the Moon currently migrates outward from Earth at a rate of approximately 3.8 cm (1.5 in) per year; in a few billion years, the Earth–Moon system's center of mass will lie outside Earth, which would make it a double-planet system.

Answer 4

The reason Mercury is the closest planet to all of the planets on average is in part because of the fact that, because of Kepler's laws, period scales with the semimajor axis of an orbit, and so big orbits are going to be slow and spend lots of time far away from any given object because the orbits are so slow. At opposition, for any two objects, they will be (given a near-circular orbit) the sum of the two semi-major axes away from each other. So naturally, if a planet has a small semimajor axis it's going to move fast around and be a lot closer a lot more of the time than another object with a larger semimajor axis and slower orbit.

This principle is easily expandable to other systems, as Kepler's laws apply to any planetary system. As for the binary system, dynamics close to a binary star system can get pretty complicated so I'm not sure what would happen exactly, but inasmuch as the binary system could be approximated as one mass because of distances, then this would still hold.

Let me know if this explanation is confusing; I can edit and reword this to make it clearer if necessary.

Edit: Upon further consideration and with the help of the comments, one needs to consider what kind of average we're looking at here. In our solar system, a lot of the orbits are near-circular, and in situations like these, circular approximations can yield the possibility of purely spatial averages because of uniform angular velocity. However, as seen by Kepler's laws, orbits are ellipsis, and technically except in the case of a perfectly circular orbit, have varying angular velocities (and by extension tangential velocities). For an orbit with an eccentricity that can't be approximated by a circular orbit, a temporal average must be considered as opposed to just a spatial one.

A good example of this is given by d_e in the comment; he says

Let's imagine 3 planets - one is very close to the Sun. the other 2 are very far (in aphelion) from the Sun and have about the same semimajor axis - and the same direction of the apsides line. Now, the trick is to make them have very very high eccentricity - so the time they spend near the sun is very short; therefore most of the time the planets will spend in their aphelion (which their aphelion, as we said is near to each other)

For circular orbits (or near thereunto) a simple thought experiment can make the reasoning for the mercury conclusion a bit clearer. Let's take mercury, Saturn and Uranus for example. The semi-major axes for each of these is (roughly) .4, 10 and 20 AU (again there's appreciable rounding going on here, but this is a heuristic example). Assuming circular orbits, Saturn will only ever be 9.6 or 10.4 AU away from mercury at all points in its orbit,which means the average would have to fall in there. For Saturn and Uranus, they will be about 10 AU at there closest, and 30 at their farthest. Given the range here, it's not difficult to imagine that the average will likely be a decent amount larger than 10.4, the absolute maximum possible distance average between Saturn and Mercury.

While this example is almost completely qualitative, I hope it's useful for believing the math and quantitative analysis presented by the link posted in the question.

For non-circular orbits, like d_e illustrated quite well, this reasoning does not necessarily extend, and so the answer would be no, this does not extend generally. Each system would have to be analyzed individually. But for a group of near-circular orbits, this seems to be the case.

I have left my previous, faulty answer here as context for the comments below, which are quite illustrative of some important principles.

Answer 5

Not necessarily

The authors of the article linked above assume that the planets involved are

in roughly circular, concentric, and coplanar orbits

Suppose Mercury and Earth had circular co-planar orbits with Mercury at 0.4 AU and Earth at 1 AU from the Sun. With a quick program and Monte Carlo analysis, we get the same result as the above referenced article author's that the average distance from the Earth to Mercury is 1.04 AU.

Alter the above scenario slightly so that Mercury now has a stellar orbital inclination offset from Earth by 90 degrees, i.e. their orbital planes are perpendicular. Now a Monte Carlo analysis reveals an average distance of 1.0584 AU.

So, clearly (notwithstanding orbital instabilities) we could have an innermost planet with an inclination perpendicular to the ecliptic, which on average is further away from Earth than the next planet out with a co-planar inclination.

Add in the possibility for a highly eccentric orbiting innermost planet (where semi-major axis is our measure of stellar proximity) and we can get an even more glaring discrepancy in average distance since the vast amount of time for such a planet is spent far away from the star at apoapsis.

Note: A specific exoplanet counterexample of this kind could be exceedingly difficult to find as:

1. planets tend to evolve in near co-planar orbits.
2. Such a system would almost certainly be unstable.
3. Perpendicular orbital planes would make detection of both planets via transit methods extremely rare.

Code co-planar:

n=1e7;
theta = rand(1,n)*2*pi;
points =[0.4*sin(theta)-1;0.4*cos(theta)];
aveDist = mean(sqrt(points(1,:).^2+points(2,:).^2));

Code perpendicular:

n=1e7;
theta = rand(1,n)*2*pi;
phi = rand(1,n)*2*pi;
points =[0.4*cos(phi).*sin(theta)-1;0.4*sin(phi).*cos(theta);0.4*cos(theta)];
aveDist = mean(sqrt(points(1,:).^2+points(2,:).^2+points(3,:).^2));

Answer 6

[This is complementary answer to Justin Tackett answer. I felt somewhat compelled in light of the comments (even after edits), and to illustrate my example (referenced in the answer) with a diagram.]

I say there are 2 naïve situations where the innermost planet is not the closet. In the attached illustration there are only 2 planets and I consider the distance to the Sun (As we might assume the innermost planet is so close to the Sun - so it won't make any difference to take the distance to the Sun instead).

1. In the first example the period (and therefore the semi-major axis) and the pehilion angle of the outer planets are the same. They differ only in eccentricity. We can see clearly see that the distance between the planets is always shorter than the distance to the Sun. (I used to same orbit phase - but we can allow some small difference - also in the pehilion location)

One can justly claim that this setting is not possible in real-life - and I'll agree with him. The value in my answer is primarily to demonstrate that there is nothing universal here that can be deduced from the Keplerian system; rather, that every system has its own characteristics.

2. The second example that was given in Justin Tackett answer, includes the two planets to have very high eccentricity and about the same semi-major axis and pehilion angle. I admit I thought - in the numbers below given - the effect would be stronger; nevertheless even here it is quite easy to see that the average distance between the planets is shorter than the the distance to the Sun. Note that the diagram is not on scale: the X-axis is longer - had it on scale the orbits would seem much more like strong ellipses. At most of the time the planets are at X>3 while the sun is at 0. this enough to establish I believe my claim here.

Answer 7

The page you link to and the paper refer to PCM (the Point Circle Method), and the PCM treats the orbits of two objects as circular, concentric, and coplanar. If planets in a system had odd (and probably unstable) orbits, this might not be the case.

The PCM method treats the planet's position at any given time as a uniform probabilistic distribution. That means that if you look at a random point in time over the last billion years, the each planet has about the same probability of being at any point in it's orbit. What that gives you is the ability to treat one planet as a point (P), and calculate an average for all points in the other planet's circular orbit as if they are equally likely (C).

In general then for any two planets you look at, the average distance between them will get smaller as the inner planet's orbit gets smaller. If you made a list of the closest planets to any other, they would always be in order of closest distance to the sun (smallest orbit).

In the picture below there is the test planet (outer light blue), and two inner planets that are green and blue where we want to find which one is closest on average.

PCM uses a static position for the test planet (outer light blue) and calculates the average distance to each point on the circles representing the other planets' orbits.

You don't need to do any calculations to tell that the average distance will be smaller for the inner planet though. If we take any angle around the circle and plot a vertical line (on the right), we can see that the outer planet will be further to the side at that point in it's orbit. It will be closer 1/2 the time and further 1/2 the time vertically than the outer planet, but in all cases except for when they're aligned with our test planet, the outer planet will have a larger horizontal component to the distance.

Answer 8

In a binary star system, there are two types of orbits which planets can have.

A planet in an S-type or non circumbinary orbit will orbit one of the two stars. Thus the distance between the two stars must be at least several times as great as the semi-major axis of the planet's orbit, if the planet is to have a stable orbit.

A planet in an p-type or circumbinary orbit will orbit both of the two stars. Thus the semi-major axis of the planet's orbit must be at least a few times as great as the distance between the two stars for the planet to have a stable orbit.

It is possible for a binary star system to have planets in S-type orbits around one of the stars, or both of the stars, and to have planets in P-type orbits around both of the stars.

Each planet around a star will have a forbidden zone around its orbit where no other planet can orbit because of gravitational interactions. The closer two planets are to their star, the smaller their forbidden zones will be, and the closer the two orbits can be.

If a binary system has two stars, A and B, and planets in S-type orbits around each of them, it seems obvious to me that the innermost planet around A will be - on average, as the question asks,-closest to each of the other planets orbiting around A. And similarly the innermost planet orbiting around B will be, on average, as the question asks, closest to each of the other planets orbiting around B.

If the separation between A and B must be at least, for example, at 5 times the distance of the orbit of the outermost planet around either star, we can make a picture of the system.

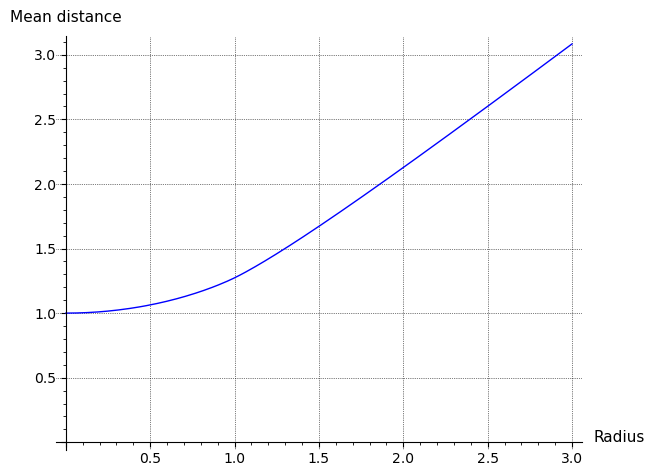
Assume that the outermost planet around each of the stars orbits at 10 units, and the separation of the two stars in their orbits is 50 units. Obviously the separation between the outermost planets of each star will vary between 40 to 60 units, with an average separation of about 50.99 units.

But an inner planet orbiting star A at 5 units, for example, planet A I, will always be between 5 and 15 units from the outermost planet orbiting Star A at 10 units, planet A II, and the average Separation between the two planets will be about 11.180 units according to the Pythagorean theorm.

So any planet orbiting star A will always be closer to any planet orbiting star A than any planet orbiting star B can ever get, even at its closest.

Assume that there are also planets in P-type or circumbinary orbits around the center of mass of the two stars A & B. Imagine that planet AB I orbits at 250 units and planet AB II orbits at 500 Units and planet AB III orbits at 1,000 units.

The distance bween AB I and AB II will always be between 250 and 750 units with an average of 559.01 units.

The distance between AB II and AB III will always be between 500 and 1,500 units with an average of 1118.03 units.

The distance between AB I and AB III Will always be between 750 and 1,250 units, with an average of 1,030.77 units.

So that indicates that the innermost planet orbiting in a P-type orbit around the center of gravity of the two stars A and B, namely planet AB I, will be the closest - on the average, as the question asks - to the other planets orbiting. in P-type orbits around both of the stars.

So that leaves the question of whether any of the planets in S-type orbits around only one of the stars, A or B, will be closer - on the average, as the question asks - to the planets in P-type orbits around the center of mass of the stars A & B.

Assuming that stars A & B have equal mass, they will orbit the center or mass or barycenter, at equal distances. Assuming almost perfectly circular orbits, each star will always be almost exactly 25 units from the barycenter.

I stated that no planet of A or B could have a stable orbit more than 10 units from its star. So no planet of A or B could ever get more 35 units from the barycenter of the two stars.

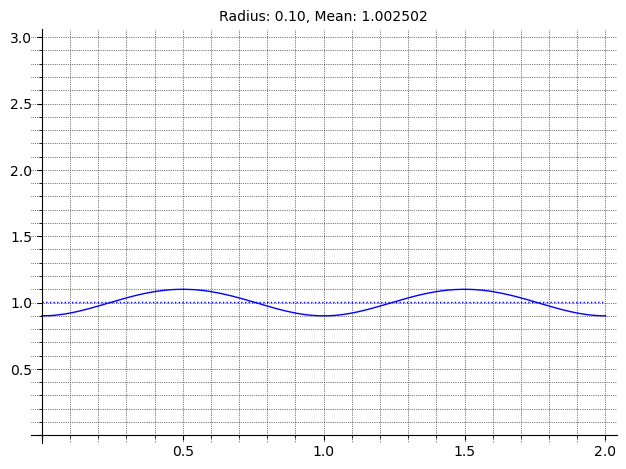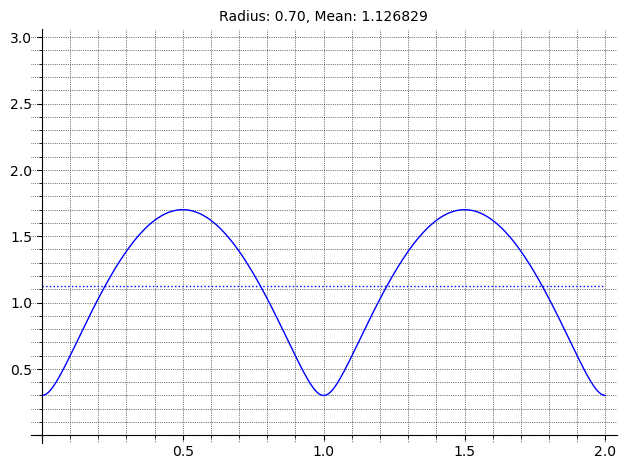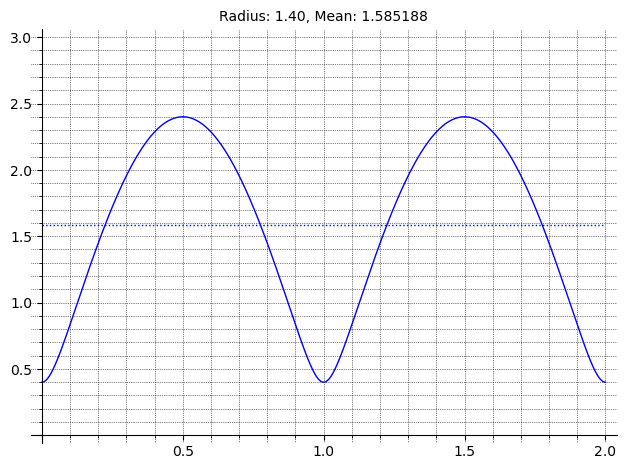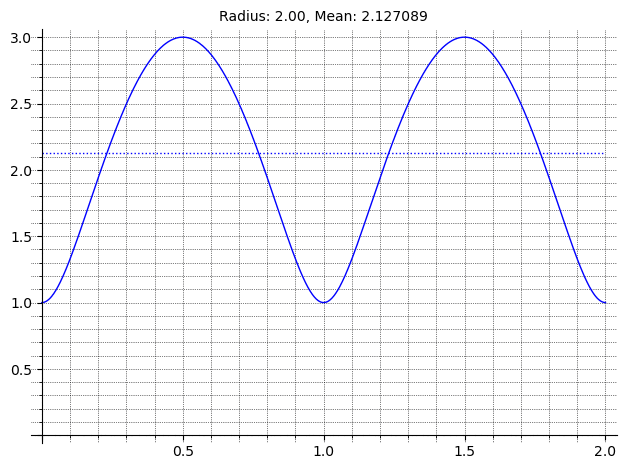
So a planet orbiting A or B could get between 215 and 285 units from planet AB I, with an average distance of about 251 units.

That means that a planet orbiting A or B could sometimes be closer to planet AB I than planet AB II is.

A planet orbiting A or B could get between 465 and 535 units from the planet AB II, with an average distance of about 500.646 units. Which is shorter than the average distance between AB I and AB II.

A planet orbiting A or B could get between 965 and 1,035 units from the planet AB III, with an average distance of about 1,000.3124 units. Which is shorter than the average distance between AB II and AB III and shorter than the averaage distance between AB I and AB III.

So apparently in a binary star system with planets in S-type orbits around one or each of the stars, as well as planets in P-type orbits around the center of mass of both the stars, the planets in S-type orbits will be closer - on the average, as defined in the question - to the planets in P-type orbits than any of the planets in P-type orbits will be - on the average, as defined in the question - to each other.

Of course I have only demonstrated that for the specific orbits I have chosen for the system in my example.

Answer 9

Lets take a more general look at the situation in systems with one star:

What is the average distance to a planet? Break this down into two components, the distance along a line from the planet you are measuring from (which I will call O), through the barycenter of the planetary system which I will call X, and the distance the planet is offset from this line which I will call Y.

Now, what is the average X? While the planet will spend more time near it's apoapsis than it's periapsis this is independent of the orbit of the planet you are measuring from and thus will average to zero. Thus the average X is always the distance from O to the barycenter. This is the same for all planets.

This leaves the Y. Once again, the shifting angles between O and the target average things out--it's going to vary from zero to the average orbital radius of the target. My calculus is far too rusty to sum this but it doesn't matter--it's clearly dependent on the orbital radius and nothing else.

This we have X fixed and Y varying at the orbital radius. Thus sqrt(x^2 + y^2) will likewise be ordered by orbital radius although the relationship is no longer linear. Thus the average distance to a planet will be ordered by the orbital radius of the planet. The inner planet is always the closest.

Now, there are some special cases that have been presented in other answers: double planets, L4 & L5 objects. However, one of the requirements to be a planet is to have cleared one's orbit--and all such cases mean you have two objects in the orbit. Thus they can't be planets to be a closer planet.

Answer 10

A modification to this question that might clarify it:

Is the average distance between a planet in a standard orbit (one planet per circular-ish orbit) and its primary, always less than the average distance between the planet and any other planet orbiting the primary?

The nearer a planet is to the primary, the nearer its average distance to any other planet is to the average distance between the primary to any other planet.