Meaning of $\cos H$ in the rising and setting formula

Question

In the rising and setting formula$$\cos H=-\frac{\left(\sin\nu+\sin\phi\sin\delta\right)}{\cos\phi\cos\delta},$$ $H$ is hour angle, $\nu$ is vertical shift, $\delta$ is declination and $\phi$ is the observer's latitude. If $\cos H>1$,the object never rises; if $\cos H<-1$, the object never sets. My question is why does $H$ not having a solution in the above formula imply an object either never rises or never sets?

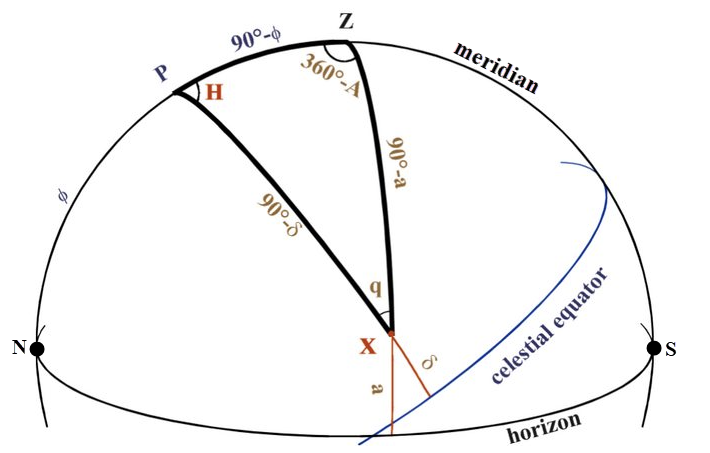
A little background. I understand the derivation of the above formula. It's a variation of $$\cos H=\frac{\sin a-\sin\delta\sin\phi}{\cos\delta\cos\phi},$$ where $a$ is altitude, and $\nu=-a$. The derivation was explained nicely by @HDE 226868 in the answer to this question. According to the diagram in that answer, no solution for $H$ implies some sort of failure of the spherical triangle PZX. But I can't visualise what such a failure actually means. Can anyone help? Thanks

Answer 1

The problem is not with the spherical triangle. A triangle can always be formed between three points on a sphere as long as the three points are not on a great circle. (The three points are the celestial pole P, the zenith Z, and the object X in the figure.)

The problem is the triangle cannot be drawn with an altitude of 0 for X if:

• the object X never rises.
• the object X never sets.

Since the spherical triangle cannot be created in the two cases, the value of the hour angle H cannot be found.

Or another way to say it, the time 2*H is the time from rising to setting. When the object never rises, there is no value of H that satisfies the rising and setting formula. If the object never sets, there is no value of H that satisfies the rising and setting formula. In both cases, the right side of the equation is greater than 1 or less than -1, but the value of cos H is limited to ranges between -1 and 1.