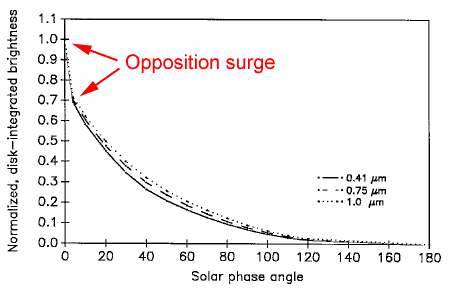
Brightness of the lunar disk varies as a function of phase angle. This phenomenon is very well established, as is the increase in brightness at low phase angles.
Is there a simple analytical formula that describes this function? It looks like brightness could be modeled as an exponential as a function of phase angle, modulo the opposition surge.
Thanks.
EDIT: I realize that these effects depend on the wavelength of the reflected light. I'm looking for an average curve, though.
The magnitude of the Moon without the opposition surge is given by a simple equation:
$m = -12.73 + 1.49 \cdot |\psi| + 0.043 \cdot \psi^4,$
where $\psi$ is the phase angle in radians (Allen 1976). This can be converted to flux using $m \propto -2.5 \cdot ^{10}\!\log F$, where $^{10}\!\log$ denotes the 10-base logarithm (as opposed to e.g. the natural log).
For the increase in flux due to the variable distance and opposition surge, I multiply F with the factor
$\left(\frac{\Delta_0}{\Delta}\right)^2 \cdot \max\left(1, 1.35 - 2.865 \cdot |\psi| \right),$
where $\Delta_0$ and $\Delta$ are the mean and current distance to the Moon, respectively. However, I can't find a reference for the last term at the moment, and I can't remember where I obtained it. I'll post that information if I find it.
CM to the lunar magnitude, it doesn't discuss the meaning.
$\endgroup$