7
$\begingroup$

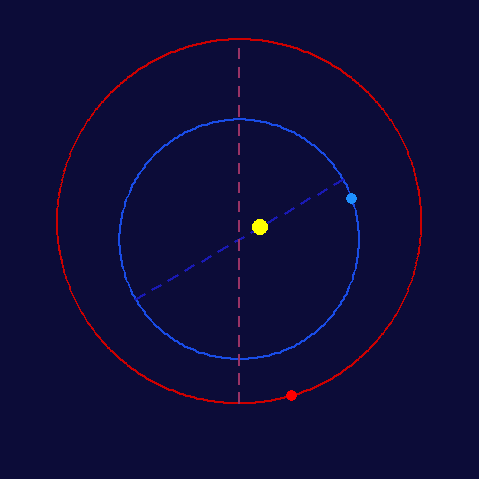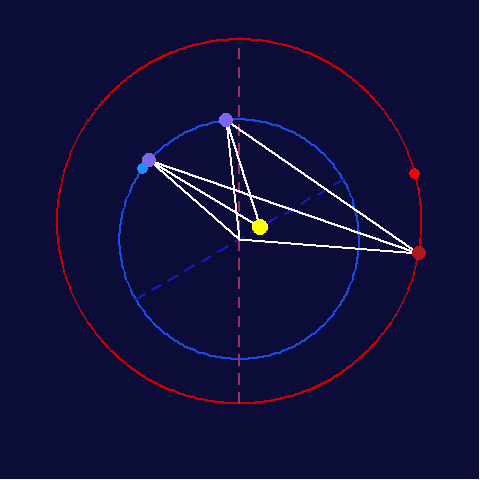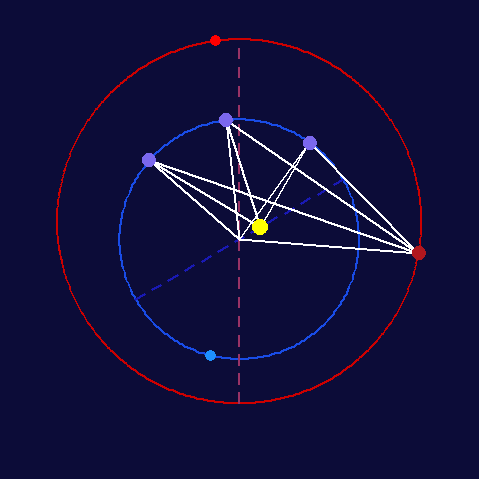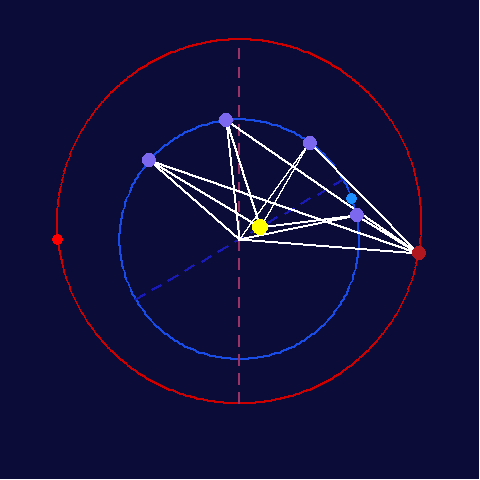
How did astronomers in the 18th and 19th centuries used to calculate a comet's or planet's orbit using observational data, given that this data is relative to a non static reference point (i.e. the Earth)?

For instance, here one can find the measurements of Mars' declination by Tycho Brahe throughout two decades. Not only it seems very difficult to me to deduce the right curve from the original observations points, but also the peaks of the curve (which resembles a sinusoid) do not seem perfectly equally distant from each other.

Improve this question
$\endgroup$
6
  • 2
    $\begingroup$ Epicycles for a geocentric view point and best fit ellipses if you accept both planets orbit the sun elliptically. If you're really into this sort of stuff, contact me directly, I've done some work on best fitting ellipses. $\endgroup$
    – user21
    Commented Sep 11, 2016 at 16:27
  • 3
    $\begingroup$ At risk of stating the obvious, it was enormously difficult. Article on that here. plus.maths.org/content/origins-proof-ii-keplers-proofs It mentions 987 pages of remaining mathematical calculations by Kepler. The Scientific American article that your website reference, is, unfortunately a pay article, so didn't read that one. By the 18th and 19th century, with telescopes and teams of people dedicated to this, they probably had the procedure pretty much down pat. Scientists were the rockstars of the 18th and 19th century. They had many people to crunch numbers for them. $\endgroup$
    – userLTK
    Commented Sep 11, 2016 at 17:49
  • $\begingroup$ @barrycarter thanks for the reply, I will send you a message by e-mail now $\endgroup$
    – gilbertohasnofb
    Commented Sep 11, 2016 at 19:35
  • $\begingroup$ @userLTK I can imagine these calculations were extremely difficult (and probably extremely tedious), but I would be very curious about the method behind them. I will take a look in this article you pointed, and I already reserved that Scientific American issue at my library as well. $\endgroup$
    – gilbertohasnofb
    Commented Sep 11, 2016 at 19:35
  • 1
    $\begingroup$ There's a reason it took Kepler nearly 20 years to work out his three laws of planetary motion. I'm sure the math was long and tedious. Note that they did not have calculators or computers (of course) so any and all math was done on paper. And what's more, math beyond what you learned in highschool didn't exist. Things like logarithms and cosines were looked up in a lookup table (people back then published entire books on calculating the log of all values they could - In fact, most of Copernicus' book was actually just lookup tables). $\endgroup$
    – zephyr
    Commented Sep 12, 2016 at 14:56

1 Answer 1

Reset to default
11
$\begingroup$

After researching a bit more, I think I can answer my own question (or at least share what I have found). When Kepler derived his laws using Tycho Brahe's observational data of Mars, he followed the procedure below:

  • first, the orbital period of Mars must be known. This value was already known in the time of Kepler (aprox. 687 days) $^1$, and can be derived as follows: first, one takes notes of the synodic period of Mars (which "[...] is the time that elapses between two successive conjunctions with the Sun–Earth line in the same linear order."$^2$). This can be done by calculating the elapsed time between two consecutive observations in which Mars is in the same apparent position in the sky. Then to calculate the orbital period of Mars, one can use:$\dfrac{1}{T_{M}} = \dfrac{1}{T_{E}} - \dfrac{1}{T_{M\mathrm{syn}}}$,$^3$ where $T_{M}$ is the orbital period of Mars, $T_{E}$ is the orbital period of Earth and $T_{M\mathrm{syn}}$ is the synodical period or Mars.

  • knowing the orbital period, Kepler then selected some of Brahe's observations that are exactly 687 days apart from each other (that is, one Martian year apart). This means that regardless of Mars relative position in the sky, the planet will be in the same position in relation to the Sun:

enter image description here

  • using triangulation, Kepler then calculated the distance of Mars to the Sun in terms of the distance of Earth to the Sun (1 AU). He did this for several positions of Mars.$^1$

  • while any three points define a circle, four or more do not necessarily do so. Kepler noticed that the collected data of distances between Mars and the Sun could be fit into an ellipse with the Sun in one of the focuses.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .