What are some applicable problems with the correct usage of G?

Question

In some fields of astrophysics, where distances are measured in parsecs (pc), velocities in kilometers per second (km/s) and masses in solar units (), it is useful to express G as approximately 4.302 x 10^-3 pc M^-1 (km/s)^2 ... Is G not approximately 6.67 x 10^-11 N(m/kg)^2? Any reasonable explanation would be appreciated.

Answer 1

Usually, using different units is done for convienience, as HDE 226868's answer explains. If we're talking about stars, then measuring masses relative to the Sun is sensible. The Sun is the closest star to us, and astronomers understand it much better than any other, making it the de facto standard of comparison. Similarly, on the interstellar scales the parsec is more convenient than the meter. The conversion is trivial and doesn't change anything physically. Recall $F = ma$ to see how the newton corresponds to base SI units: $$\mathrm{N}\cdot\left(\frac{\mathrm{m}}{\mathrm{kg}}\right)^2 = \left(\frac{\mathrm{kg}\cdot\mathrm{m}}{\mathrm{s}^2}\right)\frac{\mathrm{m}^2}{\mathrm{kg}^2} = \frac{\mathrm{m}^3}{\mathrm{kg}\cdot\mathrm{s}^2}\text{,}$$ so we can just multiply the SI value of $G$ by $$\left(\frac{1.989\times 10^{30}\,\mathrm{kg}}{1\,\mathrm{M}_\odot}\right) \left(\frac{1\,\mathrm{pc}}{3.086\times 10^{16}\,\mathrm{m}}\right) \left(\frac{1\,\mathrm{km}}{1000\,\mathrm{m}}\right)^2\text{.}$$ But there could be another reason to use solar masses in particular. The value of Newton's gravitational constant is: $$\begin{eqnarray*} G &=& 6.674\times 10^{-11}\,\frac{\mathrm{m}^3}{\mathrm{kg}\cdot\mathrm{s}^2}\\ &=& 2.959122083\times 10^{-4}\,\frac{\mathrm{AU}^3}{\mathrm{M}_\odot\cdot\mathrm{day}^2}\\ % &=& 3.964015993\times 10^{-14}\,\frac{\mathrm{AU}^3}{\mathrm{M}_\odot\cdot\mathrm{s}^2}\\ &=& 4.300917271\times 10^{-3}\,\frac{\mathrm{pc}}{\mathrm{M}_\odot}\frac{\mathrm{km}^2}{\mathrm{s}^2}\text{.} \end{eqnarray*}$$ The difference in precision is real. The 2010 CODATA value of $G$ is $ (6.67384\pm0.00080)\times 10^{-11}$ in the SI units, which has a relative uncertainty of $10^{-4}$, so we can't expect more than about $4$ significant figures. On the other hand, the standard gravitational parameter $\mu = GM$ of the Sun is known to a much greater precision than either $G$ or their masses individually, so expressing $G$ in solar masses gives a more precise value. The basic reason for that is simple: it's much easier to compare the orbits of planets to each other than, say, to compare the Sun to the International Prototype of the Kilogram kept in a French vault.

Of course, masses of other stars and interstellar distances tend to have uncertainties larger than that, so this is irrelevant on that scale. Still, within the solar system, using units of solar masses (or just $GM_\odot$ directly) allows one to make much more precise measurements, and similarly Earth masses for satellite orbits around the Earth.

Measuring $G$ in terms of parsecs (or light-years) and $(\mathrm{km}/\mathrm{s})^2$ would be more convenient any time one is dealing with galactic-scale distances, such as in the galactic rotation curve: 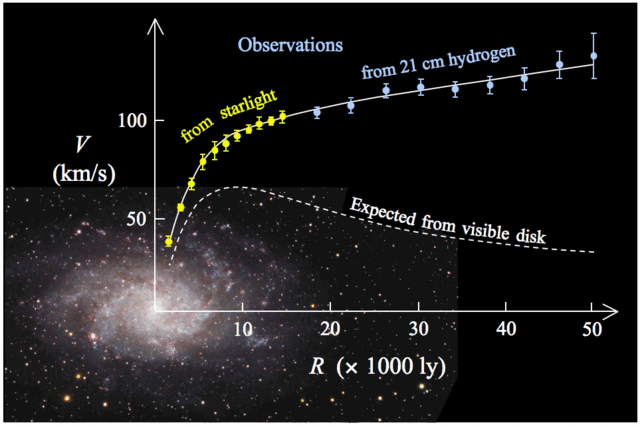(Image from Wikipedia).

Answer 2

I think one of the reasons for using different units is how you take the measurements for the other variables in the equation.

For example, let's say you have two marbles, and you want to calculate the gravitational force between them (not a lot!). You place them a certain distance apart, and measure it with your meter stick. You find the distance between them to be $0.146 \text{ meters}$. Now you weigh them, and find their masses to be $0.00156 \text{ kilograms}$ apiece. You decide to use the value of $G$ with units including meters and kilograms.

Now let's say you have two stars far, far away. You take measurements based on parallax and find that they're roughly $35,400 \text{ light-years}$ away from each other. Your instruments can't measure this to any greater accuracy. You measure their masses by calculating how much they deflect light, and come up with a figure of $10 \text { solar masses}$ apiece. You therefore decide to use some form of $G$ that, for some reason, has solar masses and light-years as some of its units.

Basically, you choose different forms of a constant depending on the units you're able to measure with your instruments. You won't be able to figure out exactly how many meters your stars are apart from each other, but you can figure out just about how many light-years apart they are, with less than a light-year of uncertainty.