How does this strategy for chasing the sunset work?

Question

In Randall Munroe's What If? blog he tackles this question:

What is the longest possible sunset you can experience while driving, assuming we are obeying the speed limit and driving on paved roads?

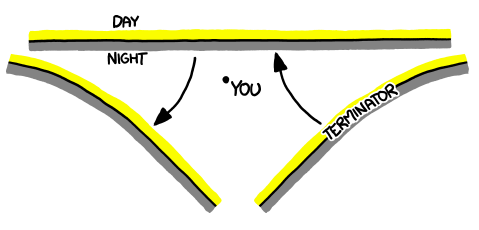
His final answer is the following, which allows you to experience about a 95 minute sunset. But the diagram isn't the most self-evident. What does the diagram mean, and how does the strategy work?

If you're in northern Norway on a day when the Sun just barely sets and then rises again, the terminator (day-night line) moves across the land in this pattern:

To get a long sunset, the strategy is simple: Wait for the date when the terminator will just barely reach your position. Sit in your car until the terminator reaches you, drive north to stay a little ahead of it for as long as you can (depending on the local road layout), then u-turn and drive back south fast enough that you can get past it to the safety of darkness.

Answer 1

I would go to the Arctic circle, where you can experience an almost perpetual sunset, without having to drive around.

Edited to answer the question: My interpretation of the diagram, is:

1. The terminator at a certain time near sunset, is the one shown towards the east (darkness approaches), running NE/SW.
2. As time progresses, it moves into the top horizontal location, running E/W; this consistent with the more northerly latitudes having continual daytime, coinciding with a certain parallel polar circle.
3. As morning approaches, the terminator moves towards the west (bringing daylight with it), and runs NW/SE.

I think the arrows would be better if the tip of the one on the right, joined the base of the one on the left, showing your car journey as a continuous one.