A supplemental answer to probably someone's answer:
Why this can indeed be called interferometry:
Once one thinks in terms of physical optics (e.g. $\text{exp}(j(\omega t - \mathbf{k} \cdot \mathbf{r} ))$ ) instead of ray optics, imaging is always an interference problem, and the math behind correlating signals from an array of radio telescopes to produce an image is not so much different than the math behind calculating an intensity pattern at the focal plane of an optical telescope.
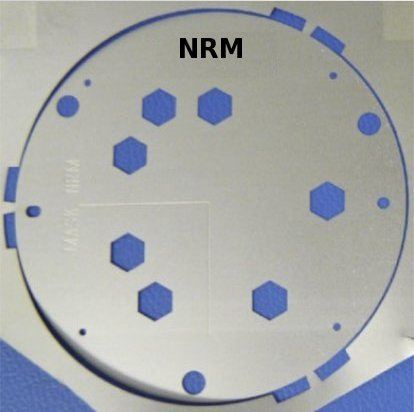
From the Aperture Mask Interferometry section on page 5 of the JWST Pocket Guide (see below) you can see that they have no problem using terms like "interferometry" and "non-redundant baselines" as one would when laying out a sparse pattern of dish antennas in an array. See for example a Google Maps image of the Meerkat Array Core below, which has already produced images with only 16 sites occupied with active dishes.
While the mask shown in the question is a pupil mask, the pupil plane is conjugate to the telescope's aperture. So it's very similar to having seven large hexagonal holes in front of the JWST, or just using seven of the telescopes primary mirror's hexagonal mirror elements (with additional masking, these hexagons to not cover the full size of the individual elements, as shown in the illustration).
An important distinction though is that these seven small apertures are of the same order in size as their separation, while in a large radio telescope array, the spacing between receivers is usually somewhere between much larger and much much larger than the aperture of individual elements. So the analogy breaks down at this point.
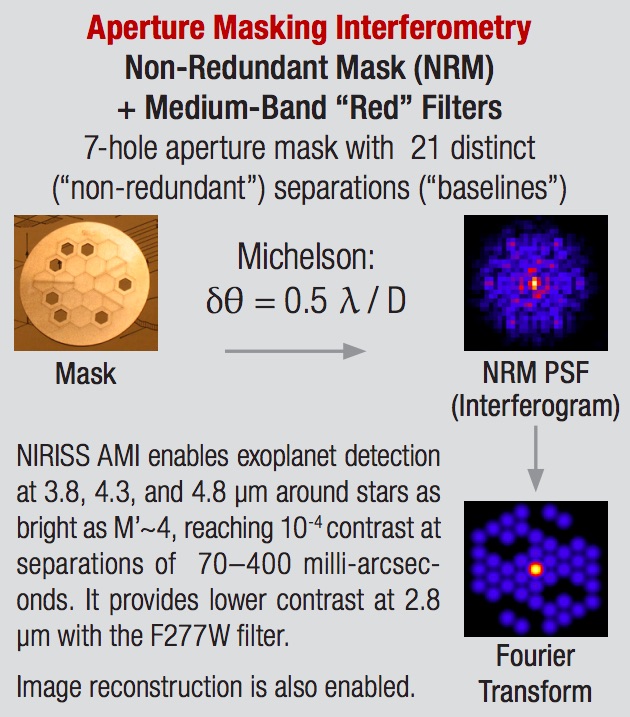
What Aperture Masking Interferometry is for:
The purpose of using the mask is to enhance the system's resolution by narrowing the central peak of the point spread function compared to what you would get from the full aperture.
By loosing contrast in the full field due to diffraction artifacts, as well as loosing ~90% of the transmitted intensity, you can enhance contrast near the center of the field by narrowing the central diffractive peak, in this case in the range of 70 to 400 milli-arc seconds, according to the JAM Team's (JWST Aperture Masking Team) slides linked below.
From the first link:
Light admitted by 7 apertures in an otherwise opaque pupil mask interfere to produce an interferogram on the detector. This interfogram has a sharper core than is provided by normal "direct" imaging. The advantage is significant: while the ability to separate closely spaced objects with normal imaging is given by the familiar Rayleigh criterion (separation δθ=1.22λ/Dδθ=1.22λ/D , where λ is the wavelength of light and D is the diameter of the telescope), interferometry can resolve objects as close as δθ=0.5λ/Dδθ=0.5λ/D (the Michelson criterion). AMI allows planetary or stellar companions that are up to ~9 magnitudes fainter than their host star and separated by 70–400 mas to be detected and characterized. AMI can also be used to reconstruct high-resolution maps of extended sources, such as active galactic nuclei.
You can read more about it in:
Below: MeerKAT array core from Google Maps at (30.7136109S, 21.4399576E).