What keeps Chthonian planets so dense?

Question

The cores of gas giants are kept under incredible pressure by the weight of the gasses of the giant. In a chthonian planet, said gases are stripped away, revealing the core of the gas giant, which should alleviate the pressure compressing the core of the gas giant. In a chthonian planet candidate, Kepler 52-c, its density is over 600 times that of Jupiter. What is keeping the planet at such a high density?

Answer 1

10 Jupiter masses at around 2 Earth radii?
That for sure doesn't exist / would be quite the sensation to discover.

When you look at data of any kind, one should pay attention to the measurment errors at least as much as to the actual value.
A regular physics result (for example for a measurment of the gravitational acceleration $g$ of where you stand) looks like $$g=(9.81 \pm 0.02) \frac{m}{s^2} $$ or if for any reason you have asymmetric errors
$$g=(9.81^{+0.02}_{-0.01}) \frac{m}{s^2} $$ and errors always give an idea about how uncertain the method is with which the value was derived. Now if you do take a look at the errors reported for the Mass quoted on the website you see that they are $$M_{planet} = (10.41^{+0.0}_{-10.41}) $$ or so to say highly asymmetric, which should make one suspicious.
A look then into the original publication makes it clear that this cited mass is in fact only an absolute upper limit.
The authors of the paper were using two methods to estimate the masses of planets.

1. Looking for transit timing variations of known, and seen transiting systems. That means they had the system Kepler 52, with transiting planets K52b,c . K52b because it transits way more often than c has a well-determined period (Period with small errors!) and because of that any deviation in expected future transit time could be attributed to the \textbf{maximum masses} of K52c.
2. The more massive and the more compact a system is, the quicker it will destabilize. This fact is often used in reverse, to take the system age and at given distances derive maximum masses below which the system must lie, or else it would have already flown apart.

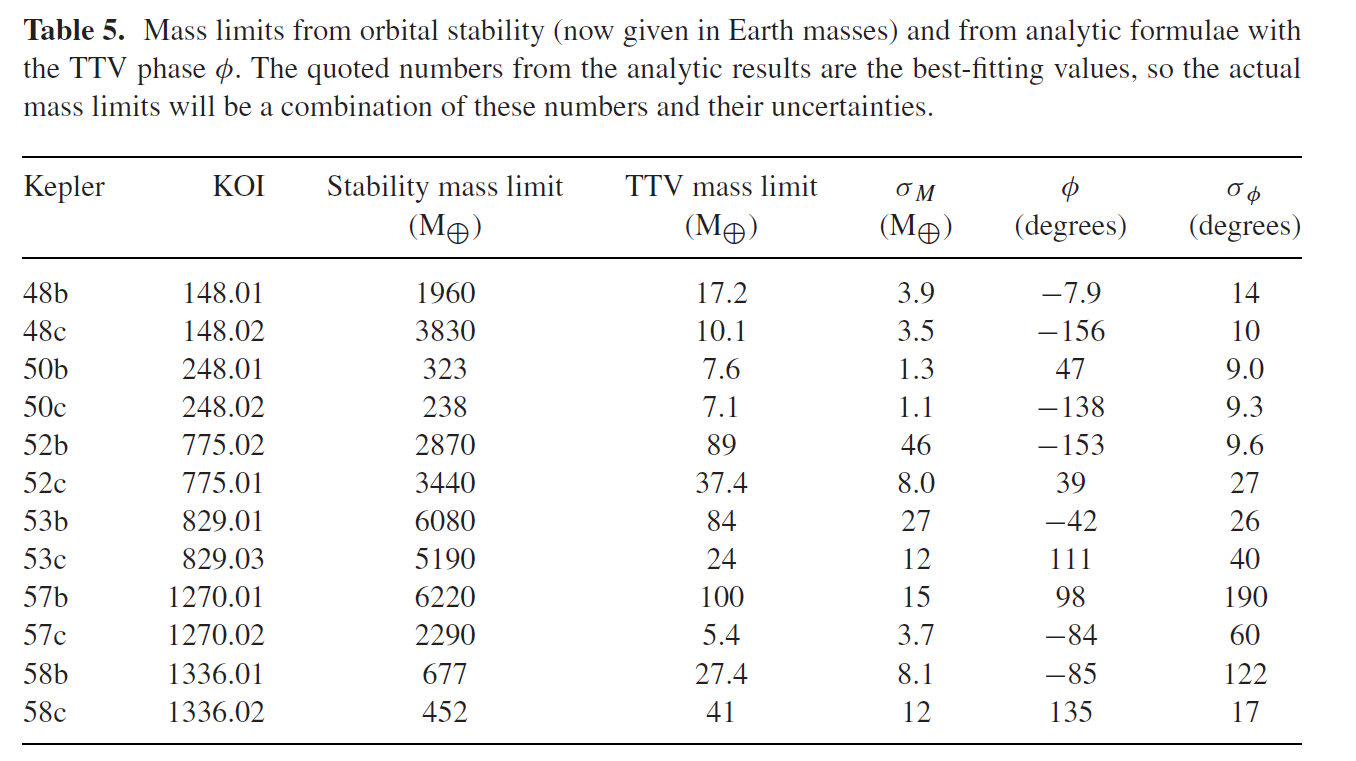
Both methods can only give maximum masses and I'll just leave here fig. 5 from the original paper with the planet you're interested in:

Now remembering, that $1 M_J \approx 320 M_{\oplus}$, you see where your 10 Jupiter masses for K52c come from: That's the planets possible maximum mass for system stability. The TTV method gives already a constraint that is 100 times lower ($37.4 M_{\oplus} \approx 0.11 M_J$).
Thus $37.4 M_{\oplus}$ is the planets true maximum mass.

This is clearly an error on the side of exoplanet.eu, but then probably there are too many planets and papers to read for whoever puts those data in there.

Summarizing
What we have here is only a maximum mass. Also the wrong one. To say what is now more probable, if $M_{K52c} = 37.4 M_{\oplus}$ or $M_{K52c} = 3.74 M_{\oplus}$ i'm not certain enough if I understand their anticorrelation method for the TTV signals.